I’m going to jump down here to continue discussing Lawvere’s topos-theoretic approach to mechanics… the tree of comments is getting oppressively complex!
Urs wrote:
But actually, what I understand best [of Lawvere and Kock’s work on physics and geometry] is that where the topos-theoretic underpinning is not directly visible.
Yeah, I feel the same way. Luckily, we don’t really need to know much topos theory to deal with this stuff. We can just imagine that we’re working in a category of smooth spaces that lets us do everything we might ever want to do — including work with infinitesimal spaces.
As you know, the most important infinitesimal space is what Jim Dolan calls ‘walking tangent vector’: an infinitesimal arrow. Lawvere calls it , since maps from to any smooth space are tangent vectors in :
Chen’s category of smooth spaces, as defined in our paper, is not big enough to include such infinitesimal spaces. We defined this category as follows:
A smooth space is a set equipped with, for
each convex set , a collection of functions
called plots in , such that:
-
If is a plot in ,
and is a smooth map between convex sets,
then is a plot in ,
-
If is an open cover of a convex
set by convex subsets ,
and has the property that
is a plot in for all , then is a plot in .
-
Every map from a point to is a plot in .
A smooth map from the smooth space to the smooth
space is a map such that for every
plot in , is a plot in .
In highbrow lingo, this says that smooth spaces are sheaves
on the category whose objects are convex sets and whose morphisms are
smooth maps, equipped with the Grothendieck topology where a cover
is an open cover in the usual sense. However, smooth spaces are
not arbitrary sheaves of this sort, but precisely those
for which two plots with domain agree whenever they agree
when pulled back along every smooth map from a point to .
When we restrict to sheaves with the special property above, we exclude infinitesimal spaces and we don’t get a topos — we get something called a ‘concrete quasitopos’.
If we allow all sheaves, we get a topos. Unfortunately, I believe this does not include infinitesimal spaces.
(I wanted to exclude infinitesimal spaces in our paper since I wanted our smooth spaces to be sets with extra structure. In other words, I wanted a forgetful functor from smooth spaces to sets which was faithful — mainly so differential geometers and topologists wouldn’t freak out. That’s the point of condition 3 above, and that’s also what the word ‘concrete’ means above.)
When I started writing this post I hoped Chen’s smooth spaces would include the ‘walking arrow’ if we modified the definition to obtain a topos. Now I don’t believe this. But, since I spent a lot of time writing this post, I’ll describe the modification anyway!
The idea is that we stop saying that a smooth space is a set equipped with a set of plots for each convex set . Instead, we simply say that a smooth space is a magical gizmo which assigns to each convex set a set of ‘-shaped plots in ’. We also need a way to ‘pull back’ -shaped plots to -shaped plots whenever we have a map .
More precisely, we say:
An abstract smooth space is a functor from convex sets to sets.
So, for any convex set we have a set of -shaped plots in , and if is a smooth map between convex sets, we get a
pullback map
such that
and
Moreover, we demand that satisfy
the sheaf condition: if is an open cover of a convex
set by convex subsets , and
are plots in that agree when pulled back to the intersections , there is a unique -shaped plot in that restricts to all the :
This is a topos of sheaves on a category with a certain Grothendieck topology. But, I’m pretty sure it doesn’t include the ‘walking arrow’ , since I can’t imagine what the nontrivial plots in would be.
I now think we need to generalize Mostow’s approach to smooth spaces instead of Chen’s if we want to include infinitesimal spaces. Or, we could just read Kock’s book — he has a construction that works too.
Now, as for how Lawvere uses such topos to describe ‘laws of motion’, maybe I’ll talk about that in a separate post. I heard him discuss these things at his 60th birthday conference in Florence, and — somewhat to my shock — I felt for the first time that I actually understood them! But, I’ll need to dig up my notes or look at his paper again before I can say anything intelligent.

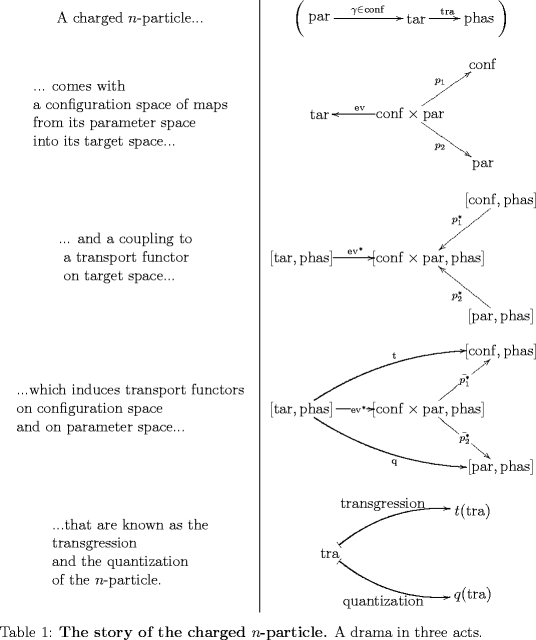
Action as a functor
John writes, in this week’s lecture notes:
Incidentally, when reading this I am in the middle of writing up a new refinement of the description of the cube.
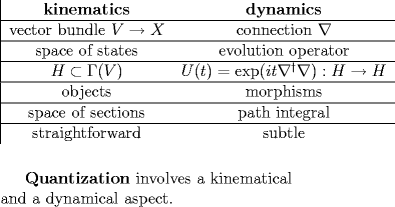
I am going to post more details in a while, but I am also trying to condense the main idea into a single table, that organizes all the physics terms and tries to show what’s really going on.
The action functor is featured in the middle column:
I will describe this in more detail in a seperate post and discuss examples.
Two caveats:
(1) The above can be applied blindly only to the kinematical part of the quantization. Dynamics should follow the same pattern, but is more subtle.
But, on the other hand, we have a kind of holography at work, which says that the kinematics of the -particle looks like the dynamics of the -particle. I am gradually better understanding the details of the formalism behind that, but not sufficiently yet.
I do wonder, though, if, in the end, we want to turn that around and make it a definition: instead of directly saying what the quantum dynamics of the -particle is, we define it as the quantum kinematics of the -particle.
I’ll need to think about this and work through more examples.
(2) Where the above table says “transgression” I am slightly abusing common terminology.
Ordinary transgression is the composition of a pullback along as above, but then followed by “integrating out parameter space”, by pushing forward along the projection As you can see, I don’t use this push-forward in the prodecure indicated in the above table.
Instead, I retain the information of parameter space and get out an extended QFT, namely a functor on in one step (instead of successively integrating out various parts of parameter space).
So, maybe I shouldn’t say “transgression” in the above. But it actually does, in the end, amount to the same sort of construction. (See this discussion for more on how “my transgression” relates to ordinary transgression)