QFT of Charged n-Particle: Algebra of Observables
Posted by Urs Schreiber
What is the “algebra of observables”, really?
Another puzzle piece in my quest for understanding the arrow-theory of quantum mechanics, and in particular of the quantum mechanics of -dimensional objects coupled to -bundles with connection.
After meditating a little about how the functorial conception of quantum field theory according to Segal relates to the “AQFT” approach of Doplicher, Haag, Kastler, Roberts like the “Schrödinger picture” relates to the “Heisenberg picture”, it is time to come to terms with the true nature of the algebra of observables of the charged -particle.
The -particle of shape with configuration space has a space of states given by the generalized elements of the -bundle with connection it couples to.
(examples: the point particle, the string)
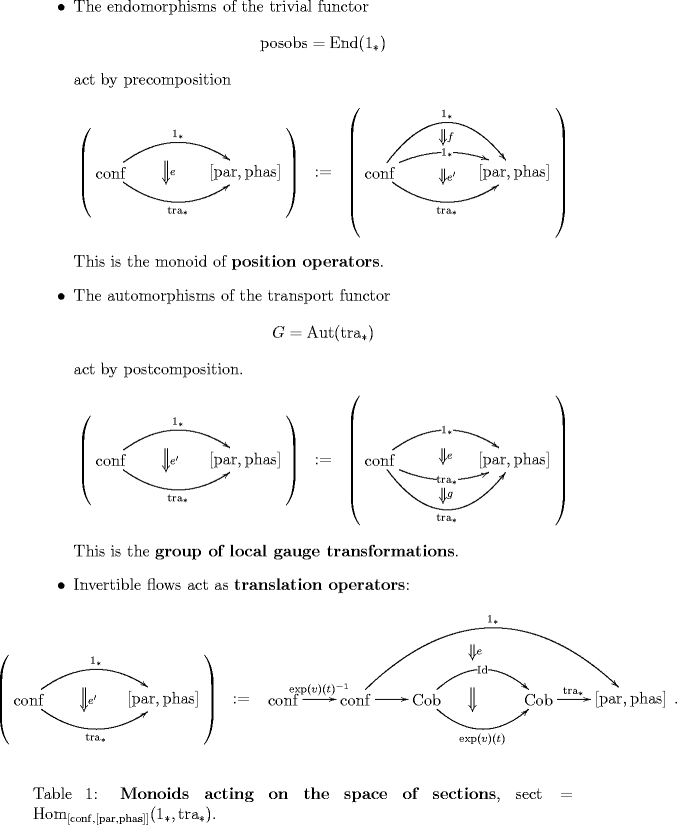
As described in the “Rosetta Stone”-section (1.2), there are three monoids naturally acting on this space of states:
1) Endomorphisms of the trivial bundle may be precomposed with a state This corresponds to acting with a position operator or multiplication operator (multiplication by a function on configuration space) on the state.
2) Automorphisms of the gauge bundle may be postcomposed with a state This corresponds to acting with a local gauge transformation on a state.
3) Flows on configuration space may be composed with a state.

The standard example is the electromagnetically charged 1-particle on target space coupled to the line bundle .
In this case, the space of states, , is that of sections of the given line bundle. The above position operators are given by mutiplying with functions . The translation operators are given by 1-parameter groups of diffeomorphisms on sections. Their differential yields the action of the covariant derivative on sections, known as the (covariant) momentum operator.
For trivial line bundle this gives the familiar Weyl algebra, the algebra of observables of the free 1-particle on .
What is so very special about this? Why does nature care?
Stare at the three different actions on the space of states above for a while… precomposition of 2-morphisms, postcomposition, and whiskering.
Question: What kind of transformation is that?
Answer: A (pseudo)natural transformation!
The above three operations are unified in the following tin can equation:

This tin can encodes: a diffeomorphism (the left vertical morphism), the covariant translation along it (the 2-cell adjacent to it), a gauge transformation (the 2-cell on top of that), and multiplication by a function on configuration space (the 2-cell on the right) – all different components of one natural operation on sections.
These are the components of a map of the space of sections (where I regard as an -category with two objects) which is connected to the identity
We are apparently lead to
Definition: The monoid of observables of the charged -particle is the submonoid of endomorphisms of the space of sections that is connected to the identity.
This neatly generalizes the way that flows along vector fields are endomorphisms of configuration space connected to the identity.
It seems that we are entitled to coin a slogan:
The algebra of observables acts on the space of states like, and generalizing how, the monoid of arrow fields acts on configuration space.
By the natural nature of everything in sight, this action automatically contains translation operators (momentum) as well as multiplication operators (position) and local gauge transformations.
If you look closely, you see that this is more flexible concerning the presence of invertibles in configuration space, thereby alleviating the issue Bruce pointed out. This may be relevant for directed configuration spaces like those discussed here.
The above figures are taken from The Charged Quantum -Particle: Kinematics and Dynamics

Re: QFT of Charged n-Particle: Algebra of Observables
By private email I was asked concerning the use of “position observable” versus “position operator”, for instance with respect to the above terminology .
The standard convention, at least in the AQFT literature
(By the way, a good source is Hans Halvorson, Michael Mueger: math-ph/0602036 , the appendix of which I talked about here)
is to take the “algebra of observables” (i.e. the algebra their axioms assigns to a given subset of parameter space) to be the algebra generated by the Weyl algebra.
Given a classical phase space, we get the corresponding Weyl Algebra (see for instance p. 34 of the above) by using the symplectic form as an abelian group cocycle.
For instance, for the single 1-particle on target space the real line this gives the algebra containing the exponential “position operators” and the exponential “momentum operators” with product
This we may try to represent on a Hilbert space, and Stone-vonNeumann tells us that there is only one non-pathologic irrep, namely the standard one on square integrable sections where acts by multiplication and by differentiation .
The “algebra of observables” is then usually taken to be the algebra generated by this.
Either generated as a -algebra, or as a vonNeumann-algebra, which would be the double commutant of the above, i.e. the collection of all operators on which commute with all operators which commute with the Weyl algebra operators.
Since the canonical rep is an irrep, this means that we’d consider all bounded operators on to be the “observables”.
Among them are the operators that arise as arbitrary universally measurable functions of the operator (compare Alain Connes’s recent remark on that, over at the NCG blog). These I would call the “position observables”.
In my “arrow theoretic” treatment above, I did not even get as far as considering such worldly issues as Hilbert space structures and boundedness. In that respect my use of the term “position observable” would perhaps make some people feel itchy, and they’d rather see me say “position operator” or whatever.
But of course my point was another one. I was trying to understand why it is that we consider our “observables” to be those generated from some Weyl algebra of a classical phase space, postpoining any discussion of technical details of implementation.
So, I am at the point: I know, abstractly, what an “charged -particle” is. Why and what should I consider as a “phase space” in the first place. Why and what would be a notion of “algbra of observables” associated with that.