Higher Gauge Theory and the String Group
Posted by John Baez
Tomorrow — unless stopped by global warming demonstrations at Heathrow — I’ll go to meet Urs Schreiber in Vienna. I’ve been wanting to talk to him for quite a while now. He produces math faster than I can keep up, and I hope it’ll be easier to catch up in person. Blogs are great, but conversation is still better for many things.
We’ll be attending a Workshop on Poisson Geometry and Sigma Models organized by Anton Alekseev, Henrique Bursztyn and Thomas Strobl at the Erwin Schrödinger Institute. I’m giving this talk:
- John Baez, Higher gauge theory and the string group.
Abstract: Higher gauge theory is a generalization of gauge theory that describes the parallel transport not just of particles, but also strings or higher-dimensional branes. To handle strings, we categorify familiar notions from gauge theory and consider ‘principal 2-bundles’ with a given ‘structure 2-group’. These are a slight generalization of nonabelian gerbes. We focus on examples related to the 2-groups associated to any compact simply-connected simple Lie group . We describe how these 2-groups are built using the level- central extension of the loop group of , and how they are related to the ‘string group’. Finally, we discuss 2-bundles with as structure 2-group, and pose the problem of computing characteristic classes for such 2-bundles in terms of connections.
Those of you who’ve already understood my Abel Symposium talk on Higher gauge theory and elliptic cohomology will find little really new here except a description of characteristic classes for -bundles, and the problem of computing them in terms of connections — a problem which Urs may know the answer to already.
Indeed, everything about this new talk is nearly identical to that previous one up to page 14, where I spend more time explaining how the “nerve” construction turns a topological 2-group like into a topological group called . A lot of topologists already know this construction; I’m not sure how many mathematical physicists do! For similar reasons I say more about the string group on page 16, and more about string structures on page 20. I decided to leave out all allusions to elliptic cohomology.
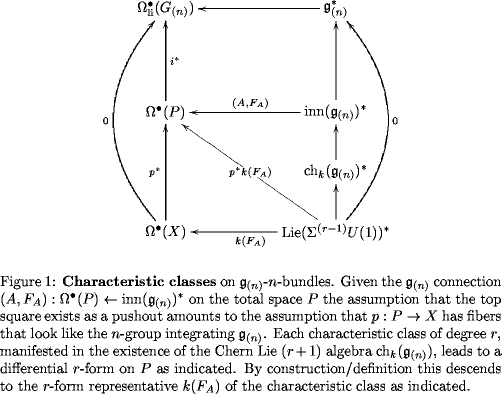
So, overall this talk is gentler and I hope clearer than the last. One feature that might interest experts is that I describe the real cohomology of the classifying space for -2-bundles! Filling in some gaps in the work of Branislaw Jurco, Danny Stevenson and I showed this classifying space is just In other words, it’s the same as the classifying space for -bundles. So, to understand the characteristic classes for -2-bundles, you need to understand the cohomology of .
I learned at the Abel symposium that if you work over the real numbers, this cohomology ring is just what you’d hope: it’s the cohomology of modulo the ideal generated by the ‘second Chern class’. In short: where is some nonzero element (it doesn’t matter here which) in .
Now, given a -2-bundle over a nice space , it’s classified by some map so any cohomology class gets pulled back to a class These elements are the ‘characteristic classes’ of our 2-bundle . The problem, then, is to describe all these characteristic classes in terms of deRham cohomology, starting from an arbitrary connection on .
(Note: here we can’t require that the connection satisfy the ‘fake flatness’ condition we demand of a full-fledged 2-connection with well-defined holonomies! Such 2-connections only exist on certain -2-bundles.)
I have a vague memory that Urs and Jim Stasheff have already done most of what it takes to solve this problem — I just can’t find the relevant web page. There’s a well-known isomorphism between and the algebra of invariant polynomials on the Lie algebra of . This should somehow give a description of in terms of the Lie 2-algebra of , or perhaps the closely related ‘Chern–Simons Lie 3-algebra’. Given this and the isomorphism we should get a description of the real characteristic classes of -2-bundles in terms of connections.
So, when I pose the ‘Nice Problem’ at the end of my talk, I expect Urs will pretend to think very hard for 20 seconds… and then solve it!

Re: Higher Gauge Theory and Elliptic Cohomology
Shouldn’t the real characteristic classes of a 2-bundle come from invariant expressions involving its 3-form curvature?
Is that what you have in mind?
It seems to me you either have something else in mind or I am misunderstanding you.
For a while now, ever since I understood # how ordinary Lie algebra cocycles, invariant polynomials and transgression elements give rise to higher Lie algebras (the key is to understand all of thse issues in terms simply iof the coholomogy of the qDGCA corresponding to !), each, I was thinking about repeating this process in higher categorical dimension.
There should be a notion of Lie -algebra cocycle, of Lie -algebra invariant polynomial and of Lie -algebra transgression elements, for any given Lie -algebra.
And it’s actually pretty clear what these should be:
- a Lie -algebra cocycle is simply a closed element in the (quasi-free) differential graded algebra which is Koszul dual to that Lie -algebra.
- an “invariant polynomial” on a Lie -algebra is a closed element in the qDGCA corresponding to the inner-derivation Lie -algebra, with a certain restriction on the degree.
And a transgression element is a potential of the latter which restricts to the former.
(I can describe this more intelligibly, if desired.)
Anyway, I was thinking that once one has defined invariant polynomials on Lie -algebras this way, there would be an obvious way to generalize Chern classes etc, and in fact get a notion of characteristic classes of -bundles.
This was one of the things on my “to do” list, an item with woefully small chances of being assigned a sufficiently high nice value any time soon.
But that’s not the “Nice problem” which you are referring to, it seems. (?)