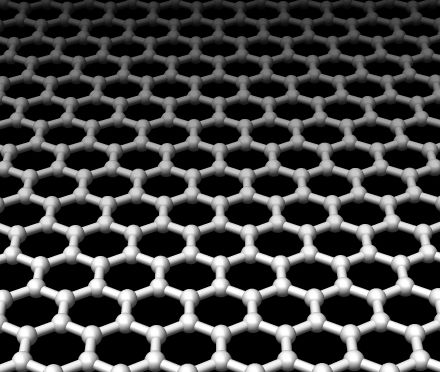
In week277 of This Week’s Finds, find out what’s a million times thinner than paper, stronger than diamond, a better conductor than copper, and absorbs exactly
π α
of the light you shine through it.
Hint: if you were really tiny, it would look sort of like this:
TrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/2034
Cool. I’m just beginning to learn about graphene. There’s a lot one can do with it, and people keep inventing new tricks — apparently it’s a really hot topic.
Neat. This reminds me of my old physics days. We were early researchers of looking at their molecular dynamics via ultra-fast (femtosecond) laser pulses. It was fun.
“… Edward Conrad from the Georgia Institute of Technology and colleagues have now grown graphene layers from a silicon carbide substrate in such a way that each layer is rotated by 30 degrees with respect to the lower layers. This MEG differs from naturally occurring graphite where each layer is rotated by 60 degrees with respect to the lower layers….”
In the 4th paragraph you say “Then at the end I’ll give you a little update about the star Betelgeuse.” Will this be coming in a later update to the article, or should that sentence be deleted?
Also, a typo: “whenever you’re got”
Oh, and the heading at the top of the article claims to be “Week 278” rather than 277.
Thanks for all the corrections, Stuart. Fixed!
I wrote the column before my trip back home from Paris, but posted it after I got home. As you can see, I was pretty jet-lagged. I was going to include the news about Betelgeuse, but then I got too tired! I’ll include it next Week, assuming Betelgeuse doesn’t go supernova by then.
Check out these images of graphene.
Nice! Actual photos of the atoms!
I was particularly caught by the points vibrating on an interval:
Staring at the animation but not long enough to be sure, it seems to me that two particles get very close to each other (narrowly avoided mid air collision)
but NOT 3 or more simultaneously getting very close
correct?
why?
which dynamical system is this the animation of? I can’t answer your question just by looking at the picture… not that I would be necessarily be able otherwise..
Oops. Sorry. I see the formula for the Hamiltonian in the wikipedia article the picture is linked from. Never mind.
Now you can answer my question? no bunching of 3 or more particles near collision?
what in the Hamiltonian says `can’t happen’??
I don’t see anything in the classical Hamiltonian that prevents triple collisions, let alone bunching. For a moment I thought this was some version of the Toda lattice. Then I checked Leznov and Saveliev but couldn’t find the system there.
Watching it, my intuition is that triple collisions would be vanishingly rare. For a precise conjecture: The phase space (which may be thought of as the space of initial conditions) should be a manifold (or at least an orbifold) and so have a notion of set of measure zero; given any finite period of time, the set of initial conditions that will produce a triple collision has measure zero. Since the graphic above covers only a finite period of time (and then jumps back to the beginning), we expect (in the sense of probability theory) never to see a triple collision.
Let me start by saying that I am rusty as far as dynamical systems are concerned and that I am only commenting about the classical Hamiltonian system.
Toby, you are certainly right that if a set of configurations has measure 0 and if it is unstable, you will never see any motion near it.
Now, I am assuming that the Hamiltonian
we are looking at describes N particles on a line. There is an obvious translational symmetry: shift all particles at once by the same amount and the Hamiltonian doesn’t change. So we can reduce by the action of . This amounts to introducing new variables , , I think. The reduced system has an equilibrium at and it’s stable. Hence the original, non-reduced system has a relative equilibrium and it’s relatively stable.
Am I missing anything?
Now, I am assuming that the Hamiltonian
That would be a rather stupid system (as you have alluded).
Presumably, the springs have a nonzero equilibrium length. So the Hamiltonian is
what I have in mind, as many of you probably have guessed, is compactifying the configuration space dynamics
You are right of course. And the Wikipedia article has a silly typo. I should have realized that, but clearly I am not thinking like a physicist!
Anyway, if you reduce the correct Hamiltonian, it becomes a system of N-1 particles with the potential of the form which is a bunch of uncoupled harmonic oscillators all vibrating around the stable equilibrium .
Apparently in the simulation the total linear momentum is 0, so the relative equilibrium is an equilibrium (as opposed to the center of mass drifting in one direction with constant speed).
For those unwilling to read the Wikipedia article, this physical system is just like a row of identical rocks, each attached to its neighbors by ideal springs.
In the continuum limit this system reduces to the wave equation, and a good quantum field theory course will make you quantize this system and then take the continuum limit, to help you understand what a quantum field is like.
But in the theory of phonons we don’t take the continuum limit!
I don’t care much for your “But these quantized steps are there nonetheless, no matter what is doing the wiggling - it appears to be a completely general principle.” and the sentences that follow.
Firstly, we can construct arbitrary superpositions of bosonic eigenstates of the number operator, continuously varying observables of a quantum field, so it’s not clear that there’s any more discrete structure than there is in anything that allows mode decompositions (here I mean, for example, a string of infinite length, not a string with constraints on the motion of its ends). Secondly, the particle content of a model depends on the relative acceleration of an observer (although the literature on the Unruh effect is perhaps indecisive). All in all, it’s almost conventional to doubt that there is a particle interpretation of a bosonic quantum field.
The particle content seems to be limited to the discrete superselection sectors of fermionic fields. Since we can’t construct superpositions of superselection sectors, we can’t continuously vary fermion number observables of the field (I discount mixtures). Even for fermions, we can construct superpositions of (m fermions,n anti-fermions) states with (m+k fermions,n-k anti-fermions) states, so about the only thing we can be sure is discrete is an aggregate fermion number.
That kind of discreteness matches up moderately well with the discrete structures of topology, so I take it there is a similar but different categorical structure to superselection and topological sectors. Although people who work with graphene can be expected to talk as if there is a topological discrete structure, I suppose you might like to be sure of the categorical structure.
That’s all sucking eggs, but you don’t seem to have written much about superselection in past “weeks”, and, as I say, I don’t care for your discussion in Week 277. Be nice to see you look at it.
By the end of your article it seems clear that the excitations of most interest are fermionic, but it’s not clear to me how much interest there is in bosonic excitations of the graphene structure?
Peter wrote:
I don’t care much for your “But these quantized steps are there nonetheless, no matter what is doing the wiggling - it appears to be a completely general principle.” and the sentences that follow.
Firstly, we can construct arbitrary superpositions of bosonic eigenstates of the number operator, continuously varying observables of a quantum field, so it’s not clear that there’s any more discrete structure than there is in anything that allows mode decompositions…
I was thinking that This Week’s Finds has become too technical, so I decided to write an easier one.
I was having fun trying to quickly explain the quantization of a harmonic oscillator, how this gets applied to free quantum fields, and also the idea of “dispersion relations”, all in the context of condensed matter physics, where the particles involved are typically what people call “quasiparticles” rather than “elementary particles”. And, I was trying to do it while denying myself every form of mathematical vocabulary that’s more complicated than addition, subtraction, multiplication and division.
This is an almost impossible task — quantum mechanics is famously hard to explain without using math. So, I’m not surprised you’re dissatisfied with the result.
But anyway, when I wrote:
But these quantized steps are there nonetheless, no matter what is doing the wiggling - it appears to be a completely general principle.
what I meant is that the number operator has a discrete spectrum.
All in all, it’s almost conventional to doubt that there is a particle interpretation of a bosonic quantum field.
Sure, but people in quantum optics will continue to talk about photons, and people in condensed matter physics will continue to talk about phonons. They’re just too handy, despite their limitations. There are problems where they work well, and problems where they don’t.
Typo: when you reduced the Energy-momentum-mass formula to the rest-mass energy formula, you forgot to remove a square.
The massless case of this formula (which you mentioned) is also well-approximated by the large-momentum limit for massive objects. This is handy for estimating the Chandrasekhar bound on the mass of an irrotational white dwarf.
Scott wrote:
Typo: when you reduced the Energy-momentum-mass formula to the rest-mass energy formula, you forgot to remove a square.
Fixed! You’d think I could at least get right. 
The massless case of this formula (which you mentioned) is also well-approximated by the large-momentum limit for massive objects. This is handy for estimating the Chandrasekhar bound on the mass of an irrotational white dwarf.
I guess it’s held up by the ‘degeneracy pressure’ of a highly relativistic gas of electrons?
Yes, everything acts approximately massless when it’s zipping along fast enough…
>>I guess it’s held up by the “degeneracy pressure” of a highly relativistic gas of electrons?
Yeah. As you add more electrons to the gas, the transition from non-relativistic to relativistic behavior alters the dimensional analysis when solving for an energy minimum, and the equilibrium radius drops to zero. I vaguely remember doing a homework exercise on this from the Griffiths dead-cat book.
The phase transition in multi-electron systems between nonrelativistic and relativistic also applies to the Bohr atom, and to more sophisticated corrections (relativistic Dirac equation) of that model. The break occurs at or a couple of electrons beyond atomic number 137:
This could be fun:
Re: This Week’s Finds in Mathematical Physics (Week 277)
It turns out that bilayer graphene is better suited for computers because it creates a significant gap at ambient temperature, that one can tune, thus making it possible to make a transistor.