Division Algebras and Supersymmetry II

March 13, 2010

Division Algebras and Supersymmetry II

Posted by John Baez

$MathML-enabled post (click for more details).$

John Huerta and I are finishing up another paper:

John Baez and John Huerta, Division algebras and supersymmetry II.

We’d love comments and corrections! In particular, the material on supergravity theories needs improvement. But the story is already quite cool, since it connects the octonions and higher gauge theory to superstrings, super-2-branes and supergravity.

$MathML-enabled post (click for more details).$

In case you’re wondering, I don’t “believe in” string theory. But I’ve always been curious about it, since it’s full of interesting mathematics. After I officially quit work on quantum gravity — a subject rife with warring factions — I eventually realized I could relax and do whatever I wanted, without needing to take sides. So, I’ve been slowly trying to learn more about superstrings, supergravity and supersymmetric membranes, as kind of back burner hobby. But it seems the best way to learn a bit about a subject is to do some work on it. So, with John Huerta’s help, I’ve been dipping my toe into this pool. I make guesses; he does the hard work required to prove or disprove them.

I’d always been curious about why supergravity is so quirky when it comes to working in some dimensions and not others. It’s frustrating to hear people wax rhapsodic about, say, 11-dimensional supergravity, when you don’t know what’s so special about 11 dimensions! There are some simple explanations one often hears, but they’re not completely satisfying.

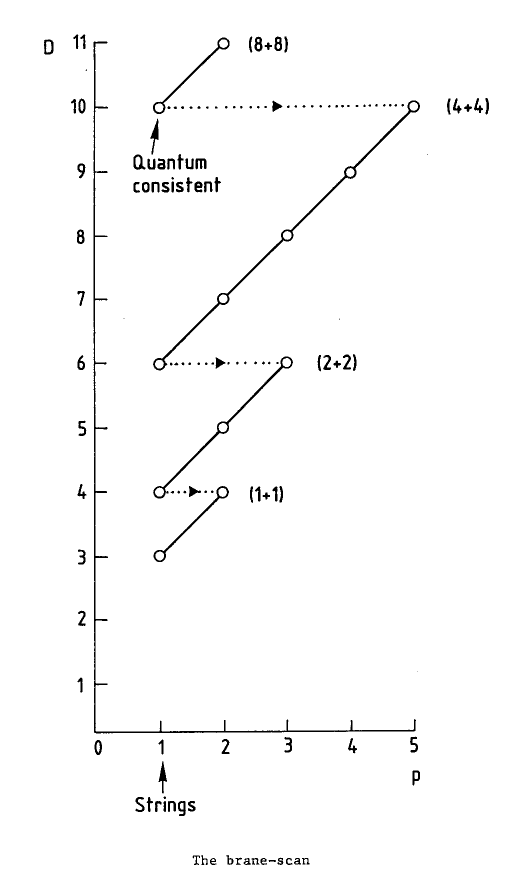
Now I feel happier. I know I’m still just skimming the surface — but it’s nice to see some beautiful mathematical structures that explain why there are classical superstrings in dimensions 3, 4, 6, and 10, and super-2-branes in dimension 4, 5, 7 and 11, as shown in this old chart by Michael Duff, which shows super- $p$ -brane theories in $D$ -dimensional spacetime:

Duff says that the four diagonal lines here come from the four normed division algebras. In our previous paper on this subject, we explained how these algebras, namely:

the real numbers, of dimension 1,
the complex numbers, of dimension 2,
the quaternions, of dimension 4, and
the octonions, of dimension 8

explain subtle features of the interplay between spinors and vectors in spacetimes of dimensions 3, 4, 6, and 10, respectively. It’s no coincidence that these dimensions are two higher than those of the division algebras! We explained why. And we explained how the mathematics of division algebras leads to an identity involving 3 spinors — an identity that holds only in these dimensions:

$(\psi \cdot \psi) \psi = 0$

We explained how this ‘3- $\psi$ ’s rule’ underlies the existence of supersymmetric Yang–Mills theories when spacetime has dimension 3, 4, 6, or 10. It also underlies the existence of classical superstring theories in these dimensions.

None of this was new: we just wanted to explain it clearly, all in one place. Now we’re doing something a bit more novel. We can also use division algebras to prove a fancier rule involving 4 spinors. This ‘4- $\Psi$ ’s rule’:

$\Psi \cdot ((\Psi \cdot \Psi) \Psi) = 0$

holds in spacetimes of dimensions three higher than those of the division algebras: that is, dimensions 4, 5, 7, and 11. And this rule is why there exist supersymmetric 2-branes in these dimensions!

Even better, in the octonionic case — that is, 11-dimensional spacetime — these 2-branes are closely related to 11-dimensional supergravity. In fact, a lot of people expect that 2-branes and supergravity in 11 dimensions are just offshoots of a magnificent, magical, mysterious, murky mess called ‘ $M$ -theory’.

But our focus in this paper lies elsewhere. In fact, the 3- $\psi$ ’s rule and the 4- $\Psi$ ’s rule are ‘cocycle conditions’.

Let me sketch why this is interesting.

The groups that physicists like all have Lie algebras. Lie algebras show up in particle physics because they describe how particles transform as they move around. But recently people have discovered gadgets called Lie $2$ -algebras, which do the same job for strings. And Lie $3$ -algebras, which do the same job for 2-dimensional membranes, usually called ‘ $2$ -branes’. And so on!

In fact, a Lie $n$ -algebra is actually a kind of hybrid structure: a blend of a Lie algebra and an $n$ -category.

But if you’re a practical sort of person, you may want to build a Lie $n$ -algebra starting from some stuff you can easily get your hands on. The simplest way is to start with a Lie algebra and a gizmo called an $(n+1)$ -cocycle: some sort of function satisfying some equation called a ‘cocycle condition’. From this, you can get a Lie $n$ -algebra that includes your original Lie algebra.

All stuff has a supersymmetric version, too — a version where we treat bosons and fermions in a unified way!

In particular, the 3- $\psi$ ’s rule actually asserts the existence of a 3-cocycle, which lets us build a Lie 2-superalgebra which is useful for superstring theories. And the 4- $\Psi$ ’s rule asserts the existence of a 4-cocycle, which lets us build a Lie 3-superalgebra which is useful for super-2-brane theories.

What Lie superalgebra do we start with in this game? It’s a very important one, called the ‘Poincaré superalgebra’.

You see, special relativity says we live in Minkowski spacetime. The group of symmetries of Minkowski spacetime is called the Poincaré group. This has a Lie algebra: the Poincaré algebra. And there’s a supersymmetric analogue of all this, starting from ‘super-Minkowski spacetime’. Super-Minkowski spacetime unifies vectors and spinors in a nice way. And the supergroup of symmetries of super-Minkowski spacetime has a Lie superalgebra, called the Poincaré superalgebra.

Super!

The 3- $\psi$ ’s rule implies that the Poincaré superalgebra has a nontrivial 3-cocycle when spacetime has dimension 3, 4, 6, or 10.

Similarly, the 4- $\Psi$ ’s rule implies that the Poincaré superalgebra has a nontrivial 4-cocycle when spacetime has dimension 4, 5, 7, or 11.

So, the 3- $\psi$ ’s rule gives Lie 2-superalgebras extending the Poincaré superalgebra in dimensions 3, 4, 6 and 10. We call these superstring Lie 2-algebras, because they appear to be useful in understanding how classical superstrings move around in these dimensions.

Similarly, the 4- $\Psi$ ’s rule gives Lie 3-superalgebras extending the Poincaré superalgebra in dimensions 4, 5, 7 and 11. We call these 2-brane Lie 3-algebras, because they appear to be useful in understanding how classical super-2-branes move around in these dimensions.

The biggest and best of these gadgets is the Lie 3-superalgebra built using the octonions — the one that governs super-2-branes in 11 dimensions. This was already studied by Sati, Schreiber and Stasheff. They called it sugra(10,1), thanks to the role it plays in 11-dimensional supergravity. Indeed, you can see Urs blogging about these ideas back in 2007, and even earlier, on my birthday back in 2006.

So, what we’re doing now is fitting this gadget into a bigger pattern — a pattern that involves the division algebras. Since I like $n$ -categories and I like division algebras, it makes me very happy to see them fitting together this way. In mathematics, everything sufficiently beautiful is related.

Posted at March 13, 2010 2:06 AM UTC

TrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/2189

35 Comments & 1 Trackback

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Why don’t arbitrary composition algebras work? What is it about normed division algebras that’s necessary for doing these constructions?

Posted by: Mike Stay on March 14, 2010 10:38 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Good question! A composition algebra is one which satisfies

(1)

|a b|^2=|a|^2|b|^2

without requiring $|-|^2$ to be positive definite. It’s a classic theorem that the only real composition algebras have dimension 1, 2, 4, and 8, just like normed division algebras. In fact, the only real composition algebras are either the normed division algebras for which the norm is positive definite, or the split composition algebras, for which the norm has signature $(n/2, n/2)$ , where $n$ is the dimension of our composition algebra.

I think the answer to your question is: they do work, but not for physical applications. In dimensions $n+2$ , vectors are built from $2 \times 2$ Hermitian matrices over $\mathbb{K}$ . This is nice, because determinant gives the norm:

(2)

\left| \begin{matrix} t + x & y \\ y^* & t - x \end{matrix} \right| = t^2 - x^2 - |y|^2

If $\mathbb{K}$ is normed of dimension $n$ , you’ll see the above formula gives signature $(1, n+1)$ . If $\mathbb{K}$ is split, it gives a split signature!

Most of the formulas (all of them, I bet — but I’d need to check) would still work in the split case, so you’d still get vectors, spinors and intertwiners, all for spacetimes with split signature. These might be worth thinking about, but they’re not the spacetimes one usually considers for superstrings or more general membranes.

[John Baez: I’m still stuck inside the computer here, so I can’t reply like a normal person. Here’s an explanatory remark for the lurking layfolk: ‘spacetimes with split signature’ is jargon for spacetimes that have an equal number of space and time dimensions! These have mathematically beautiful properties — but few physicists work on them, for an obvious reason: nobody wants to be called a ‘low-down dirty two-timing rat’.]

Posted by: John Huerta on March 15, 2010 12:09 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Since it’s now become possible again for me to post comments, I’ll celebrate by trying to transfer some comments from the n-Forum to this thread.

Posted by: John Baez on March 16, 2010 5:31 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Thanks, John and John for these results. This is very pleasing.

The 3- $\psi$ ’s rule implies that the Poincaré superalgebra has a nontrivial 3-cocycle when spacetime has dimension 3, 4, 6, or 10.

Similarly, the 4- $\Psi$ ’s rule implies that the Poincaré superalgebra has a nontrivial 4-cocycle when spacetime has dimension 4, 5, 7, or 11.

Very nice! That’s what one would have hoped for.

Can you maybe see aspects of what makes these cocycles special compared to other cocycles that the Poincaré super Lie algebra has? What other cocycles that involve the spinors are there? Maybe there are a bunch of generic cocycles and then some special ones that depend on the dimension?

Is there any indication from the math to which extent $(3,4,6,10)$ and $(4,5,7,11)$ are the first two steps in a longer sequence of sequences? I might expect another sequence $(7,8,10,14)$ and $(11, 12, 14, 18)$ corresponding to the fivebrane and the ninebrane. In other words, what happens when you look at $n \times n$ -matrices with values in a division algebra for values of $n$ larger than 2 and 4?

Here a general comment related to the short exact sequences of higher Lie algebras that you mention:

properly speaking what matters is that these sequences are $(\infty,1)$ -categorical exact, namely are fibration sequences/fiber sequences in an $(\infty,1)$ -category of $L_\infty$ -algebras.

The cocycle itself is a morphism of $L_\infty$ -algebras

$\mu : \mathfrak{siso}(n+1,1) \to b^2 \mathbb{R}$

and the extension it classifies is the homotopy fiber of this

$\mathfrak{superstring}(n+1,1) \to \mathfrak{siso}(n+1,1) \to b^2 \mathbb{R} \,.$

Forming in turn the homotopy fiber of that extension yields the loop space object of $b^2 \mathbb{R}$ and thereby the fibration sequence

$b \mathbb{R} \to \mathfrak{superstring}(n+1,1) \to \mathfrak{siso}(n+1,1) \to b^2 \mathbb{R} \,.$

The fact that using the evident representatives of the equivalence classes of these objects the first three terms here also form an exact sequence of chain complexes is conceptually really a coincidence of little intrinsic meaning.

One way to demonstrate that we really have an $\infty$ -exact sequence here is to declare that the $(\infty,1)$ -category of $L_\infty$ -algebras is that presented by the standard model structure on dg-algebras on $dgAlg^{op}$ . In there we can show that $b \mathbb{R} \to \mathfrak{superstring} \to \mathfrak{siso}$ is homotopy exact by observing that this is almost a fibrant diagram, in that the second morphism is a fibration, the first object is fibrant and the other two objects are almost fibrant: their Chevalley–Eilenberg algebras are almost Sullivan algebras in that they are quasi-free. The only failure of fibrancy is that they don’t obey the filtration property. But one can pass to a weakly equivalent fibrant replacement for $\mathfrak{siso}$ and do the analog for $\mathfrak{superstring}$ without really changing the nature of the problem, given how simple $b \mathbb{R}$ is. Then we see that the sequence is indeed also homotopy-exact.

This kind of discussion may not be relevant for the purposes of your article, but it does become relevant when one starts doing for instance higher gauge theory with these objects.

Here some further trivial comments on the article:

Might it be a good idea to mention the name “Fierz” somewhere?
page 3, below the first displayed math: The superstring Lie 2-superalgebra is [an] extension of
p. 4: the bracket of spinors defines [a] Lie superalgebra structure
p. 6, almost last line: this [is] equivalent to the fact
p. 13 this spinor identity also play[s] an important role in
p. 14: recall this [is] the component of the vector

Posted by: Urs Schreiber on March 16, 2010 6:00 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Thanks for the discussion of exact sequences, Urs. I have at times worried a lot about why one would ever be interested in “strict” exact sequences of the sort we’re using here. It’s nice to get a clear general picture of this, and I may add your comments — citing you, of course — to our paper. I guess whenever we construct a Lie $n$ -algebra by extending a Lie algebra using a cocycle, we actually get a “strict” exact sequence of the sort mentioned in our paper. But yes, I see that this is a “coincidence”.

And yes, we should talk about Fierz identities. We just forgot! And thanks for catching all those typos.

Can you maybe see aspects of what makes these cocycles special compared to other cocycles that the Poincaré super Lie algebra has? What other cocycles that involve the spinors are there? Maybe there are a bunch of generic cocycles and then some special ones that depend on the dimension?

Well, of course these cocycles are special because they come from Fierz identities that hold only in certain special dimensions, and we’ve tried to “explain” that by giving a proof using normed division algebras.

But alas, we don’t know reallly good answers to any of the questions you are asking here. Of course we’ve considered these questions. I think they are utterly fascinating. At times I’ve wanted John Huerta to do his thesis on these questions! But right now, other questions seem a bit easier, and perhaps interesting to more people. The questions you’re asking require a highly developed expertise in representation theory.

Is there any indication from the math to which extent $(3,4,6,10)$ and $(4,5,7,11)$ are the first two steps in a longer sequence of sequences? I might expect another sequence $(7,8,10,14)$ and $(11, 12, 14, 18)$ corresponding to the fivebrane and the ninebrane.

If John Huerta gets really good at representation theory we could work out the full story, but right now we are mainly happy to see that the techniques we’re using do not give a 3-brane sequence $(5,6,8,12)$ . I.e., they do not give a ‘5- $\Psi$ ’s rule’ in all these dimensions. And that’s what one would expect, given the apparent lack of a supersymmetric 3-brane theory in 12d Minkowski spacetime.

Posted by: John Baez on March 16, 2010 6:16 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Urs wrote:

Is there any indication from the math to which extent $(3,4,6,10)$ and $(4,5,7,11)$ are the first two steps in a longer sequence of sequences? I might expect another sequence $(7,8,10,14)$ and $(11, 12, 14, 18)$ corresponding to the fivebrane and the ninebrane. In other words, what happens when you look at $n \times n$ -matrices with values in a division algebra for values of $n$ larger than $2$ and $4$ ?

Aren’t the first two sequences supposed to be from the columns in Duff’s chart in the post? Is the specialness of $5$ and $9$ connected to their being $(1+4)$ and $(1+8)$ ?

Posted by: David Corfield on March 16, 2010 6:08 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

David wrote:

Aren’t the first two sequences [strings in dimensions $3, 4, 6, 10$ and 2-branes in dimension $4, 5, 7, 11$ ] supposed to be from the columns in Duff’s chart in the post?

Yes, and you’ll see that he explicitly links this chart to the normed division algebras, but in a somewhat mysterious way, which we wanted to clarify.

Is the specialness of $5$ and $9$ connected to their being $(1+4)$ and $(1+8)$ ?

I’m not sure what you think is special about $5$ and $9$ .

Posted by: John Baez on March 16, 2010 6:22 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

I was just wondering about Urs’ fivebrane and ninebrane as in the quoted portion. He then replied about the sequence (string, fivebrane, ninebrane) of dimension $4 k + 1$ .

Posted by: David Corfield on March 16, 2010 6:25 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Okay, David I get what you mean now. I think the cocycles governing Urs’ fivebrane and ninebrane are “purely bosonic”, unlike the ones John Huerta and I are considering. I now realize that’s why I’m having trouble making any connection between what Urs is talking about now and what we did. But they should be part of the same story.

In other words: I’m guessing all $p$ -brane theories involve cocycles on the Poincaré Lie superalgebra. This superalgebra is a Z/2-graded vector space with a bracket. The even part, or “bosonic part”, is an ordinary Lie algebra, namely the Lie algebra of the Poincaré group. The odd part, or “fermionic part”, is the space of spinors. I think Urs is implicitly getting cocycles on the Poincaré Lie superalgebra from cocycles on its bosonic part.

(Checking that this is possible requires a tiny calculation, which I am alas too busy to do right now).

What are cocycles on the Poincaré Lie algebra like? Well, it should include the cohomology of the rotation Lie algebra, and in fact that could even be all there is.

The Lie algebra of the rotation group has a bunch of interesting cocycles, related to Pontryagin classes.

If I’m not getting mixed up, the Lie algebra of the rotation group has a nontrivial 3-cocycle, a nontrivial 7-cocycle, a nontrivial 11-cocycle… and so on up to a certain cutoff — and if you work with rotations in high enough dimensions, you can make this cutoff as high as you like.

So, we get cocycles of degree $4k+3$ for $k$ below a certain cutoff. These give Lie $(4k+2)$ -algebras, which in turn can be used to describe the parallel transport of $(4k+1)$ -branes.

Oh, good — the calculation is working — it matches Urs’ claim! I was worried until the end there.

But anyway, all this stuff is “purely bosonic”. John Huerta and I were focusing on cocycles that only exist on the Poincaré Lie superalgebra.

I will need to think about this more someday.

Posted by: John Baez on March 16, 2010 6:32 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

John wrote:

In other words: I’m guessing all p-brane theories involve cocycles on the Poincaré Lie superalgebra.

I shouldn’t get so carried away. Let me just say that some $p$ -brane theories involve cocycles on the Poincaré Lie superalgebra.

Posted by: John Baez on March 16, 2010 9:03 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

John,

there are these bosonic cocycles, but I was indeed wondering about the fermionic ones.

Take the case of the string: it is governed (in the sense we are discussing here) at least by one bosonic cocycle – the canonical 3-cocycle on $\mathfrak{so}(n)$ – and one fermionic cocycle – the one you are discussing with John Huerta.

The super-fivebrane we know is similarly controlled by the 7-cocycle on $\mathfrak{so}(n)$ . But shouldn’t there also be a fermionic cocycle to go with this, as with the string?

Posted by: Urs Schreiber on March 16, 2010 4:15 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

I guess you’re right: there should be a fermionic 7-cocycle, at least in 10 dimensions where Duff’s old brane scan shows a super-5-brane.

(There are newer brane scans which show many more super- $p$ -brane theories, which I don’t understand yet. I like the old brane scan because it’s based on a simple recipe for constructing super- $p$ -brane theories from cocycles.)

I would be very happy if the dashed horizontal lines in Duff’s brane scan came from some sort of ‘Poincaré duality’ that holds in the cohomology of the Poincaré superalgebra.

(I’m using “Poincaré” in two ways here!)

Since these horizontal lines correspond to ‘duality transformations’ it is perhaps not a completely ridiculous hope.

In other words, maybe there’s a simple way to turn a 3-cocycle into a 7-cocycle when we’re working with the 10d Poincaré superalgebra. Hmm, the numbers even seem promising here: $7+3=10$ .

How much do you believe that there are fivebranes in dimensions $7,8,10$ and $14$ ?

Posted by: John Baez on March 16, 2010 4:59 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Hmm, the numbers even seem promising here: 7+3=10.

Sure, yes, that’s the equation which identifies the fundamental super-fivebrane as the “magnetic” object dual to the “electrical” fundamental string in 10-dimensions.

And that electric/magnetic dualiy is indeed effectively Poincaré-duality – or rather some kind of refinement of it to differential cohomology .

As you know, right? Recall the kind of discussion we once had here at the blog entry

Electric-Magnetic-Duality and Hodge Duality Extended to Differental Cocycles.

If we use the magnetic fivebrane instead of the string to describe physics in 10-dimensions, we arrive at what is called dual heterotic string theory . Some discussion of this is at the blog entry

Dual Formulation of String Theory and Fivebrane Structures .

You ask:

How much do you believe that there are fivebranes in dimensions 7,8,10 and 14?

I believe in fundamental fivebranes in 10-dimensions, being the magnetic duals of the fundamental string.

I also believe in fivebranes as being the next $(4k+1)$ -branes after the $(4 \cdot 0 + 1)$ -brane that is the string.

Posted by: Urs Schreiber on March 16, 2010 6:15 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

John wrote:

$7 + 3 = 10$

Urs wrote:

Sure, yes, that’s the equation which identifies the fundamental super-fivebrane as the “magnetic” object dual to the “electrical” fundamental string in 10-dimensions.

Yeah, I knew that, but it somehow sounded different when I thought of it this way: “there’s a Fierz identity involving 3 spinors that gives a 3-cocycle on the Poincaré superalgebra, and there’s probably one involving 7 spinors that gives a 7-cocycle, and $7 + 3 = 10$ so maybe these are related by some kind of Poincaré duality in Lie superalgebra cohomology”.

Much more impressive, eh?

I know a little bit about Poincaré duality for Lie algebra cohomology but not so much for Lie superalgebras — that’s the main reason this idea seemed new and interesting.

But you’re right, maybe I can see it now beginning to boil down to the same darn thing you said!

That would be nice…

Posted by: John Baez on March 16, 2010 6:35 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

How could you classify in different fundamental theories a string theory whose objects were 5 branes? In 2 dimensions, there are very few parameters to restrict the degrees of freemdom, and get the number “5 theories”. For example, one uses relatively trivial stuff like open and closed, chiral or not, the symmetry between the right and left mode movers. Basically, it boils down on how strings attach.

But in 5 dimensions, all kinds of crazy stuff can happen. One can attach them to a number of dimensions from 0 to 5, and each border with the craziest geometries. So, how one could classify those 5 branes in different species of theory?

Posted by: Daniel de França MTd2 on March 16, 2010 6:57 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

so maybe these are related by some kind of Poincaré duality in Lie superalgebra cohomology”.

Oh, you mean in Lie algebra cohomology? I see. Hm, so this is asking the following, I suppose:

take the Chevalley-Eilenberg dg-algebra of the super Poincaré Lie algebra in 9+1 dimensions and regard it is a model for a rational (super)space. Does this rational (super)space satisfy Poincaré duality with formal super-dimension $10|something$ in the sense of rational homotopy theory?

This sounds like something somebody should look into.

Posted by: Urs Schreiber on March 16, 2010 7:06 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

David wrote:

Is the specialness of $5$ and $9$ connected to their being $(1+4)$ and $(1+8)$ ?

Well, at least one has to be careful with the numerology here, as the string= $1$ -brane and membrane= $2$ -brane would not fit that pattern that you suggest.

But I think there is yet another pattern running here, where “fundamental $n$ -branes” exists for $(4k +1)$ (string, fivebrane, ninebrane) whose worldvolume theory is conformal, and then one dimension higher runs the sequence of $(4k + 2)$ -branes whose worldvolume theory is the corresponding Chern-Simons theory (membranes, etc.).

But I have only a vague understanding of the general pattern here.

Posted by: Urs Schreiber on March 16, 2010 6:13 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

I do not know if you noticed the recent article

Fei Han, Kefeng Liu, Gravitational anomaly cancellation and modular invariance, arxiv/1007.5295

…we obtain general anomaly cancellation formulas of any dimension. For $4k+2$ dimensional manifolds, our results include the gravitational anomaly cancellation formulas of Alvarez-Gaumé and Witten in dimensions 2, 6 and 10 as special cases. In dimension $4k+1$ , we derive anomaly cancellation formulas for index gerbes. In dimension $4k+3$ , we obtain certain results about eta invariants, which are interesting in spectral geometry.

Of course, it is well known that the eta invariants are related to the cancellation of anomalies, but here a rather complete picture is claimed for the dimensions discussed in this cafe post, some aspects of which are directly related to the work of Urs with Hisham Sati. Unfortunately the above article is dense with complicated formulas, hence hard to read; fortunately the classical formulas from Bismut, Lott, Freed and others are recalled.

Posted by: Zoran Skoda on August 3, 2010 8:11 PM | Permalink | Reply to this

dense with complicated formulas

As Dan Kan remarked to me after my first talk at MIT
(paraphrased): just because you have written a formula on the board doesn’t mean you have communicated with your audience.

Posted by: jim stasheff on August 4, 2010 12:10 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

The Wikipedia composition algebra page says there are 1-dimensional composition algebras when $char(K) \ne 2$ . Has anyone considered your construction for $char(K) \ne 0$ or 2?

Posted by: Mike Stay on March 16, 2010 6:41 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

What’s ‘our construction’? Lie 2-superalgebras and Lie 3-superalgebras extending the Poincaré superalgebra? No, nobody has considered this in characteristic other than 0. After all, we only revealed the construction for characteristic 0 this weekend! Math moves fast these days, but not that fast.

There’s a book that discusses composition algebras in nonzero characteristic, though: The Book of Involutions.

Posted by: John Baez on March 16, 2010 6:56 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

I am wondering what the most general abstract way would be to understand that division algebras are related to supersymmetry.

I am guessing it must be all related to the fact that the four division algebras are twistings by a 2-cocycle of the group algebras over $\mathbb{R}$ of the groups $(\mathbb{Z}_2)^0 = \{0\}$ , $(\mathbb{Z}_2)^1 = \mathbb{Z}_2$ , $(\mathbb{Z}_2)^2$ and $(\mathbb{Z}_2)^3$ , as described in the last paragraph of John Baez: The Fano plane.

So at the bottom of it, it is all governed by $\mathbb{R}$ and $\mathbb{Z}_2$ . But of course that’s also true for supersymmetry: super vector spaces are precisely the $\mathbb{Z}_2$ -graded real vector spaces equipped with the nontrivial symmetric monoidal structure.

How can might one pin down this similarity and relation more precisely?

And: suppose I replace $\mathbb{Z}_2$ here with another (abelian?) group $G$ . Then I can still talk about $G$ -graded real vector spaces and look for nontrivial symmetric braidings on them. And I can look for group 2-cocycles on the group algebras $\mathbb{R}[G]^n$ . So do I get a story completely analogous to that of supersymmetry and division algebras?

Posted by: Urs Schreiber on April 9, 2010 10:53 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Urs wrote:

I am wondering what the most general abstract way would be to understand that division algebras are related to supersymmetry.

Me too!

I am guessing it must be all related to the fact that the four division algebras are twistings by a 2-cocycle of the group algebras over $\mathbb{R}$ of the groups $(\mathbb{Z}_2)^0 = \{0\}$ , $(\mathbb{Z}_2)^1 = \mathbb{Z}_2$ , $(\mathbb{Z}_2)^2$ and $(\mathbb{Z}_2)^3$ , as described in the last paragraph of John Baez: The Fano plane.

I wish that were true. But this construction produces lots of algebras, not just the four normed division algebras. For example, I can get any Clifford algebra by taking the group algebra of $(\mathbb{Z}_2)^n$ and twisting it by a 2-cocycle.

Furthermore, the octonions are obtained by taking the group algebra of $(\mathbb{Z}_2)^3$ and twisting it by a 2-cochain that’s not a 2-cocycle. That’s why the octonions are nonassociative.

And if I allow myself to twist group algebras of the groups $(\mathbb{Z}_2)^n$ by 2-cochains that aren’t cocycles, I can get all sorts of crazy nonassociative algebras. For example: if we keep applying the Cayley-Dickson construction starting with the real numbers, we get the complex numbers, the quaternions, the octonions, the sedenions, and so on — an infinite sequence of algebras! After the quaternions, they’re all nonassociative. And they can all be obtained by twisting the group algebras of the groups $(\mathbb{Z}_2)^n$ by certain 2-cochains.

I would love it if these nonassociative algebras were all related to supersymmetry, but I don’t see any sign of that.

From the papers John Huerta and I have written, it seems quite clear that supersymmetric field theories beloved by physicists have a lot to do with composition algebras. The real numbers, complex numbers, quaternions and octonions are all composition algebras. And, the properties of composition algebras, such as the alternative law, give all the cocycles that we need to construct superstring theories in dimensions 3,4,6, and 10, and super-2-brane theories in dimensions 4,5,7, and 11.

As further evidence for the importance of composition algebras, note that besides the real numbers, complex numbers, quaternions and octonions, there are precisely three more composition algebras over $\mathbb{R}$ : the split complex numbers, the split quaternions and the split octonions. And, I believe we get classical superstring and super-2-brane Lagrangians from these as well! These theories live in spacetimes with more than one time dimension — so people don’t think about these theories very much. But, I think they exist.

So, I believe we should think about composition algebras and their special properties. Anyone who likes composition algebras and category theory should read this:

Markus Rost, On the dimension of a composition algebra.

It gives a purely diagrammatic proof that the dimension of a composition algebra must be 1, 2, 4, or 8!

Posted by: John Baez on April 16, 2010 4:22 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

It gives a purely diagrammatic proof that the dimension of a composition algebra must be 1, 2, 4, or 8!

Unfortunately it does not: there are no diagrams, string or otherwise, in that short paper. It would be nice to have some.

Posted by: Todd Trimble on April 16, 2010 3:17 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

Was it the next reference from TWF169 that was intended - Dominik Boos’s Ein tensorkategorieller Zugang zum Satz von Hurwitz? Note that the link in TWF is outdated.

Posted by: David Corfield on April 16, 2010 4:07 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

Yeah, sorry — David’s right. Both papers study the same subject using a lot of the same ideas, but Boos, the student of Rost, explains the proof using string diagrams. For people who already understand string diagrams and tensor categories, the fun starts on page 36. You don’t need to understand German: just look at the pictures. The key axiom is this:

 \       /        \   /         \     /     \        /     \        /
  \     /          \ /           \   /       \      /       \      /
   \___/            |             \ /         \    /         \____/
   /   \     +      |     =  2     /    -      |  |     -     ____
  /     \           |             / \         /    \         /    \
 /       \         / \           /   \       /      \       /      \
/         \       /   \         /     \     /        \     /        \

I’ve given a talk about this proof now and then. It’s a great proof — it’s like a magic trick, where after some string diagram calculations you pull the numbers 0,1,3 and 7 out of a hat as solutions to a certain polynomial equation. I generalized Boos’ proof by extracting exactly the bare minimum requirements on the underlying category, instead of just using real or rational vector spaces.

Bruce Westbury has gone further and written a nice paper about this stuff, entitled Hurwitz’ Theorem. It seems he hasn’t made it public yet, but I guess he will someday.

Posted by: John Baez on April 16, 2010 9:51 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

This does look like fun! Although I do feel hampered by my lack of facility with German in following this paper…

I’m slowly writing up some details in the Lab based on the nice book by Conway and Smith, On Quaternions and Octonions. I also lack facility with drawing string diagrams in the Lab, but maybe someone else can help. The proofs by Conway and Smith do have a very tensor-calculus feel to them…

Posted by: Todd Trimble on April 17, 2010 12:33 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Todd wrote:

I do feel hampered by my lack of facility with German in following this paper

That does not need to be a problem, of course, there are people who are willing to train both their English and their category knowledge by translating the interesting parts of this paper - but I assume that this becomes obsolete once Bruce has published his paper?

Is the hierachy of categories $A_1,...A_4$ defined by Dominik Boos something standard? I thought that at least $A_1$ should be broadly known and have a more pertinent name like FinGraph, but did not find any reference, maybe I don’t now the right buzzwords.

Posted by: Tim van Beek on April 17, 2010 10:14 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

I am open to suggestions on how to make this publicly available. At the moment I am in Colorado with my children hoping to be able to get home next week.

It seems that this is not something that a journal would be interested in publishing. I could put this on my web pages (when I get back) and then submit to the arXiv.

Posted by: Bruce Westbury on April 17, 2010 1:05 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

Bruce wrote:

At the moment I am in Colorado with my children hoping to be able to get home next week.

I hope Eyjafjallajökull lets you go back!

It seems that this is not something that a journal would be interested in publishing.

Really??? It’s a lot more interesting than most stuff I see in journals. I’m sure you could get it published if you wanted.

I could put this on my web pages (when I get back) and then submit to the arXiv.

Okay, great. It should definitely be on the arXiv.

Posted by: John Baez on April 17, 2010 2:30 AM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

I’ve been given the okay to make this draft available:

Bruce Westbury, Hurwitz’ theorem.

Some small corrections are forthcoming, but you can already see a very nice discussion of composition algebras and vector product algebras, and a diagrammatic proof that every vector product algebra has dimension 0, 1, 3 or 7.

The classic example of a vector product algebra is the space of 3d vectors with its usual dot product and cross product. This arises from the imaginary quaternions by setting

$a \cdot b = - Re(a b)$

$a \times b = Im(a b)$

More generally, every normed division algebra is a composition algebra. We can start with any composition algebra, take its space of imaginary elements, and get a vector product algebra using the same formulas. Conversely, any vector product algebra gives a composition algebra.

Posted by: John Baez on April 17, 2010 10:25 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

I’m interested in how this relates to Hisham Sati’s papers.

OP2 bundles in M-theory

On the geometry of the supermultiplet in M-theory

According to his theory, M-theory is a limiting case of a more fundamental 27-dimensional theory, the same way the string theories are points on the moduli space of M-theory.

M-theory is 11-dimensional and Hisham Sati’s theory has 27-dimensions. However, the 16 new dimensions are not spatial dimensions but time dimensions. Therefore, this new theory, more fundamental than M-theory, has 10 spatial dimensions and 17 time dimensions.

Posted by: Anonymous on April 16, 2010 3:01 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Thanks for pointing out those papers! For some reason I hadn’t known about them. Crazy people like me have hoped for a long time that the octonionic projective plane would be important in physics. Octonionic structures are showing up all over in string theory and $M$ -theory, so why not this?

But, it will take me a long time to say something intelligent about these papers.

Posted by: John Baez on April 17, 2010 2:16 AM | Permalink | Reply to this

Read the post Division Algebras and Supersymmetry III
Weblog: The n-Category Café
Excerpt: John Huerta explains his new paper, which constructs some Lie 2-supergroups that are important in superstring theory.
Tracked: September 24, 2011 2:44 AM

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

Did you see citations of your work in An octonionic formulation of the M-theory algebra?

Posted by: David Corfield on March 17, 2014 11:04 PM | Permalink | Reply to this

Re: Division Algebras and Supersymmetry II

$MathML-enabled post (click for more details).$

No—thanks for pointing it out!

But in Oxford I had a great conversation with Leron Borsten (a former student of Duff) and Leo Hughes (a student of Borsten) about A magic square from Yang–Mills squared, A magic pyramid of supergravities and other connections between octonions and physics.

Posted by: John Baez on March 18, 2014 8:46 AM | Permalink | Reply to this

The n-Category Café

Skip to the Main Content

March 13, 2010