State-Observable Duality (Part 1)

November 25, 2010

Posted by John Baez

$MathML-enabled post (click for more details).$

I’m writing a paper called “Division algebras and quantum theory”, which is mainly about how quantum theory can be formulated using either the real numbers $\mathbb{R}$ , the complex numbers $\mathbb{C}$ , or the quaternions $\mathbb{H}$ — and how these three versions are not really separate alternatives (as people often seem to think), but rather three parts of a unified structure.

This is supposed to resolve the old puzzle about why Nature picked $\mathbb{C}$ when it was time for quantum mechanics, while turning up her nose at $\mathbb{R}$ and $\mathbb{H}$ . The answer is that she didn’t: she greedily chose all three!

But sitting inside this paper there’s a smaller story about Jordan algebras and the Koecher–Vinberg classification of convex homogeneous self-dual cones. A lot of this story is ‘well-known’, in the peculiar sense that mathematicians use this term, meaning at least ten people think it’s old hat. But it’s still worth telling — and there’s also something slightly less well-known, the concept of state-observable duality, which is sufficiently lofty and philosophical as to deserve consideration on this blog, I hope.

So I’ll tell this story here, in three parts. The first is just a little warmup about normed division algebras. If you’re a faithful reader of This Week’s Finds, you know this stuff. The second is also a warmup, of a slightly more esoteric sort: it’s about an old paper on the foundations of quantum theory written by Jordan, von Neumann and Wigner. And the third will be about the Koecher–Vinberg classification and state-observable duality.

$MathML-enabled post (click for more details).$

The laws of algebra constrain the possibilities for theories that closely resemble the quantum mechanics we know and love. Various classification theorems delimit the possibilities. Of course, theorems have hypotheses. It is easy to get around these theorems by weakening our criteria for what counts as a theory ‘closely resembling’ quantum mechanics; if we do this, we can find a large number of alternative theories. This is especially clear in the category-theoretic framework, where many theories based on convex sets of states give rise to categories of physical systems and processes sharing some features of standard quantum mechanics Here, however, I want to focus on results that pick out real, complex and quaternionic quantum mechanics as special. But before we start, let’s make sure we’re up to speed on our normed division algebras!

After discovering that complex numbers could be viewed as simply pairs of real numbers, Hamilton sought an algebra of ‘triples’ where addition, subtraction, multiplication and division obeyed most of the same rules. Alas, what he was seeking did not exist. After much struggle, he discovered the ‘quaternions’: an algebra consisting of expressions of the form $a 1 + b i + c j + d k$ ( $a,b,c,d \in \mathbb{R}$ ), equipped with the associative product with unit $1$ uniquely characterized by these equations: $i^2 = j^2 = k^2 = i j k = -1.$ He carved these equations into a bridge as soon he discovered them:

Yes, that’s him. And he spent the rest of his life working on the quaternions, so this algebra is now called $\mathbb{H}$ in his honor.

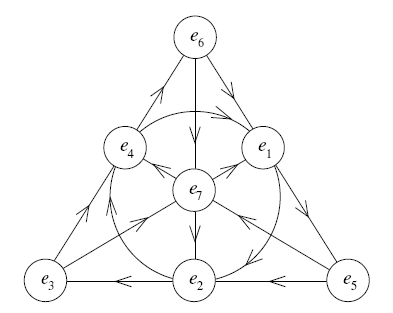
The day after Hamilton discovered the quaternions, he sent a letter describing them to his college friend John Graves. A few months later Graves invented yet another algebra, now called the ‘octonions’ and denoted $\mathbb{O}$ . The octonions are expressions of the form $a_0 1 + a_1 e_1 + a_2 e_2 + \cdots + a_7 e_7$ with $a_i \in \mathbb{R}$ . The multiplication of octonions is not associative, but it is still easily described using the Fano plane, a projective plane with 7 points and 7 lines:

This is my feeble attempt to rival Tim Silverman when it comes to pretty pictures. The ‘lines’ here are the sides of the triangle, its altitudes, and the circle containing the midpoints of the sides. Each line contains three points, and each of these triples has a cyclic ordering, as shown by the arrows. If $e_i, e_j,$ and $e_k$ are cyclically ordered in this way then $e_i e_j = e_k, \qquad e_j e_i = -e_k .$ Together with these rules:

$1$ is the multiplicative identity,
$e_1, \dots, e_7$ are square roots of -1,

the Fano plane completely describes the algebra structure of the octonions. If you haven’t done it before, make sure to use these rules to check that the octonions are nonassociative! When is it true that $(e_i e_j) e_k = e_i (e_j e_k) ,$ and when is it false? There’s a cute answer.

What is so great about the number systems discovered by Hamilton and Graves? Like the real and complex numbers, they are ‘normed division algebras’. For us, an algebra will be a real vector space $A$ equipped with a multiplication that is real-linear in each argument. We do not assume our algebras are associative. We say an algebra $A$ is unital if there exists an element $1 \in A$ with $1 a= a = a 1$ for all $a \in A$ . And we define a normed division algebra to be a unital algebra equipped with a norm $| \cdot | : A \to [0, \infty)$ obeying the rule $|a b| = |a| \, |b| .$

The real and complex numbers are obviously normed division algebras. For the quaternions we can define the norm to be: $|a + b i + c j + d k| = \sqrt{a^2 + b^2 + c^2 + d^2} .$ A similar formula works for the octonions: $|a_0 + a_1 e_1 + \cdots + a_7 e_7| = \sqrt{a_0^2 + \cdots + a_7^2}.$ With some sweat, one can check that these rules make $\mathbb{H}$ and $\mathbb{O}$ into normed division algebras.

The marvelous fact is that there are no more! In an 1898 paper, Hurwitz proved that $\mathbb{R}$ , $\mathbb{C}$ , $\mathbb{H}$ and $\mathbb{O}$ are the only finite-dimensional normed division algebras. In 1960, Urbanik and Wright removed the finite-dimensionality condition:

Theorem: Every normed division algebra is isomorphic to either $\mathbb{R}$ , $\mathbb{C}$ , $\mathbb{H}$ or $\mathbb{O}$ .

For quantum mechanics, it is important that every normed division algebra is a $\ast$ -algebra, meaning it is equipped with a real-linear map $x \mapsto x^*$ obeying these rules: $(x y)^* = y^* x^* , \qquad (x^*)^* = x.$ For $\mathbb{C}$ this map is just complex conjugation, while for $\mathbb{R}$ it is the identity map. For the quaternions it is given by: $(a 1 + b i + c j + d k)^* = a - b i - c j - d k$ and for the octonions, $(a_0 1 + a_1 e_1 + \cdots + a_7 e_7)^* = a_0 1 - a_1 e_1 - \cdots - a_7 e_7 .$ One can check in all four cases we have $x x^* = x^* x = |x|^2 1 .$

For the three associative normed division algebras, the $\ast$ -algebra structure lets us set up a theory of Hilbert spaces. Let’s quickly sketch how. Suppose $\mathbb{K}$ is an associative normed division algebra. Then we define a $\mathbb{K}$ -vector space to be a right $\mathbb{K}$ -module: that is, an abelian group $V$ equipped with a map $V \times K \to V$ that obeys the laws $(v + w)(x) = v x + w x, \qquad v(x + y) = v x + v y,$ $(v x)y = v(x y) .$ We say a map $T : V \to V'$ between $\mathbb{K}$ -vector spaces is $\mathbb{K}$ -linear if $T(v x + w y) = T(v)x + T(w)y$ for all $v,w \in V$ and $x,y \in \mathbb{K}$ . When no confusion can arise, I’ll call a $\mathbb{K}$ -linear map $T : V \to V'$ a linear operator or simply an operator.

Yeah, I know: you’re probably appalled that I’m multiplying by scalars on the right here. It makes no difference except for the quaternions, which are noncommutative. Even in that case, we could use either left or right multiplication by scalars, as long as we stick to one convention. But since we write the operator $T$ on the left in the expression $T(v)$ , it makes more sense to do scalar multiplication on the right, so no symbols trade places in the law $T(v x) = T(v)x$ . This will be especially nice when we write the operator $T$ using a matrix.

There is a category of $\mathbb{K}$ -vector spaces and operators between them. As usual, every vector space has a basis, and any two bases have the same cardinality, so we can talk about the dimension of a vector space over $\mathbb{K}$ , and every finite-dimensional vector space over $\mathbb{K}$ is isomorphic to $\mathbb{K}^n$ . Every operator $T : \mathbb{K}^n \to \mathbb{K}^m$ can be written as matrix: $(T v)_i = \sum_j T_{i j} v_j .$ See? This obeys $T(v x) = T(v)x$ , with the scalar $x$ coming out on the right!

The $\ast$ -algebra structure of the normed division algebras becomes important when we study Hilbert spaces. We define an inner product on a $\mathbb{K}$ -vector space $V$ to be a map sending a pair of vectors $u,v \in V$ to a number $\langle u,v \rangle \in \mathbb{K}$ that is $\mathbb{K}$ -linear in the second argument: $\langle u, v x + w y \rangle = \langle u, v\rangle x + \langle u,w \rangle y,$ obeys $\langle v, u \rangle = \langle u, v \rangle^*,$ and is positive definite: $\langle v, v \rangle \ge 0$ with equality only for $v = 0$ .

As usual, an inner product gives a norm: $\|v\| = \sqrt{\langle v, v\rangle} ,$ and we say a $\mathbb{K}$ -vector space with inner product is a $\mathbb{K}$ -Hilbert space if this norm makes the vector space into a complete metric space. Every finite-dimensional $\mathbb{K}$ -Hilbert space is isomorphic to $\mathbb{K}^n$ with its standard inner product $\langle v, w \rangle = \sum_{i = 1}^n v_i^* w_i .$ And, it turns out we can do quantum theory using these Hilbert spaces for either the real numbers, the complex numbers or the quaternions! Next time I’ll tell you a theorem about why these three choices are special.

This is fine for $\mathbb{R}$ , $\mathbb{C}$ and $\mathbb{H}$ : what about $\mathbb{O}$ ? It would be nice if we could think of $\mathbb{O}^n$ as an $n$ -dimensional octonionic vector space, with scalar multiplication defined in the obvious way. Unfortunately, the law $(v x)y = v(x y)$ fails, because the octonions are nonassociative. Furthermore, there are no maps $T : \mathbb{O}^n \to \mathbb{O}^m$ obeying $T(v x) = T(v)x$ except the zero map. So, nobody has managed to develop a good theory of octonionic linear algebra. (But that doesn’t mean it doesn’t exist!)

While the octonions are nonassociative, they are still ‘alternative’. You’ve heard of people leading an ‘alternative lifestyle’, and that’s very much what the octonions are like. We say an algebra is alternative if the associative law holds whenever two of the elements being multiplied are equal: $(x x)y = x(x y) \qquad (x y)x = x(y x) \qquad (y x)x = y(x x) .$ Surprisingly, this is enough to let us carry out a bit of quantum theory as if $\mathbb{O}$ , $\mathbb{O}^2$ and $\mathbb{O}^3$ were well-defined octonionic Hilbert spaces. The 3-dimensional case is by far the most exciting: it leads to a structure called the ‘exceptional Jordan algebra’. This leads to a whole world of fun. But beyond dimension 3, it seems there is little to say.

Posted at November 25, 2010 3:08 AM UTC

TrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/2307

54 Comments & 1 Trackback

Re: State-Observable Duality (Part 1)

If you relax the axioms a little further (skip alternativity but keep power associativity), you get sedenions.

Posted by: Thomas Larsson on November 25, 2010 8:40 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Yes, it’s possible Graves discovered the sedenions: in January 1844 he considered a general theory of “ $2^m$ -ions”, and tried to build a 16-dimensional normed division algebra, but “met with an unexpected hitch”.

The really cool thing about the sedenions is that every element has a multiplicative inverse. Nonetheless, they have zero divisors.

Toby Bartels discovered this after I wrote week59. It may have been known earlier, but if you read his comment at the end there, you’ll see it starts like this:

I spent a couple days thinking about why hexadecanions have no inverses, and the first thing I want to say about it is that they do.

However, these inverses are of limited applicability, because the hexadecanions are not a division algebra.

A division algebra allows you to conclude, given x y = 0, that x or y is 0. If your algebra has inverses, you might try to multiply this equation by the inverse of x or y (whichever one isn’t 0) to prove the other is 0, but this only works if the algebra is associative.

The hexadecanions are the same as what most people, following Cayley I believe, call the sedenions.

I keep hoping that some of the special features of $SO(16)$ in heterotic string theory will turn out to be related to the hexadecanions, just as many special features of 10-dimensional superstring theory are related to the octonions. So far it’s not happening. But on the other hand, so far nobody seems to be working on it.

Posted by: John Baez on November 25, 2010 11:40 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Hi John:

4 years have passed since this comment, are there any updates? Is anyone working in this area?

In Week 61, way back in 1995, you wrote:

Start with an 8-dimensional complex vector space V with a non - degenerate symmetric bilinear form on it. We can think of V as the representation of SO(8), hence Spin(8), where now I’ve switched notation and write SO(8) to mean SO(8,C), and Spin(8) to mean Spin(8,C). We can split V into two 4-dimensional subspaces V+ and V- such that ⟨v,w⟩ = 0 whenever v and w are either both in V+, or both in V-. Let Cliff be the Clifford algebra over V. Note that as a vector space, there is a natural identification of Cliff with Λ V+ ⊗ Λ V- where Λ means “exterior algebra” and ⊗ means “tensor product”. (If you are physicist you may enjoy following Dirac and thinking of Λ V+ as a Fock space for “holes”, and Λ V- as a Fock space for “particles”. If you don’t enjoy this, ignore it! We will now to proceed to fill all the holes.) Pick a non - zero vector v in Λ^4 V-, the top exterior power of V-.

Let S denote the subspace of Cliff consisting of all elements of the form uv with u in Cliff. Note that Cliff and S are representations of Cliff by left multiplication, and therefore are representations of Spin(8) - because Spin(8) sits inside Cliff. (This is a standard way to get one’s hands on the spin groups.) Note that Λ V+ and Λ V- both have dimension 2^4 = 16. We can think of both of these as subspaces of Cliff; for example, we can think of the vector u in Λ V+ as the vector 1 x u in Cliff. Note that uv = 0 when u is in Λ V+. (For physicists: since the sea of holes is filled, you can’t create another.) Thus S consists of vectors of the form uv where u lies in Λ V-, and if you think a bit, it follows that S is 16-dimensional.

Is it possible to plug a Sedenion in here as S? Or a hexadecanion?

Posted by: John Sweeney on December 4, 2014 3:26 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Sedenions are the same as hexadecanions, and while the latter term is more logical, the former is traditional so I think I’ll stick with that from now on.

I don’t know much new work on sedenions. They’re deeply unpopular.

It’s possible that we can think of the 16-dimensional space $S$ as the space of sedenions… thanks. What it is, is the 16-dimensional spinor representation of $\mathrm{Spin}(8)$ . Later in week61 I showed how to break it up into the two 8-dimensional spinor representations, the left-handed and right-handed ones. But then both of these are seen as octonions! So it makes a lot of sense to think of an element of $S$ as a pair of octonions. If we could define the sedenion product on $S$ in an elegant way, that would give a new way to think about sedenions.

Posted by: John Baez on December 6, 2014 5:43 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

“Yes, that’s him.”
Ha!

Posted by: Garrett on November 25, 2010 10:30 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

It’s actually Tevian Dray. We took a little pilgrimage to the bridge where Hamilton carved his equations when we were in Dublin for that conference where Hawking conceded his bet on black hole entropy. Alas, Hamilton’s original graffiti was lost behind newer, fresher (in every sense) graffiti. But there was a plaque on the bridge:

so Tevian posed in front of that.

Posted by: John Baez on November 25, 2010 10:46 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

…it turns out we can quantum theory using these Hilbert spaces for either the real numbers, the complex numbers or the quaternions!

Is that missing a ‘do’, or has ‘quantum theory’ become a verb?

Is it that to do quantum theory we need all of the properties of a normed division algebra (and preferably associativity too, or else we are limited to a fragment)? You defined the ‘algebra’ part as a kind of real vector space. Does quantum theory require us to have ‘real’ there?

I guess not since people have worked on p-adic quantum mechanics. But is too much lost then?

Posted by: David Corfield on November 25, 2010 10:43 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

David wrote:

Is that missing a ‘do’, or has ‘quantum theory’ become a verb?

Everything is a verb, man… the universe is, like, just a bunch of verbing.

Seriously, thanks.

Is it that to do quantum theory we need all of the properties of a normed division algebra (and preferably associativity too, or else we are limited to a fragment)?

I think it’ll be a lot better to talk about this after part 2, since that’s when I’ll really start talking about the foundations of quantum mechanics!

You defined the ‘algebra’ part as a kind of real vector space. Does quantum theory require us to have ‘real’ there?

There’s a beautiful theorem that pulls the real numbers (and complex numbers, and quaternions) out of a hat, starting from assumptions that seem to say nothing about the continuum. I’ll talk about that next time, too.

But of course, all theorems have hypotheses, and if we lived in a world governed by p-adic quantum mechanics I bet we could state theorems making that sound inevitable, too.

(Well, at least if such a world allowed for the existence of mathematical physicists!)

Posted by: John Baez on November 25, 2010 11:03 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

There’s a beautiful theorem that pulls the real numbers (and complex numbers, and quaternions) out of a hat, starting from assumptions that seem to say nothing about the continuum.

I’ll try my hardest then to see if you slipped the rabbit in beforehand. Hmm, I seem have a thing about such rabbits at the moment.

Posted by: David Corfield on November 25, 2010 11:34 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

Hmm, I seem have a thing about such rabbits at the moment.

You’re not the only one who seems to have an occasional thing about rabbits.

Posted by: Todd Trimble on November 25, 2010 3:58 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

In math, unlike nature, there is conservation of rabbits. All theorems are tautologies, after all. So, you just have to keep your eye on the hat!

Posted by: John Baez on November 25, 2010 11:52 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Right now, but probably not for very much longer after I have said this, there is a typo where you define unitality.

Posted by: Urs Schreiber on November 25, 2010 1:19 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

and there’s also something slightly less well-known, the concept of state-observable duality

Do you mean what elsewhere would be called the state-operator correspondence ?

(very succinctly in this wiki entry or in more detail for instance in section 2.9 of Polchinski, String Theory , part I)

Posted by: Urs Schreiber on November 25, 2010 1:28 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

I’m thinking of something a bit different, since it involves mixed states rather than pure ones. I’ve never heard anyone talk about it. I’ll explain it in the third episode.

But still, it’s related somehow. (We should talk about how.) And maybe ‘correspondence’ is a better term than ‘duality’. I’ll think about changing it for the paper I’m writing (but not for these blog entries).

Thanks for the reference!

Right now, but probably not for very much longer after I have said this, there is a typo where you define unitality.

Thanks! If I can pull a rabbit out of a hat, surely I can make a typo disappear.

Posted by: John Baez on November 25, 2010 1:43 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

I’m thinking of something a bit different, since it involves mixed states rather than pure ones.

Every trace class operator $A$ gives a state $\rho_A := tr(A \cdot -)/tr(A)$ in the sense of state on an operator algebra.

I’ve never heard anyone talk about it.

Then it must be something different! :-)

Posted by: Urs Schreiber on November 25, 2010 2:29 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Every trace class operator $A$ gives a state $\rho_A := tr(A \cdot -)$ in the sense of state on an operator algebra.

Yeah, that’s related to what I’m going to talk about.

I’ve never heard anyone talk about it.

Then it must be something different! :-)

Well, I guess what I should have said is that I’ve never heard anyone talk about what I plan to say.

But please, let’s not talk about this stuff anymore until Part 3. A good story needs a little suspense.

Posted by: John Baez on November 25, 2010 2:42 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

John wrote:

In an 1898 paper, Hurwitz proved that ℝ, ℂ, ℍ and 𝕆 are the only finite-dimensional normed division algebras.

Isn’t there a deep and mysterious connection to the fact that the only parallelizable spheres are $S^1, S^2$ and $S^7$ , discovered by Michel Kervaire, Raoul Bott and John Milnor in 1958?

Posted by: Tim van Beek on November 25, 2010 3:11 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

At least in one direction, the connection isn’t that deep or mysterious: the norm- $1$ elements in $\mathbb{R}$ , $\mathbb{C}$ , $\mathbb{H}$ and $\mathbb{O}$ are the spheres $S^0$ , $S^1$ , $S^3$ and $S^7$ (with their usual metric coming from the norm), and elements of these spheres act by left multiplication to give isometries of the spheres. The infinitesimal isometries corresponding to one-parameter families of these then give nonwhere-zero vector fields on the spheres.

Posted by: Tim Silverman on November 25, 2010 3:29 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

Michael Atiyah and Jürgen Berndt did some complicated malabar games with this. See http://arxiv.org/abs/math/0206135

Also, Atiyah gave two weeks ago a talk in the IAS relevant to octonions, but nobody has uploaded a set of notes or something so. I am *very* interested on knowing the content of the talk and the reaction of the public.

Posted by: Alejandro Rivero on November 26, 2010 12:15 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

What is interesting from Milnor is the use of this fact, or perhaps of the tools needed to prove this fact, to build a more sophisticated arguments about fiber bundles whose basis is a sphere, the fiber another sphere, and the resulting manifold again an sphere. The biggest beast is S15, but the most interesting is S7.

Posted by: Alejandro Rivero on November 26, 2010 12:20 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Tim wrote:

Isn’t there a deep and mysterious connection to the fact that the only parallelizable spheres are $S^1$ , $S^2$ and $S^7$ , discovered by Michel Kervaire, Raoul Bott and John Milnor in 1958?

$S^2$ isn’t parallelizable: you ‘can’t comb a hairy coconut’. The only parallelizable spheres are $S^0$ , $S^1$ , $S^3$ and $S^7$ . Add one to these numbers and smile.

While the proof of this fact is deep, its connection to division algebras is not. It’s not extremely hard to show that given any way of making $\mathbb{R}^n$ into a division algebra you get a parallelization of $S^{n-1}$ . (It’s incredibly easy to see this for normed division algebras, but there are also other division algebras, and for these it’s a bit more work.) So, an instant consequence of Kervaire, Bott and Milnor’s profound result is that all finite-dimensional division algebras have dimensions 1, 2, 4, or 8. This is a nice example of an algebraic theorem whose only known proof uses topology.

It doesn’t require any topology to prove that the only possible normed division algebras are $\mathbb{R}, \mathbb{C}$ , $\mathbb{H}$ and $\mathbb{O}$ , and indeed Hurwitz did that back in 1898. I know two nice proofs: a string diagram proof and a proof using Clifford algebras.

Kervaire, Bott and Milnor’s work limited the possibilities for division algebras that aren’t normed division algebras.

So, to get a taste of this stuff you need to know one example of a division algebra that’s not a normed division algebra. There are uncountably many so it should be easy to find just one.

A division algebra is just a vector space with a product and multiplicative unit having the property that if $a b = 0$ , either $a = 0$ or $b = 0$ . (Throughout this comment, I’m always talking about vector spaces over the real numbers.)

You can derive quite a bit from this property — like the ability to ‘divide’ — if the algebra is associative. If it’s nonassociative, you have to be much more careful! And all the weird division algebras that aren’t normed division algebras are nonassociative.

So, with no further ado, here’s an example: take the quaternions and modify the product slightly, setting $i^2 = -1 + \epsilon j$ for some small nonzero real number $\epsilon$ while leaving the rest of the multiplication table the same.

This is a 4-dimensional division algebra (in fact an uncountable family of them, one for each $\epsilon$ ), and it’s nonassociative. The number $i$ has a left inverse and a right inverse, but they’re not equal.

As far as I can tell, this gadget, and all the other finite-dimensional division algebras that aren’t normed division algebras, are completely useless. Nonetheless people have tried to classify them, perhaps just because they seem like rather exotic beasts. These papers both have nice expository introductions:

Marion Hübner and Holger Petersson, Two-dimensional real division algebras revisited.
Erik Darpö and Ernst Dietrich, Classification of the real commutative division algebras

There are also vast collections of infinite-dimensional division algebras, many of which are commutative, associative and quite useful — but none of which are normed.

Posted by: John Baez on November 26, 2010 12:57 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

John said:

$S^2$ 2 isn’t parallelizable: you ‘can’t comb a hairy coconut’. The only parallelizable spheres are $S^0, S^1, S^3$ and $S^7$ .

Whoops: typo.

The hairy ball theorem is linked to from the article about the (more general)

Poincaré-Hopf theorem, Wikipedia.

(The German version is “every continuously combed hedgehog has a bald patch”, which is less convincing because hedgehogs aren’t barbed everywhere anyway).

Posted by: Tim van Beek on November 26, 2010 1:40 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Tim wrote:

hedgehogs aren’t barbed everywhere anyway.

Maybe that theorem explains why.

Down here in Singapore we have more coconuts than hedgehogs, but they still have ‘eyes’.

Posted by: John Baez on November 27, 2010 8:33 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Tangential note on this part:

This is supposed to resolve the old puzzle about why Nature picked $\mathbb{C}$ when it was time for quantum mechanics, while turning up her nose at $\mathbb{R}$ and $\mathbb{H}$ .

Goyal et al. might say that the answer is, “Because a primitive notion of complementarity implies the Feynman rules”. There’s some fun discussion of different kinds of composition in their paper, which a category theorist might be able to do something interesting with.

Posted by: Blake Stacey on November 25, 2010 6:25 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

The paper by Goyal et al. looks interesting. I’ll admit that at first glance, I’m underwhelmed by the idea of deriving the role of complex numbers in quantum mechanics starting from this:

Pair Postulate: each sequence of measurement outcomes obtained in a given experiment is represented by a pair of real numbers, where the probability associated with this sequence is a continuous, non-trivial function of both components of this real number pair.

As we’ll see later in this story, various axiomatic schemes for quantum mechanics pick out $\mathbb{R}, \mathbb{C}$ and $\mathbb{H}$ as special. So for all those schemes, as soon as you say you’re especially fond of pairs of real numbers, you’re down to $\mathbb{C}$ .

But why pairs?

I bet there’s good stuff in the Goyal et al. paper. But it would be interesting to see what would happen if they replaced pairs by quadruples: would they get the quaternions? Or triples: would that assumption lead to a contradiction?

Posted by: John Baez on November 26, 2010 2:55 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

As to the question of “why complex numbers”, Scott Aaronson gave several reasons.

Posted by: Mike Stay on November 27, 2010 11:37 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Thanks, Mike! Aaronson gives some references at the end, including to Lucien Hardy’s paper Quantum theory from five reasonable axioms, and also a paper by Caves, Fuchs and Schack, both of which give some reasons for preferring complex quantum mechanics. My own theory, explained in the paper these blog posts are coming from, is that real, complex and quaternionic quantum mechanics are all true.

Posted by: John Baez on November 28, 2010 7:44 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

My first hunch is that as you increase the size of your tuple, you increase the number of conditions necessary to specify the algebraic rules by which tuples must be combined, so instead of getting a contradiction (when the tuple size is wrong for a normed division algebra, say) you would just be unable to decide definitively on how to combine your numbers. Goyal et al. use three items of input: how sequence composition works in series, how sequence composition works in parallel and that tuples map to probabilities according to a continuous real-valued function which depends nontrivially on each tuple element. I suspect the series composition wouldn’t provide the same constraint in the case of a larger tuple, but that’s just a guess on my part.

Posted by: Blake Stacey on November 26, 2010 5:48 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

You say “The answer is that she didn’t: she greedily chose all three!” Are the actually examples of physical systems which use real numbers or quaternions in the way you are discussing? It seems to me that you’ve explained how all three give mathematically consistent systems, but you haven’t argued that nature uses them all.

Posted by: David Speyer on November 30, 2010 3:35 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

David wrote:

Are the actually examples of physical systems which use real numbers or quaternions in the way you are discussing?

Yes.

It seems to me that you’ve explained how all three give mathematically consistent systems, but you haven’t argued that nature uses them all.

Right. That’s what my paper ‘Division algebras and quantum theory’ will do. These blog entries on ‘state-observable duality’ are taken from that paper — but they’re about a smaller story, namely the story of formally real Jordan algebras and how these are secretly the same as a certain class of pointed self-dual convex cones.

Posted by: John Baez on December 1, 2010 3:25 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

There is a clever proof using string diagrams of some statement vaguely like “any associative normed division algebra must have dimension 1, 2, or 4.” The idea is to note that the multiplication of a NDA induces both a “dot product” and a “cross product” on its “purely imaginary” subspace (the complement of the span of 1), write some string diagram identities involving the resulting cross product, use them to evaluate a particular string diagram down to a composite of loops in two different ways, and use the fact that a loop represents the dimension of the underlying vector space to deduce a polynomial equation which the dimension must satisfy, whose only roots are 0, 1, and 3. However, I am currently failing to reconstruct the argument; does anyone know it, or know where to find it?

Posted by: Mike Shulman on November 25, 2010 11:16 PM | Permalink | PGP Sig | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

There’s a really fun argument that also covers the nonassociative case. I like it so much that presented it to the category theory seminar in Cambridge — I think Tom Leinster and Eugenia Cheng were there, but I know Martin Hyland and Peter Johnstone were. (Apparently fear is an aid to memory.)

This argument was developed by Dominik Boos, based on ideas of Markus Rost — for a summary with links see week169. More recently, Bruce Westbury wrote a really great exposition of the argument, probing into various interesting side-issues:

Bruce W. Westbury, Hurwitz’s Theorem.

One of the wonderful things is that Bruce starts with a purely diagrammatic argument showing that if you have a normed division algebra whose space of imaginary elements has dimension $d$ , you get a solution of the equation

$d(d-1)(d-3)(d-7) = 0$

Then, by manipulating these diagrams some more, he shows that either $d = 7$ or the algebra is associative.

Then, in the associative case, he shows that either $d = 3$ or the algebra is commutative.

I should add, for those not in the know, that these arguments cover not just normed division algebras but the somewhat more general ‘composition algebras’. These are a purely algebraic concept, whereas the concept of a norm involves some inequalities. And even over the reals, it includes a few more examples.

For example, there are two 8-dimensional real composition algebras: the octonions, and the split octonions. I hope that someday John Huerta and James Dolan will publish, or at least publicize, their work on ‘split octonions and the rolling ball’.

(By the way, Mike: every time I quickly glance at your comment, I think it mentions looped strands of DNA. Then, when I look at it carefully, that illusion goes away.)

Posted by: John Baez on November 26, 2010 2:20 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Awesome, thanks! I added some links to composition algebra and normed division algebra.

Posted by: Mike Shulman on November 26, 2010 4:45 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

The links took me to ncatlab.org with a picture of a pretty girl. I don’t think this is what was intended.

Posted by: Bruce Westbury on November 29, 2010 11:33 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

The reason is a temporary domain problem as explained in this comment.

Posted by: Tim van Beek on November 29, 2010 11:57 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

I was there, and a very memorable seminar it was too.

In fact, I used the Boos–Rost argument as an example of graphical reasoning in a talk I gave many years later to the Cambridge Philosophy of Mathematics seminar. It was called The peculiar traits of human mathematics, and an excerpt of their argument appears on slide 22.

Posted by: Tom Leinster on November 27, 2010 7:20 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

My paper is now on the arXiv.
http://arxiv.org/abs/1011.6197

Posted by: Bruce Westbury on November 30, 2010 8:00 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Great! During this discussion I realized to my horror that your paper was only available on my website. I’m glad that’s no longer the case.

Posted by: John Baez on November 30, 2010 12:52 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

Adler did a lot of work on quaternions for quantum mechanics. It could be relevant.

Posted by: Alejandro Rivero on November 26, 2010 12:22 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Yes, I read his book once. My only complaint was in his treatment of joint systems and Fock spaces.

As you know, combining two quantum systems into a ‘joint system’ requires that you tensor their Hilbert spaces. Adler’s quaternionic Hilbert spaces are one-sided modules over the quaternions, but he tries to tensor two of them and get another such Hilbert space. Mathematicians know that you shouldn’t tensor two one-sided modules of a noncommutative ring and hope to get another such module. So his procedure seems rather unnatural.

An alternative approach is to treat quaternionic Hilbert spaces as bimodules over the quaternions. Mathematicians know that you can tensor two bimodules and get another bimodule. In fact, Adler’s procedure seems to involve choosing a bimodule structure, without making this entirely explicit. There’s not a canonical choice, and given any choice of bimodule structure you can easily get a bunch of other choices by ‘twisting’ with an element of $Aut(\mathbb{H}) = SO(3)$ .

For more see:

• Toby Bartels, Functional analysis with quaternions.

In the paper I’m writing now, I’m using another alternative approach. But I think there’s a lot left to understand here. My first goal is to show that real and quaternionic quantum mechanics are in fact visible in nature, so that studying them is not a purely ‘academic’ pursuit (in some insulting sense of the term ‘academic’).

Posted by: John Baez on November 26, 2010 1:54 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

By the way, in case the right sort of algebraist happens to read this:

Am I correct in believing that the main claim of the Wikipedia article Albert algebra is false? They say “Over the real numbers, there is only one such Jordan algebra up to isomorphism.” I believe there are two: the $3 \times 3$ self-adjoint matrices with octonion entries, and the $3 \times 3$ self-adjoint matrices with split octonion entries. I’d like to correct this; I hope I’m not under some sort of delusion.

Posted by: John Baez on November 26, 2010 3:43 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

Indeed the split octonions are interesting. They already arise in the study of M-theory compactifications, giving the U-duality groups O(5,5) and E6(6) for M-theory wrapped on T^⁵ and T^⁶ respectively. This is done by noticing that O(5,5) and E6(6) are just the determinant preserving groups for the 2×2 and 3×3 Jordan algebras of Hermitian matrices over the split-octonions, J(2,Os) and J(3,Os).

Gauge bosons are in the 27 of E6(6), which under O(5,5) decomposes as 27→10+16+1. Particle excitations carrying the 10 charges are Kaluza-Klein and winding strings. States in the 16 arise from ways of wrapping Dp-branes to give D-particles: 10 for D2, 5 for D4 and 1 for D0. The state carrying the 1 charge corresponds to the NS5-brane wrapped completely on the T^⁵.

Jordan algebraic minded folks will notice the 27->10+16+1 is the decomposition of J(3,Os) when E6(6) fixes a point of the 16-dimensional split Cayley plane, thus identifying an O(5,5) subgroup. This O(5,5) subgroup acts on elements of 27-dimensional J(3,Os) via J(2,Os) vectors in the 10, which fix a diagonal entry in the 1.

Posted by: Mike Rios on November 26, 2010 3:42 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Fascinating, fascinating! There are a lot of questions I could ask. But for starters: am I right that the main claim in that short Wikipedia article is false?

Posted by: John Baez on November 27, 2010 1:51 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

Indeed things get even more fascinating when one notices the split octonions are a real subalgebra of the bioctonions. See the paper by Shukuzawa.

The main claim in the article appears to be false as the exceptional Jordan algebra and the split exceptional Jordan algebra are both 27-dimensional Albert algebras over the reals.

Posted by: Mike Rios on November 27, 2010 7:41 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Mike Roos wrote:

The main claim in the article appears to be false as the exceptional Jordan algebra and the split exceptional Jordan algebra are both 27-dimensional Albert algebras over the reals.

In that case, the two algebras must be isomorphic. That there is only one exceptional 27-dimensional Jordan algebra is a widely known fact, proved, e.g., in the book

Jacques Faraut, Adam Korányi, Analysis on symmetric cones, 1994.

Posted by: Arnold Neumaier on November 30, 2010 8:42 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Mike wrote:

The main claim in the article appears to be false as the exceptional Jordan algebra and the split exceptional Jordan algebra are both 27-dimensional Albert algebras over the reals.

Arnold wrote:

In that case, the two algebras must be isomorphic. That there is only one exceptional 27-dimensional Jordan algebra is a widely known fact, proved, e.g., in the book

• Jacques Faraut, Adam Korányi, Analysis on symmetric cones, 1994.

Oh, good — a controversy!

I got this book out of the library on your recommendation, Arnold, and it looks very nice: much more readable than, say, Max Koecher’s book. Could you point me to a result stating that there’s only one 27-dimensional exceptional Jordan algebra over the reals?

I know that there’s only one formally real 27-dimensional Jordan algebra over the reals that is ‘exceptional’ in the technical sense of not being embeddable in an associative algebras. (Faraut and Korányi use the term ‘Euclidean’ as a synonym for ‘formally real’.)

However, I believe that when we drop the ‘formally real’ condition, we get two.

Mike Rios might be able to prove that these two are nonisomorphic by showing that their automorphism groups are different real forms of $\mathrm{F}_4$ . An easier way might be to calculate the signature of the bilinear form

$tr(L_x L_y)$

where $L_x$ is left multiplication by $x$ .

Posted by: John Baez on December 1, 2010 11:04 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

JB: However, I believe that when we drop the ‘formally real’ condition, we get two.

Possibly yes. Indeed, Faraut and Koranyi only classify the Euclidean ones. It is only these that correspond to symmetric cones, hence these are those of physical interest. The Euclidean exceptional algebra in 27 dimensions is the Albert algebra.

I don’t know about non-Euclidean ones, and take back my too general claim. Still, it would be interesting to decide the question whether or not these algebras are isomorphic (and if they are, to exhibit the Euclidean structure of the split case).

Posted by: Arnold Neumaier on December 1, 2010 7:54 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

That the Euclidean exceptional Jordan algebra and the split exceptional Jordan algebra are non-isomorphic, can be shown in many different ways. Most approaches are a result of the octonions and split-octonions being non-isomorphic. This was actually formalized in 1957 by Albert and Jacobson in:

A. A. Albert, N. Jacobson, On Reduced Exceptional Simple Jordan Algebras.

Recall that a formally real Jordan algebra is a commutative and power-associative algebra J satisfying:

$x^2_1+...+x^2_n=0\quad\Rightarrow\quad x_1=...=x_n=0\quad \forall n\in\mathbb{N}^{\ast}.$

Since elements of the split exceptional Jordan algebra contain split-octonion off-diagonal entries, there exist non-trivial nilpotents in the algebra, which violate the formally real property. Such non-trivial nilpotents also prevent the trace bilinear form $tr(x\circ y)$ from being positive definite over the split exceptional Jordan algebra. Hence, the Euclidean exceptional Jordan algebra and split exceptional Jordan algebra have different signatures under this trace bilinear form, each individually preserved by different real forms of $F_4$ .

Posted by: Mike Rios on December 19, 2010 5:15 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Okay, I’ll try to fix that Wikipedia article.

Indeed things get even more fascinating when one notices the split octonions are a real subalgebra of the bioctonions.

Yes — as you probably know, the real composition algebras in dimensions 2, 4, and 8 all come in two nonisomorphic real forms, which become isomorphic when you complexify them. So we have

which along with the usual complex numbers, quaternions and octonions are real subalgebras of the

bicomplex numbers $\mathbb{C} \otimes \mathbb{C}$ ,
biquaternions $\mathbb{C} \otimes \mathbb{H}$ and
bioctonions $\mathbb{C} \otimes \mathbb{O}$ ,

respectively. And in this well-organized world of ours they all have Wikipedia pages — though the bicomplex numbers are listed under the absurd name ‘tessarines’.

But the bicomplex numbers are isomorphic to $\mathbb{C} \oplus \mathbb{C}$ , and the biquaternions are isomorphic to $2 \times 2$ complex matrices, so they scarcely warrant special names — while the bioctonions are really something new.

But you just reminded me: in addition to the two 27-dimensional exceptional Jordan algebras over $\mathbb{R}$ that we’ve just been talking about, there’s also another one, which is 54-dimensional: namely, the complexification of either of these two! Right? It’s only over algebraically closed fields that there’s just a single exceptional Jordan algebra, which is 27-dimensional.

Anyway, I will try to err on the side of correctness rather than completeness when fixing that Wikipedia article.

Posted by: John Baez on November 27, 2010 8:15 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Yes, the two 27-dimensional exceptional Jordan algebras over $\mathbb{R}$ are both contained in the exceptional Jordan $C^\ast$ -algebra, i.e. Jordan algebra of 3x3 Hermitian matrices over the bioctonions. This is part of a more general result that each JB-algebra is the self-adjoint part of a unique Jordan $C^\ast$ -algebra. See:

J. D. M. Wright, Jordan $C^*$ -algebras.

Elements of the exceptional Jordan $C^\ast$ -algebra become self-adjoint under a Hermitian transpose that uses ‘octonionic conjugation’ on the matrix entries. Octonionic conjugation transforms a bioctonion as: $\psi=\varphi_1+i\varphi_2\rightarrow \overline{\psi}=\overline{\varphi_1}+i\overline{\varphi_2}$ . Using octonionic conjugation one recovers a complex valued norm $\eta(\psi\overline{\psi})=|\psi|^2$ , which reduces to the anisotropic and isotropic real valued norms over the octonions and split-octonions. These properties lift to give a complex valued inner product on the exceptional Jordan $C^\ast$ -algebra $\langle X,Y\rangle=tr(X \circ Y\dagger)=tr(X\circ Y)$ , from which we recover a Frobenius norm $|X|^2=tr(X\circ X^\dagger)=tr(X^2)$ . We can later use this inner product to define a complex valued quartic form on the Freudenthal triple system for the exceptional Jordan $C^\ast$ -algebra.

Posted by: Mike Rios on November 27, 2010 6:14 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

A quick technical note: $\langle X, Y\rangle=tr(X \circ Y)$ and $\|X\|^2=tr(X^2)$ only reduce to a true inner product and norm over the exceptional Jordan algebra. Generally, over the full exceptional Jordan $C^*$ -algebra (and hence the split exceptional Jordan algebra) $\langle X, Y\rangle=tr(X \circ Y)$ is merely a symmetric bilinear form, as there exist non-zero elements for which $\|X\|^2=tr(X^2)=0$ .

For the bioctonions, $|\psi|^2=\psi\overline{\psi}=\overline{\psi}\psi$ arises from a non-degenerate quadratic form for which the bioctonions form a composition algebra over $\mathbb{C}$ . This quadratic form reduces to the usual inner product and norm over the octonions.

Posted by: Mike Rios on November 28, 2010 12:59 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

I was looking through Hamilton’s paper on quaternions, because I couldn’t figure out what he meant by saying that (excuse my Fortran)

i**2 = j**2 = k**2 = ijk = -1

I mean, if i**2 = -1, then i = sqrt(-1) …. but it turns out that i can be set equal to a bunch of different kinds of things, and its square can come out to equal -1, as Hamilton showed in one of his papers, so that’s OK, and so is ijk = -1. But Hamilton also stated that ij = k, while ji = -k, making ij = -ji, and likewise for jk and ik, and he said the proof was long and complicated or something to that effect, so he was omitting it.

So can anyone here prove that for quaternions, ij = -ji (and likewise for jk and ik)? It’s probably simple and elementary, but I’d like to see it, just for fun…

Posted by: streamfortyseven on November 27, 2010 11:17 AM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

streamfortyseven, starting from

$i^2 = j^2 = k^2 = i j k = -1$

one gets

$-i j = i j k k = (-1)k$

so $i j = k$ . Hence $i j i j = k^2 = -1$ . Also

$i j j i = i(-1)i = - i^2 = 1$

and therefore $i j i j = -i j j i$ , so $i j = - j i$ by cancellation. All others in the multiplication table on the basis ${1, i, j, k}$ follow by similar reasoning.

Posted by: Todd Trimble on November 27, 2010 8:14 PM | Permalink | Reply to this

Re: State-Observable Duality (Part 1)

$MathML-enabled post (click for more details).$

Of course there are lots of ways to show $i j = - j i$ starting from Hamilton’s famous relations

$i^2 = j^2 = k^2 = i j k = -1$

For example, if you start with

$i j k = -1$

and multiply both sides on the right by $-k$ , you get

$i j = k$

But if you multiply both sides on the left by $i$ , you get

$-j k = -i$

and then multiplying on the left by $j$ gives

$k = - j i$

so we see $i j = -j i$ .

Obviously Hamilton had thought about these matters long enough that when he had his final moment of insight, he was able to formulate the necessary equations in a cute — almost too cute — ‘minimalistic’ way, instead of writing down the whole quaternion multiplication table.

I’m sure most of you know what he wrote about the moment on October 16th, 1843 when he carved these equations into that bridge on the Royal Canal:

That is to say, I then and there felt the galvanic circuit of thought close; and the sparks which fell from it were the fundamental equations between i,j,k; exactly such as I have used them ever since.

But not everyone remembers that he was in the process of walking with his wife to a meeting of the Royal Irish Academy, of which he was the head. I pity his wife for having to stand by and watch him have his self-absorbed ‘moment of genius’ — especially given the back story.

At the age of 19, Hamilton met a woman named Catherine Disney and became deeply infatuated with her, and she with him. However, her parents forced her to marry a wealthy man 15 years older than her. He remained hopelessly in love with her the rest of his life, and wrote many poems about her. In fact he was friends with Coleridge, who introduced him to Wordsworth, who advised him against going into poetry, saying his talents lay in science.

At the age of 28, he married another woman, Helen Bayly. When he was made head of the Royal Irish Academy, one of his jobs was to run a small observatory in Dublin. However, he quickly lost interest in staying up nights to do observations, and he got Helen to do the job. In his later years he became an alcoholic, then foreswore drink, then relapsed. Helen became a semi-invalid, suffering from ill-defined nervous complaints. Eventually she died.

Later, Catherine began a secret correspondence with Hamilton — she still loved him! Her husband became suspicious, she attempted suicide by taking laudanum… and then, five years later, she became ill. Hamilton visited her and gave her a copy of his Lectures on Quaternions — they kissed at long last — and then she died two weeks later. He carried her picture with him ever afterwards and talked about her to anyone who would listen.

Hamilton died on September 2, 1865, following a severe attack of gout precipitated by excessive drinking and overeating. Huge stacks of mathematical papers were found on his desk, with bones of mutton chops between some of them.

Posted by: John Baez on November 28, 2010 12:59 AM | Permalink | Reply to this

Read the post The Three-Fold Way (Part 5)
Weblog: The n-Category Café
Excerpt: Learn about the functors going between the categories of real, complex and quaternionic Hilbert spaces.
Tracked: February 8, 2011 3:24 AM

The n-Category Café

Skip to the Main Content

November 25, 2010