The Three-Fold Way (Part 4)

January 29, 2011

Posted by John Baez

$MathML-enabled post (click for more details).$

It’s been more than a month since the last post in this thread… so let me remind you of the story so far.

I showed you how real and quaternionic quantum mechanics are lurking inside good old, ordinary complex quantum mechanics. Ordinary physicists only like complex Hilbert spaces. For example, they describe elementary particles using irreducible unitary representations of groups. And these are representations on complex Hilbert spaces. They couldn’t care less about real or quaternionic Hilbert spaces.

But — lo and behold! — the math gods have decreed that irreducible unitary representations come in three kinds:

The real ones. These are the ones that you get by complexifying representations on real Hilbert spaces. These guys are isomorphic to their own dual. In fact, any representation $H$ of this kind comes with an invariant nondegenerate bilinear function $g: H \times H \to \mathbb{C}$ with $g(v,w) = g(w,v) .$
The quaternionic ones. These are the ones that you get by taking representations on quaternionic Hilbert spaces, and thinking of them as complex Hilbert spaces. These guys are also isomorphic to their own dual. In fact, any representation $H$ of this kind comes with an invariant nondegenerate bilinear map $g: H \times H \to \mathbb{C}$ with $g(v,w) = -g(w,v) .$
The truly complex ones. These are the ones that aren’t isomorphic to their own dual.

So, even if we don’t want to think about real and quaternionic Hilbert spaces, the math gods are hinting that we should!

And indeed, I showed you that an electron is a quaternion.

Well, that’s just a deliberately overdramatic way of putting it. More precisely, I showed you that any irreducible unitary representation of SU(2) is either real or quaternionic. And the famous ‘spin-1/2’ representation, which we use to describe electrons, is quaternionic. We usually describe the state of a spin-1/2 particle using two complex numbers. But we could also use a single quaternion!

Now, you might think this is a completely trivial statement, since you can take two complex numbers and combine them into one quaternion. That’s part of the idea — but what I’m saying is not quite that trivial. What I’m saying is that $SU(2)$ acts as quaternion-linear transformations that preserve the inner product on a 1-dimensional quaternionic Hilbert space — and that’s the spin-1/2 representation in disguise.

In fact all the half-integer-spin or ‘fermionic’ representations of SU(2) are quaternionic, while all its integer-spin or ‘bosonic’ representations are real.

I also showed you that a unitary group representation is quaternionic if and only if it comes equipped with an antiunitary operator $J : H \to H$ that commutes with all the group operations and obeys $J^2 = -1 .$ That’s not surprising: this operator is just multiplication by the quaternion $j$ . But the cool part is that a unitary group representation is real if and only if it has a $J$ with all the same properties, except now $J^2 = 1.$ Now this operator comes from complex conjugation!

What’s the physical meaning of this operator $J$ ? For representations of $SU(2)$ , it’s time reversal: it reverses the angular momentum!

Okay, now you’re caught up.

$MathML-enabled post (click for more details).$

Last time I ended with a puzzle:

It seems natural that time reversal should obey $J^2 = 1$ , as it does in the bosonic. It may seem strange to have $J^2 = -1$ , as we do for fermions. Should not applying time reversal twice get us back where we started? It actually does, in a crucial sense: the expectation values of all observables are unchanged when we multiply a state by $-1$ . So, time reversing an electron twice gets us an electron that’s physically indistinguishable from the one we started with. Still, the minus sign seems a bit curious.

But it should remind you of the equally curious minus sign that a fermion picks when you rotate it a full turn!

Are these signs related?

The answer appears to be yes, though the following argument is more murky than I’d like. It has its origins in Feynman’s 1986 Dirac Memorial Lecture on antiparticles, together with the well-known ‘belt trick’ for demonstrating that $SO(3)$ isn’t simply connected — which is why we need its double cover $SU(2)$ to describe rotations in quantum theory. The argument uses string diagrams, which are a generalization of Feynman diagrams.

The basic ideas apply to any Lie group $G$ . If an irreducible unitary representation $H$ of this group is isomorphic to its dual, it comes with an invariant nondegenerate pairing $g : H \otimes H \to \mathbb{C}$ We can draw this as a ‘cup’:

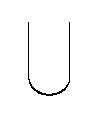
If this were a Feynman diagram, it would depict two particles of type $H$ coming in on top and nothing going out at the bottom: since $H$ is isomorphic to its dual, particles of this type act like their own antiparticles, so they can annihilate each other.

Since $g$ defines an isomorphism $H \cong H^*$ , one can show there’s a linear map back from $\mathbb{C}$ to $H \otimes H$ , which we draw as a ‘cap’:

uniquely determined by requiring that the cap and cup obey the zig-zag identities:

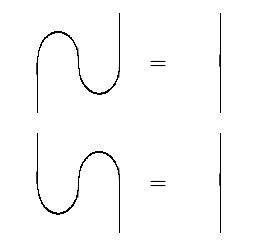
Indeed, for any $H \in Rep(G)$ , we have a ‘cup’ $H^* \otimes H \to \mathbb{C}$ and ‘cap’ $\mathbb{C} \to H \otimes H^*$ obeying the zig-zag equations: in the language of category theory, we say that $Rep(G)$ is compact closed. But when $H$ is isomorphic to its dual, we can write the cap and cup using just $H$ .

As we have seen, there are are two choices. If $H$ is real, $g$ obeys $g(v,w) = g(w,v).$ Using string diagrams, we can draw this equation as:

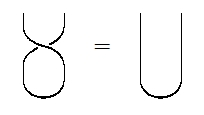
But if $H$ is quaternionic, we have $g(v,w) = -g(w,v).$ which we can draw as:

The power of string diagrams is that we can apply the same diagrammatic manipulation to both sides of an equation and get a new equation. Take the pictures I just drew, turn them clockwise a bit and stretch out the string a little! Then when $H$ is a real representation we get:

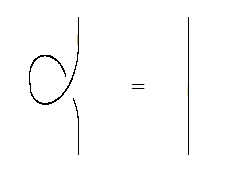
while when $H$ is quaternionic we get:

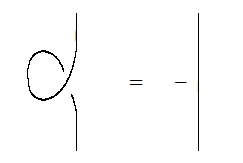
Both sides of these equations describe operators from $H$ to itself. The vertical straight line at right corresponds to the identity operator $1: H \to H .$ If you do the math, the more complicated diagram at left turns out to correspond to the operator $J^2$ . So, it follows that $J^2 = 1$ when the representation $H$ is real, while $J^2 = -1$ when $H$ is quaternionic. Of course I already told you this… but now I’m sketching a picture proof.

While it may seem puzzling to those who have not been initiated into the mysteries of string diagrams, everything so far is perfectly rigorous. Now comes the slightly murky part! The string diagram at left:

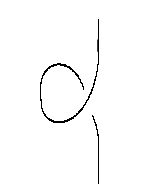
looks like a particle turning back in time, going backwards for a while, and then turning yet again and continuing forwards in time. In other words, it looks like a picture of the square of time reversal! So, the fact that the corresponding morphism $J^2$ is indeed the square of time reversal when $G = SU(2)$ somehow seems right.

Moreover, in the theory of string diagrams it is often useful to draw the strings as ‘ribbons’. This allows us to take the diagram at left and pull it tight, as follows:

At left we have a particle (or actually a small piece of string) turning around in time, while at right we have a particle making a full turn in space as time passes. So, in the case $G = SU(2)$ it seems to make sense that $J^2 = 1$ when the spin $j$ is an integer: after all, the state vector of an integer-spin particle is unchanged when we rotate that particle a full turn. Similarly, it seems to make sense that $J^2 = -1$ when $j$ is a half-integer: the state vector of a half-integer-spin particle gets multiplied by $-1$ when we rotate it by a full turn.

So, we seem to be on the brink of having a ‘picture proof’ that the square of time reversal must match the result of turning a particle 360 degrees! Unfortunately this argument is not yet rigorous, since we have not explained how the topology of ribbon diagrams (well-known in category theory) is connected to the geometry of rotations and time reversal. We started out talking about group representations… but somehow spacetime snuck into our argument. Can you explain what’s going on?

While our discussion here focused on the group $SU(2)$ , real and quaternionic representations of other groups are also important in physics. For example, gauge bosons live in the adjoint representation of a compact Lie group $G$ on the complexification of its own Lie algebra; since the Lie algebra is real, this is always a real representation of $G$ . This is related to the fact that gauge bosons are their own antiparticles.

In the Standard Model, fermions are not their own antiparticles, but in some theories they can be. Among other things, this involves the question of whether the relevant spinor representations of the groups $\Spin(p,q)$ are complex, real (‘Majorana spinors’) or quaternionic (‘pseudo-Majorana spinors’). The options are well-understood, and follow a nice pattern depending on the dimension and signature of spacetime modulo 8. We should emphasize that the spin groups $Spin(p,q)$ are not compact unless $p = 0$ or $q = 0$ , so their finite-dimensional complex representations are hardly ever unitary, and many of the results I’ve been explaining don’t apply. Nonetheless, we may ask if such a representation is the complexification of a real one, or the underlying complex representation of a quaternionic one—and the answers have implications for physics.

$SU(2)$ is not the only compact Lie group with the property that all its irreducible unitary representations on complex Hilbert spaces are real or quaternionic! For a group to have this property, it is necessary and sufficient that every element be conjugate to its inverse. All compact simple Lie groups have this property except those of type $A_n$ for $n \gt 1$ , $D_n$ with $n$ odd, and $E_6$ . I learned this from the nice people over on Mathoverflow. And for the symmetric groups $S_n$ , the orthogonal groups $\mathrm{O}(n)$ , and the special orthogonal groups $SO(n)$ , all representations are in fact real.

So there is a rich supply of real and quaternionic group representations, which leave their indelible mark on physics even if we think we are doing complex quantum theory!

Next time I want to say more about how real, complex and quaternionic quantum theory fit together. I’ve made it sound like complex quantum theory is the main thing, with the real and quaternionic theories sitting inside it. But that’s just part of the story. To see the whole story, we should use a bit more category theory.

Posted at January 29, 2011 12:43 PM UTC

TrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/2349

73 Comments & 1 Trackback

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

As usual, when I blog about something I think about it harder and later notice errors in what I said. Usually I correct them but this time I’ll leave it as a puzzle, at least for now.

What mathematical mistake did I make above? I’m not talking about poetic exaggerations like “an electron is a quaternion” — I’m talking about a straightforward mathematical statement that happens to be false.

Posted by: John Baez on January 31, 2011 12:18 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

This isn’t a mathematical mistake, but I think your cap and cup are the wrong way round; also, I think there’s a “\iso” that should be “\cong”.

Posted by: Tim Silverman on January 31, 2011 12:41 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Thanks for catching those errors. Fixed!

(But not the mistake I was talking about.)

Posted by: John Baez on January 31, 2011 2:40 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

Don’t the strings in the rotated diagrams cross in the wrong way (the one extending to the top should be behind the one extending to the bottom)? But that wouldn’t affect the rest of the argument, would it?

Posted by: Stefan on January 31, 2011 1:36 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

Thanks! I’ve fixed the figures so that now rotating this clockwise:

and stretching it gives this:

Thanks for catching that!

Of course, the original suboptimal picture didn’t affect the actual argument, since in this context there’s no difference between an overcrossing and an undercrossing. However, it’s good to have nice pictures… and there would be a difference between an overcrossing and undercrossing if we were working in a braided monoidal category, like the category of representations of a quantum group. These show up in quantum field theory when you’ve got 2 space dimensions and one time dimension.

(I’m still waiting for someone to find the erroneous statement I mentioned. But it’s great to ask people to find an error you noticed. They find all sorts of ones you hadn’t!)

Posted by: John Baez on January 31, 2011 2:49 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Programmers call that technique bebugging.

Posted by: Mike Stay on February 3, 2011 10:23 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Ordinary physicists only like complex Hilbert spaces. […] They couldn’t care less about real or quaternionic Hilbert spaces. […] And indeed, I showed you that an electron is a quaternion .

Maybe you mentioned this before, but maybe it deserves to be mentioned again: this very observation that the electron is a quaternion has been the starting point for David Hestenes and a school of followers to rethink physics in terms of Hilbert spaces more general than complex ones (they call this “Physics by Geometric Algebra”), or at least rethink the standard textbook exposition of that phyiscs.

Somewhere in his writings Hestenes gives a moving account of what was literally his awakening experience when as a student he realized fully that “the electron is a quaternion”.

As it goes with awakening experiences, their intensity may hinder their communication to the unenlightened, and possibly with less emphasis on the extraordinary and more translation into the standard math language the Geometric Algebra school might have meanwhile actually succeeded to rewrite the standard textbooks and not just their own non-standard textbooks.

Posted by: Urs Schreiber on January 31, 2011 1:38 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Urs wrote:

Somewhere in his writings Hestenes gives a moving account of what was literally his awakening experience when as a student he realized fully that “the electron is a quaternion”.

I’d like to find that story.

Yes, it’s unfortunate that Geometric Algebra has developed into a kind of separate ‘school’, especially given that the same mathematics is available in the ‘standard’ approach, but mostly available in fairly sophisticated texts like Michelson and Lawson’s Spin Geometry. Somehow we need to get Clifford algebra integrated into the standard college curriculum at a lower level.

Posted by: John Baez on February 1, 2011 1:50 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Somewhere in his writings Hestenes gives a moving account of what was literally his awakening experience when as a student he realized fully that “the electron is a quaternion”.

I’d like to find that story.

I have found it again: it’s at the beginning of

David Hestenes, Clifford algebra and the interpretation of quantum mechanics (pdf).

He starts by recalling his interest in philosophy and physics and then writes

Immediately after passing my graduate comprehensive examinations I was awarded a research assistantship with no strings attached, whereupon, I disappeared from the physics department for nearly a year. My father got me an isolated office on the fourth floor of the mathematics building where I concentrated intensively on my search for a coherent mathematical foundation for theoretical physics. One day, after about three months of this, I sauntered into a nearby math-engineering library and noticed on the “New Books shelf” a set of lecture notes entitled Clifford Numbers and Spinors by the mathematician Marcel Riesz [1]. After reading only a few pages, I was suddenly struck by the realization that the Dirac matrices could be regarded as vectors, and this gives the Dirac algebra a geometric meaning that has nothing to do with spin. The idea was strengthened as I eagerly devoured the rest of Reisz’s lecture notes, but I saw that much would be required to implement it consistently throughout physics. That’s what got me started.

About two months later, I discovered a geometrical meaning of the Pauli algebra which had been completely overlooked by physicists and mathematicians. I went excitedly to my father and gave him a lecture on what I had learned. The following is essentially what I told him, with a couple of minor additions which I have learned about since.

Then he gives some discussion on $Cl(\mathbb{R}^3)$ and its use in the Dirac equation, only that he calls is the “geometric algebra $\mathcal{R}_3$ ”.

After this little technical bit, he continues:

These observations about the Pauli algebra reveal that is has a universal significance that physicists have overlooked. It is not just a “spinor algebra” as it is often called. It is a matrix representation for the geometric algebra $\mathcal{R}_3$ , which, as was noted in my first lecture, is no more and no less than a system of directed numbers representing the geometrical properties of Euclidean 3-space. The fact that vectors in $\mathbb{R}^3$ can be represented as hermitian matrices in the Pauli algebra has nothing whatever to do with their geometric interpretation. It is a consequence of the fact that multiplication in $\mathcal{R}_3$ is associative and every associative algebra has a matrix represention. This suggests that we should henceforth regard the $\mathbf{\sigma}^k$ only as vectors in $\mathbb{R}^3$ and dispense with their matrix representations altogether, because they introduce extraneous artifacts like imaginary scalars.

I wondered aloud to my father how all this had escaped notice by Herman Weyl and John von Neumann, not to mention Pauli, Dirac and other great physicists who has scrutinized the Pauli algebra so carefully. When I finished my little talk, my father gave me a compliment which I remember word for word to this day, because he never gave such compliments lightly. He has always been generous with his encouragement and support, but I have never heard him extend genuine praise for any mathematics which did not measure up to his own high standards. He said to me, “you understand the difference between a mathematical concept and its representation by symbols. Many mathematicians never learn that.”

Not sure if you all find this account “moving” as I claimed it is. I think I found it moving back then, because I knew the kind of intense feeling this is driven by.

Of course everything he says is very “well known” in one way or other, subject to the usual shades of meaning that “well known” has in math. For instance the description of spinors by “rotors” – or whatever they call it again in Geometric Algebra circles – is of course a classical fact about Clifford algebra. What is however certainly true is that this classical fact would deserve a much more prominent role in introductory theoretical physics textbooks. It is true that the textbooks like to stick to wildly outdated notation.

Back when I was learning this stuff as a student I was struck that the “rotor” presentation of spinors that Geometric Algebra makes such a fuss about is used all over the place in the discussion of the spinning string, without people even losing a single word on how great this is. I once made a note about that here.

Posted by: Urs Schreiber on February 1, 2011 11:30 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

Urs quoted:

you understand the difference between a mathematical concept and its representation by symbols. Many mathematicians never learn that.

As I’ve probably mentioned before, back in the ’30s, Rainich took pains to point out that a vector was a thing in itself, not its representation in coordinates.

And if many mathematicians never learn that, they are unlikely to enlighten their students.

Posted by: jim stasheff on February 1, 2011 2:58 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Thanks for the long quote of Hestenes, Urs!

What’s really sad is that many of his discoveries about Pauli matrices — described in the ‘little technical bit’ you omitted — were known to Hamilton in 1853, though formulated directly in the language of quaternions instead $2 \times 2$ complex matrices.

I really wonder, now, what Clifford actually said about these topics. This 1885 quote of his is merely tantalizing:

The geometry of rotors and motors … forms the basis of the whole modern theory of the relative rest (Static) and the relative motion (Kinematic and Kinetic) of invariable systems.

Like Hamilton, he seems to have been curiously ahead of his time. I guess you’ve seen this quote from his 1876 On the space-theory of matter:

Riemann has shewn that as there are different kinds of lines and surfaces, so there are different kinds of space of three dimensions; and that we can only find out by experience to which of these kinds the space in which we live belongs. In particular, the axioms of plane geometry are true within the limits of experiment on the surface of a sheet of paper, and yet we know that the sheet is really covered with a number of small ridges and furrows, upon which (the total curvature not being zero) these axioms are not true. Similarly, he says, although the axioms of solid geometry are true within the limits of experiment for finite portions of our space, yet we have no reason to conclude that they are true for very small portions; and if any help can be got thereby for the explanation of physical phenomena, we may have reason to conclude that they are not true for very small portions of space.

I wish here to indicate a manner in which these speculations may be applied to the investigation of physical phenomena. I hold in fact

(1) That small portions of space are in fact of a nature analogous to little hills on a surface which is on the average flat; namely, that the ordinary laws of geometry are not valid in them.

(2) That this property of being curved or distorted is continually being passed on from one portion of space to another after the manner of a wave.

(3) That this variation of the curvature of space is what really happens in that phenomenon which we call the motion of matter, whether ponderable or etherial.

(4) That in the physical world nothing else takes place but this variation, subject (possibly) to the law of continuity.

It’s a bit sad that Hestenes doesn’t mention Elie Cartan’s The Theory of Spinors, which came out in 1931. It seems we’re doomed to keep rediscovering things… or at least we were, before the nLab was invented.

Posted by: John Baez on February 2, 2011 8:05 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

As Urs points out, many physicists know this already. See for example

http://arxiv.org/abs/1010.2979

Posted by: Kea on January 31, 2011 7:11 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Most of my story today is a review of stuff that can be found spread thinly throughout the literature. The problem is that there’s not enough overlap between people who think about time reversal and antiparticles, people who think about normed division algebras, people who think about unitary group representations and Dyson’s Threefold Way, and people who think about symmetric monoidal categories with duals. So, different pieces of the picture are available in different places… but I think all mathematicians and physicists should know all this stuff.

Thanks for the reference to Kauffman’s quaternion belt trick. I’d seen that in his book Knots and Physics. Note that he’s using the belt trick to show how the elements $\pm 1, \pm i, \pm j , \pm k$ live in the double cover of $SO(3)$ , namely the unit quaternions $SU(2)$ . Some aspects of this go back to Dirac’s original ‘belt trick’ for showing why we need a double cover of $SO(3)$ .

Above, I’m using the belt trick to do something quite different! I’m using it to sketch a picture proof that any irreducible unitary representation with an invariant orthogonal (resp. symplectic) structure has an invariant antiunitary operator $J$ with $J^2 = 1$ (resp. $J^2 = -1$ ). I did this more generally and rigorously back in HDA2, but without actually drawing the pictures.

And then, I’m pointing out that $J$ is related to time reversal.

So, Kauffman and I are doing different things… but they’re related, especially when we look at the spin-1/2 representation of $SU(2)$ . And my puzzle, stated in my blog entry, involves understanding the relation!

The relation between the belt trick and time reversal is implicit, but not fully brought out, in Feynman’s lecture here:

• Richard P. Feynman, The reason for antiparticles, in Elementary Particles and the Laws of Physics: the 1986 Dirac Memorial Lectures, Cambridge U. Press, Cambridge, 1987.

Feynman was focused on using the belt trick to give a heuristic proof of the spin-statistics theorem. That’s related to time reversal, since his proof uses antiparticles, and the spin-statistics theorem is related to the CPT theorem. But this whole network of ideas could use a lot of clarification.

Posted by: John Baez on February 1, 2011 3:09 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

There are many other physical representations in our
ordinary space.

The spinor spanner:

E. D. Bolker, The spinor spanner. The American Mathematical Monthly, 80(9):977 - 984, Nov 1973.

and the fact that the fundamental group of SO(3) is Z/2 can be illustrated (literally) by the contortion with a water glass rotating wrist and arm through 720 degrees (I once saw a series of still illustrating this, perhaps in Scientific American).

And most recently, using your shoulder muscles as a ‘belt’:

J. Korbas: An arm trick for unarmed performers, The Mathematical Intelligencer 31, No. 2 (2009), 5-6, DOI:10.1007/s00283-008-9021-y.

Or those who don’t have full access to Springerlink can (probably quite slowly) download a manuscript through this webpage.

Only the true belt trick as I recall requires unbuckling to illustrate it.

For a movie version reference, ask Ronnie Brown

Posted by: jim stasheff on February 1, 2011 3:14 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

The fact that the fundamental group of SO(3) is Z/2 can be illustrated (literally) by the contortion with a water glass rotating wrist and arm through 720 degress

I once saw a series of still illustrating this

Such a series of stills appears on p. 166 of Topology and Geometry by Bredon, in a section titled “Remarks on $\mathbf{SO}(3)$ ” at the end of his chapter on the fundamental group. The discussion in that section doesn’t mention belts, but it does talk about balls embedded in Jello and strings tied to chairs and chandeliers.

Posted by: Mark Meckes on February 2, 2011 2:43 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Heck, who needs pictures of this trick involving a water glass? I do it myself every time I explain this stuff to students! It’s not that hard; just practice a few times with an empty cup first. You turn the cup around twice, first under your arm, then over it.

I usually bring a cup of coffee to class, as if by chance. I then talk about SO(3) and SU(2), and explain how you need to rotate an electron 720° before its wavefunction comes back to where it started… and then I say “But it’s not just electrons! I can even show you how it works with this cup!”

It’s good to stop when you’re halfway done, after rotating the cup by 360°, and dramatically announce that now you are going to rotate the cup another full turn in the same direction. It may not be obvious that this is anatomically possible, so it’s fun to build up the suspense.

Posted by: John Baez on February 3, 2011 5:31 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

You can download a PC program that shows an animation of Dirac’s string/belt/plate/arm trick here:

Antitwister

The program allows you to change many of the parameters on the fly.

Posted by: Mark Hunter on March 5, 2021 2:18 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

I will keep the mathematical error on my blog entry until someone finds it!

Posted by: John Baez on February 1, 2011 2:32 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

I am newly suspicious of the phrase “quaternion-linear”; if $SU(2)$ acts by the left-action I’m used to, then it’ll be quaternion-right-linear as soon as you pick a compatible right-mutiplication by $\mathbb{H}$ , but none will make the action (really) linear. But maybe that’s the conventional usage anyways?

Posted by: some guy on the street on February 1, 2011 7:42 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Some Guy on the Street wrote:

I am newly suspicious of the phrase “quaternion-linear”; if SU(2) acts by the left-action I’m used to, then it’ll be quaternion-right-linear as soon as you pick a compatible right-mutiplication by $\mathbb{H}$ , but none will make the action (really) linear. But maybe that’s the conventional usage anyways?

In a previous post in this series I gave my definition of $\mathbb{K}$ -vector spaces, $\mathbb{K}$ -Hilbert spaces and $\mathbb{K}$ -linear maps when $\mathbb{K}$ is the algebra of real numbers, complex numbers or — the tough one! — quaternions.

So yeah: I defined a $\mathbb{H}$ -vector space to be a right $\mathbb{H}$ -module, and a linear map between these to be a right $\mathbb{H}$ -module morphism.

So, I was not making a mistake here. $SU(2)$ acts on the left on $\mathbb{H}$ , and this action commutes with right multiplications, so it’s ‘quaternion-linear’ by my definitions.

And that’s good, because if I were confused on this point, it would be fatal for everything I’m trying to say.

The mistake I made is much more minor: simply an error of fact, which has no repercussions elsewhere in what I’m saying.

But, I’m glad someone is paying careful attention. It’s very important to avoid pitfalls caused by noncommutativity when generalizing from real and complex Hilbert spaces to the quaternionic case!

By the way: the representation of $SU(2)$ on $\mathbb{H}$ is even ‘quaternion-unitary’! Here I’m using the definition of unitary operators for real, complex and quaternionic Hilbert spaces given in yet another post in this series.

The Three-Fold Way actually says that every self-dual irreducible unitary complex representation of a group comes from either:

• a real-unitary representation on a real Hilbert space, or

• a quaternion-unitary representation on a quaternionic Hilbert space.

Sometimes people say ‘orthogonal’ for ‘real-unitary’, and ‘symplectic’ for ‘quaternion-unitary’ (which can be confusing, since ‘symplectic’ also means something quite different, though closely related).

But I prefer to say ‘unitary’ for all three, so I can state theorems for all 3 associative normed division algebras at once.

Posted by: John Baez on February 2, 2011 4:06 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

I am hooked. Probably spacetime sneaks into the picture because complex quaternions are the rotation algebra for spacetime - see Hestenes. My itty bitty gripe with Hestenes is that Clifford’s combinatorial notation seems more perspicuous, and one can run thru the signatures to get algebra isomorphisms. Being allergic to calculus I got into classifying Clif, but I did want to know whether Clif CAN do what Hestenes wants. It does not and can not explain why space is 3d. But a deeper gripe is How can Dirac Algebra be a unifying language when it has ++++, —-, +— and the other HxH has ++– and -+++ ? That drove me crazy enough to ask what happens if we check the signatures for an anticommuting antiassociative ‘dirac number’ and then you get +— and -+++ for complex octonions. That seems to address why space is three dimensional, and why spacetime is Minkowski. It also dooms the idea that Clifford algebra alone can be what Hestenes is looking for. My other gripe is that telling me that a spinor is a left ideal leaves me with two headaches.
Anyway, since Pauli algebra is just complex quaternions, one ought to ask what would be involved in generalizing the Pauli equation from HxC to complex octonions - the question being - are there not a whole bunch of invariant thingies that live in complex octonions ? And if the alg isomorphisms of HxC are crucial, then all the alg isomorphisms of complex octonions ought to be as well, got from permuting and associating generating units. Also seems to need both the 16 component real frames and complex 8 component frames where unitary stuff can live, to get that unitary rep of rotation etc.
So, I wholeheartedly think geometric algebra ought to be introduced as early as possible, and in an elementary way, there should be a warning label that it does not explain why the universe is the way it is.

Posted by: Joel Rice on February 1, 2011 5:26 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

This is so obvious that it is likely to be wrong: there are no math gods but the math God. Sorry, I dared to say this since I haven’t done my daily prayers to the math God yet!

Posted by: s.h. banihashemi on February 1, 2011 5:56 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

When making jocular religious allusions I prefer them to be polytheistic, because people are less likely to take them seriously. There’s something overly serious about monotheism, which drives men mad.

Not that polytheism is perfect, of course! But if I had to choose between 0,1,2,3,4,… gods, the only number I’d make sure to avoid is 1. The main problem with atheism is that 0 is so close to 1.

Here in Singapore, and also in Hong Kong and Shanghai and Beijing and Chengdu and Hanoi and Hue, I’ve visited lots of temples full of statues of gods. These temples can be Buddhist or Taoist or Confucian, but a lot of the same gods tend to show up regardless. Gods aren’t so fussy about who worships them, over here! People give ‘em offerings of fruit, coca-cola, six-packs of beer, packs of instant noodles, etcetera. And there are so many gods that I can’t keep track of them all!

Around here, it’s perfectly possible for a historical person to become a god after a few centuries. I think that’s good. If you’re gonna have gods, everyone should have a shot at being one.

But, you have to work your way up the ranks. Here, for example, is Guan Yu, with a few oranges and apples:

You can tell he’s a cool dude — he’s popular with martial artists. He started out as a general; four decades after his death he became a marquis; centuries later he became a prince, and then an emperor. And if you read the Wikipedia article, you’ll see a rather offhand remark that he’s now worshipped by Chinese businessmen in Shanxi, Hong Kong, Macau and Southeast Asia as an “alternative wealth god”. That is, alternative to the main wealth god, Cai Shen.

Alas, I’ve never seen any math gods. But in Göttingen I saw lots of little notes placed on Gauss’ grave, from students wanting help with math tests! So he’s well on his way. If China takes over Germany, I bet they’ll build a temple to him someday.

By the way — Hoppy New Year! Tomorrow the Year of the Rabbit begins, and stores here are promising it’ll be a year of abundance.

Posted by: John Baez on February 2, 2011 4:55 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

Gods may be indefinite, but mathematics must be plural.

Posted by: A Marsh on April 17, 2011 7:32 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Out of curiosity: about the zero representation on the space $\{0\}$ , is it real, quaternionic, or complex?

(I don’t think this is the unidentified mistake; anyway, I’ve heard of controversy on whether $0$ is finite)

Posted by: some guy on the street on February 1, 2011 7:33 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Nice question!

I definitely would like every irreducible unitary group representation to be either real, complex or quaternionic, and not more than one of these. But the 0-dimensional representation is not irreducible, just as 1 isn’t prime, so we’re okay.

The concept of a unitary group representation being real or quaternionic makes sense for representations that aren’t irreducible. So, we can still ask if the zero representation is real or quaternionic, and the answer is both. But this is not shocking: any unitary representation of the form $H \oplus H^*$ has this property.

Posted by: John Baez on February 2, 2011 5:10 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

Did you see this?
—
Unified Theory of Ideals
Author: Cohl Furey
(Submitted on 8 Feb 2010 (v1), last revised 10 Mar 2010 (this version, v3))

Abstract: Unified field theories try to merge the gauge groups of the Standard Model into a single group. Here we lay out something different. We give evidence that the Standard Model can be reformulated simply in terms of numbers in the algebra RxCxHxO, as with the earlier work of Dixon [1]. Gauge bosons and the fermions they act on are unified together in the same algebra, as are the Lorentz transformations and the objects they act on. The theory aims to unify everything into the algebra RxCxHxO. To set the foundation, we show this to be the case for a single generation of left-handed particles. In writing the theory down, we are not building a vector space structure, and then placing RxCxHxO numbers in as the components. On the contrary, it is the vector spaces which come out of RxCxHxO.

–
I don’t understand enough of all of this, but it looks like being related.

Greetings, Marcus

Posted by: Marcus Verwiebe on February 1, 2011 8:51 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

i thought the problem was 3 generations - the idea seems to get bogged down, or be less than knockdown convincing. Perhaps the Standard Model suggests putting more properties in than Octonions want to deal with. Can one draw a distinction between ‘defining particles’ and ‘accounting for the behavior of particles’ ? If everything goes in then you would need a bigger algebra, which gets non-alternative. Where is the coexistence of particles defined ? Maybe it goes like Octonions define particles, but it takes a clifford algebra to define the relations in spacetime - Dirac being HxQ which are subalgebras of OxC.
Robert Hermann ‘Spinors, Clifford and Cayley Algebras” has lots of good stuff too - last page esp.

Posted by: Joel Rice on February 1, 2011 10:16 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Marcus wrote:

Did you see this?

Yes. Cohl Furey’s work is closely related to Geoffrey Dixon’s ideas. I’ve been friends with Geoffrey for ages; he’s one of the guys who got me interested in octonions in the first place. So, I’ve spent a lot of time thinking about his book:

• Geoffrey Dixon, Division Algebras: Octonions, Quaternions, Complex Numbers, and the Algebraic Design of Physics, Kluwer, 1994.

and I recommend this as a good place to start if you’re interested in understanding how to think of one generation of fermions in the Standard Model as a field valued in $\mathbb{T}^2$ , where the Dixon algebra $\mathbb{T}$ is defined by

$\mathbb{T} = \mathbb{R} \otimes \mathbb{C} \otimes \mathbb{H} \otimes \mathbb{O}$

(The tensor products are taken over the real numbers, so the $\mathbb{R}$ above is unnecessary — but it looks cool.)

The nicest part of this theory is that picking a unit imaginary octonion simultaneously:

• breaks the 10d Lorentz group $Spin(9,1) \cong SL(2,\mathbb{O})$ down to the 4d Lorentz group $Spin(3,1) \cong SL(2,\mathbb{C})$ ,

and

• breaks the automorphism group of the octonions, $G_2$ , down to the subgroup that fixes a unit imaginary octonion, namely $SU(3)$ .

I have pages and pages of notes on Dixon’s work, but I’ve never gotten around to writing a paper on it. I was hoping to write one with John Huerta after we did our review article on grand unified theories. After all, there are strong relations between Dixon’s theory and all the grand unified theories we discussed, since all these GUTs involve groups acting on $\mathbb{T}$ (though not as algebra isomorphisms, just linear transformations). And John is the only student I’ve had who knows enough about the octonions to help me with this stuff!

However, John wound up working on applications of the octonions to superstrings and super-2-branes, and he’s busy finishing his thesis now, and looking for jobs…

If you can’t get ahold of Geoffrey Dixon’s book, you can still get some good information from his website.

He does website design these days, with physics relegated to a hobby, but he’s been trying to get 3 generations into his model in a natural way. Since $3 \times 8 = 24$ , the Leech lattice beckons. But it’s an extraordinarily tough challenge!

Posted by: John Baez on February 2, 2011 4:15 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

I’ve been looking at Dixon’s book off and on since it came out, and have not seen any reviews, but certainly would welcome that, or commentary or philosophical reflections on the general issues raised.

Normally I would be agnostic about extra dimensions - whatever delivers the goods. But I would sacrifice tensoring a la Dixon if it means being able to explain whether space actually is 3d. Also, once one steps outside the bounds of the standard model, it is not clear how much of SM is reliable, and what might be ‘right for the wrong reasons’, and whether there are some new fundamental principles. Sometimes I think mathematicians look at Octonions as a species of physics, and physicists look at octonions as mathematics. According to Murphy’s law, nobody would be responsible for making sense of it.

Posted by: Joel Rice on February 3, 2011 1:00 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

It was my responsibility to make sense of the octonions, and I did.

Whether it actually helps — that’s another question.

Posted by: John Baez on February 3, 2011 8:10 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

It has helped quite a bit, and continues. I hope there is a Tenth Anniversary celebration of its publication. I see from my morning arXiv ritual that you have another on Division Algebras and Quantum Theory. Is this related to an upcoming Scientific American article ?

Posted by: Joel Rice on February 3, 2011 8:50 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Joel wrote:

I hope there is a Tenth Anniversary celebration of its publication.

Ten years! Holy moly! Time flies. It feels like a lot less. When I notice things like this, I feel like I’ve been wading through molasses. I’ll be dead in no time at this rate.

Anyway, I’ll drink a toast to the octonions on May 16th.

I see from my morning arXiv ritual that you have another on Division Algebras and Quantum Theory. Is this related to an upcoming Scientific American article?

No, that arXiv paper (updated version here) is about the Three-Fold Way: my blog posts here are an attempt to expand that paper and make it a bit more fun. The Scientific American article with John Huerta, which should come out in May, will be loosely based on our ‘Division algebras and supersymmetry’ papers.

John will be speaking about the same subject in a much more technical way in Lisbon soon, at the Workshop and School on Higher Gauge Theory, TQFT and Quantum Gravity.

Posted by: John Baez on February 4, 2011 5:44 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

“… real and complex quaternionic quantum mechanics are lurking inside good old, ordinary complex quantum mechanics.” Will the preceding statement soon have dramatic empirical confirmation? Abdus Salam in his Nobel prize lecture mentioned SU(8) as a possible gauge group for grand unification physics. Does SU(8) scream “quaternionic quantum mechanics”? At nks forum applied nks in the posting “Dark matter: why should Rañada and Milgrom win the Nobel prize?” are my suggestions as to why M-theory will soon be empirically validated.

Posted by: David Brown on February 2, 2011 11:44 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

David wrote:

“… real and complex quaternionic quantum mechanics are lurking inside good old, ordinary complex quantum mechanics.” Will the preceding statement soon have dramatic empirical confirmation?

I’m trying to argue that it’s already been confirmed. For example, if we think of electrons as representations of $SU(2)$ , as in nonrelativistic quantum mechanics, they’re quaternionic. And I’m trying to say that it’s not one of real, complex or quaternionic quantum mechanics that’s been confirmed, it’s all three: they fit into a single structure.

Abdus Salam in his Nobel prize lecture mentioned SU(8) as a possible gauge group for grand unification physics. Does SU(8) scream “quaternionic quantum mechanics”?

Not that I can see. It would if the relevant irreps were quaternionic. But to explain why the fermions in nature are really different than the antifermions, the fermions in any GUT need to lie in a complex representation of the gauge group.

This is why $SU(8)$ , $E_6$ or $Spin(10)$ are acceptable gauge groups for a GUT but not, say, $SO(10)$ , $E_7$ , or $E_8$ : for the latter groups, all the unitary irreps are real or quaternionic.

Posted by: John Baez on February 3, 2011 2:58 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

This is awesome! Although the way you drew the zigzag identities makes me slightly queasy, since in the general case where $H$ and $H^\ast$ are different, you have to write them as two separate equations (since $1_H$ and $1_{H^\ast}$ are different).

I feel like this question must have been addressed already, but I don’t remember the answer: to what extent is “being real” or “being quaternionic” a property of a representation, and to what extent is it structure? When you say that a real or quaternionic representation “comes with” an invariant nondegenerate bilinear form, is that form (or equivalently the operator $J$ ) determined by the given irreducible representation on complex Hilbert space, or does it depend on the expression of that representation as a complexification of a real one or the restriction of a quaternionic one?

Posted by: Mike Shulman on February 2, 2011 9:25 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Mike wrote:

the way you drew the zigzag identities makes me slightly queasy…

Good point. When $H \ncong H^*$ the zig-zag equations are two separate equations and the strings need little arrows on them. I was trying to avoid a discussion of these little arrows mean, since we don’t actually need them here. But I’ve changed the figures now, in a way that should lessen your queasiness.

… to what extent is “being real” or “being quaternionic” a property of a representation, and to what extent is it structure?

Good question! For a general unitary representation of a group on a complex Hilbert space, we must think of “being real” (or “being quaternionic”) as a structure. But for an irreducible representation, it’s almost a property, thanks to Schur’s Lemma. In other words, if the structure exists, it’s almost unique — I’ll show you why.

But first, I should add that normal folks often say an irreducible unitary group representation has the property of being real or quaternionic if there exists a real or quaternionic structure. I’ve probably slipped into doing this here and there.

Okay: what’s a real or quaternionic structure, and why is it almost unique if it exists, at least for an irreducible representation?

First, let’s forget the group representation for a minute. We can define a real or quaternionic structure on a complex Hilbert space $H$ in three equivalent ways:

1. A real (resp. quaternionic) structure is a nondegenerate bilinear form $g : H \times H \to \mathbb{C}$ that is symmetric (resp. antisymmetric).

2. A real (resp. quaternionic) structure is an antiunitary operator $J: H \to H$ with $J^2 = 1$ (resp. $J^2 = -1$ ).

The relation between 1. and 2. is this: $g(v,w) = \langle J v , w \rangle .$

The third way explains the names:

3. A real structure is a real Hilbert subspace $Re(H) \subseteq H$ whose complexification is $H$ . A quaternionic structure is a way of extending $H$ to a quaternionic Hilbert space.

The relation between 2. and 3. is this:

If $J^2 = 1$ , we can define $Re(H) = \{ v \in H : J v = v \} .$ If $J^2 = -1$ , we can uniquely extend $H$ to a quaternionic Hilbert space where multiplication by $j$ acts as the operator $J$ .

But now suppose $H$ is an irreducible unitary representation of some group and we demand that our real or quaternionic structure be invariant under this group action! Then our antiunitary operator $J : H \to H$ commutes with the group action. We can reinterpret as as a unitary operator $\tilde{J} : H \to H^*$ using the canonical antiunitary $H \cong H^*$ . And $\tilde J$ must be compatible with the group action, so by Schur’s Lemma it’s unique up to a constant factor. The same is therefore true of $J$ .

But there’s not much freedom to replace the antiunitary $J$ by a constant multiple $\alpha J$ while keeping its property $J^2 = 1$ (or $J^2 = -1$ ).

I’ll let people figure out which $\alpha$ ’s are allowed. These $\alpha$ ’s describe our freedom to change an real or quaternionic structure on an irreducible unitary group representation.

Posted by: John Baez on February 3, 2011 2:50 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

John wrote:

I’ll let people figure out which $\alpha$ ’s are allowed. These $\alpha$ ’s describe our freedom to change an real or quaternionic structure on an irreducible unitary group representation.

As a hint for this puzzle, let me add that:

The freedom to change a real structure is completely visible when we look at all the different real structures on a 1-dimensional complex Hilbert space $H$ . Each real line through the origin gives us a real structure with that line as the real subspace $Re(H) \subseteq H$ .

The freedom to change a quaternionic structure is completely visible when we look at all the different quaternionic structures on a 2-dimensional complex Hilbert space $\mathbb{C}^2$ . If we fix any quaternionic structure on $\mathbb{C}^2$ , so that now we know how to multiply vectors by quaternions, all possible quaternionic structures come from $J$ operators as follows:

$J = \cos(\theta) j + \sin(\theta ) k$

In other words, the quaternionic structure $J$ can be multiplication by any unit imaginary quaternion that’s orthogonal to $i$ . They’re all equally good choices for what counts as ‘multiplication by $j$ ’.

There’s a nice analogy between these two cases which should give away the answer to my puzzle.

Posted by: John Baez on February 3, 2011 5:55 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

There’s a (possibly trivial, possibly not) point about real and quaternionic structures I’ve always meant to try to understand but never got around to. Why does one always start with a complex Hilbert space? Couldn’t we just as well start with a real Hilbert space and in similar ways define complex and quaternionic structures, or start with a quaternionic Hilbert space and define real and complex structures?

Maybe there’s some reason I’m not seeing that this simply wouldn’t work. On the other hand, if it does work, I can imagine several (not mutually exclusive) reasons why things are usually done starting with complex Hilbert spaces:

It’s simpler that way, essentially because $\mathbb{C}$ is in the middle of $\mathbb{R} \subset \mathbb{C} \subset \mathbb{H}$ . Whereas as quaternionic structure on a complex vector space can be described as a single operator $J$ , a quaternionic structure on a real vector space would need two appropriate anticommuting operators.
People are simply most accustomed to working with complex Hilbert spaces.
Operator theory over a complex Hilbert space is more well-behaved, so it makes the most convenient background.
That’s just the way someone wrote it down first.

And maybe there are other good reasons I haven’t thought of.

Posted by: Mark Meckes on February 3, 2011 2:23 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

I forgot to include in point 3 above the closely related point:

3a. Representation theory over $\mathbb{C}$ is more well-behaved.

Posted by: Mark Meckes on February 3, 2011 2:27 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Mark wrote:

Couldn’t we just as well start with a real Hilbert space and in similar ways define complex and quaternionic structures, or start with a quaternionic Hilbert space and define real and complex structures?

Yes we can, and that’s what I’m going to explain next! The last paragraph of this blog entry was a hint:

Next time I want to say more about how real, complex and quaternionic quantum theory fit together. I’ve made it sound like complex quantum theory is the main thing, with the real and quaternionic theories sitting inside it. But that’s just part of the story. To see the whole story, we should use a bit more category theory.

Posted by: John Baez on February 4, 2011 4:05 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

The last paragraph of this blog entry was a hint:

Oh, I should have picked up on that! In that case, I look forward to next time.

Posted by: Mark Meckes on February 4, 2011 3:01 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Very nice, thank you!

I’ll let people figure out which $\alpha$ ’s are allowed.

This seems too simple, but since $J$ is antilinear we have $(\alpha J)^2 = \alpha J(\alpha J) = \alpha \bar{\alpha} J^2 = {|\alpha|}^2 J^2$ . So to maintain the value of $J^2$ we need ${|\alpha|} =1$ . That seems to match your two examples, since each is determined by a single angular parameter.

Posted by: Mike Shulman on February 5, 2011 5:38 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Mike wrote:

… we need $|\alpha| =1$ . That seems to match your two examples, since each is determined by a single angular parameter.

Right! So there’s always exactly a circle’s worth of ways to make an irreducible unitary complex rep either real or quaternionic, if there’s any way at all.

Furthermore, all these ways are isomorphic!

So, while a real or quaternionic structure really is a structure on an irreducible unitary complex rep, it’s a pretty bland structure. The tricky and interesting part of this structure is the property of whether our irrep admits this structure at all. After that it’s all downhill.

Nonetheless it’s great that you got me to clarify this. For example, you got me to notice that the all the fibers of the forgetful functors

$UnitaryIrrep_{\mathbb{R}}(G) \to UnitaryIrrep_{\mathbb{C}}(G)$

$UnitaryIrrep_{\mathbb{H}}(G) \to UnitaryIrrep_{\mathbb{C}}(G)$

are transitive faithful $\mathrm{U}(1)$ -sets. That is, they’re either empty, or $\mathrm{U}(1)$ -torsors.

Posted by: John Baez on February 5, 2011 8:12 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

So, while a real or quaternionic structure really is a structure on an irreducible unitary complex rep, it’s a pretty bland structure. The tricky and interesting part of this structure is the property of whether our irrep admits this structure at all.

Interesting! I wonder if this sort of thing fits into the general theory of stuff, structure, and property somewhere nice? My first thought was that it might be “property-like structure”, but that doesn’t seem right; any two structures on the same object are isomorphic, but the isomorphism isn’t the identity. Is there maybe some 2-category whose 2-cells are “multiplication by a unit complex number” in which we could consider “pseudo morphisms” of this structure?

all the fibers of the forgetful functors… are transitive faithful U(1)-sets.

I don’t suppose there is any relationship between this and the fact that the phase of a single particle is unobservable, and that the gauge group of electromagnetism is U(1)?

Posted by: Mike Shulman on February 5, 2011 6:42 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Mike wrote:

I wonder if this sort of thing fits into the general theory of stuff, structure, and property somewhere nice? My first thought was that it might be “property-like structure”, but that doesn’t seem right; any two structures on the same object are isomorphic, but the isomorphism isn’t the identity.

I had some very similar thoughts, and I seem to have succeeded in transmitted them telepathically without quite coming out and saying them. I was going to say something about ‘property-like structure’ myself — but as you note, that’s not quite right here!

I think there’s some important type of ‘bland structure’ lying between property and full-fledged structure, which we are seeing here. Formally, we could say a functor

$F : C \to D$

forgets bland structure if it is faithful and given any two objects $c, c'$ in the essential inverse image $F^{-1}(d)$ of any object $d \in D$ , we have $c \cong c'$ .

The paradigm of forgetting bland structure is the functor from pointed $G$ -torsors to $G$ -torsors. Here the fibers are all groupoids equivalent to $G$ .

I don’t suppose there is any relationship between this and the fact that the phase of a single particle is unobservable, and that the gauge group of electromagnetism is U(1)?

This $\mathrm{U}(1)$ business probably has a bit more to do with the unobservable phases in physics than the electromagnetic gauge group. The relation between the former and latter confused people for a long time.

At present, it appears to be something of a ‘coincidence’ that the gauge group of electromagnetism is the same as the group of phases. In other words, we shouldn’t identify these two copies of $\mathrm{U}(1)$ , because they act differently on the Hilbert spaces of physical systems. The phase $\mathrm{U}(1)$ acts in a completely natural way on all Hilbert spaces, while the electromagnetic $\mathrm{U}(1)$ acts differently on different particles, depending on their electric charge. Indeed this is the modern definition of charge.

When we completely understand physics we may have more to say about this, but for now let me ignore the electromagnetic $\mathrm{U}(1)$ and focus on the phase $\mathrm{U}(1)$ .

What do I mean by saying “The phase $\mathrm{U}(1)$ acts in a completely natural way on all Hilbert spaces”?

I really mean that $\mathrm{U}(1)$ acts as automorphisms of the identity functor $Hilb_{\mathbb{C}}$ . In other words, a phase $\alpha \in \mathrm{U}(1)$ acts as a unitary operator on every Hilbert space in the world:

$\alpha : H \to H$

and since these phases commute with all other linear transformations, we get a natural isomorphism from the identity functor $1_{Hilb_\mathbb{C}}$ to itself.

Indeed, I’m pretty sure

$Aut(1_{Hilb_\mathbb{C}}) = \mathbb{C}^\times$

but only $\mathrm{U}(1) \subset \mathbb{C}^\times$ acts as unitary natural isomorphisms (a concept that makes sense in any dagger-category).

This leads us to the following:

Puzzle: what are the unitary natural automorphisms of the identity functor on $Hilb_{\mathbb{H}}$ and $Hilb_{\mathbb{R}}$ ?

There’s more to say — you’re making me think of all sorts of interesting things — but I’ll stop here for now.

Posted by: John Baez on February 6, 2011 3:32 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

we could say a functor $F\colon C\to D$ forgets bland structure if it is faithful and given any two objects $c,c'$ in the essential inverse image $F^{-1}(d)$ of any object $d\in D$ , we have $c\cong c'$ .

Interesting. Yes, perhaps that’s a reasonable thing to say. Coincidentally I heard about some other kind of “bland structure” in a topology seminar today: any two symplectic forms on a closed manifold $M$ that represent the same cohomology class in $H^2(M;\mathbb{R})$ are diffeomorphic (i.e. there is a self-diffeomorphism of $M$ taking one to the other).

I don’t suppose one could ask for any “naturality” or “functoriality” of the isomorphisms $c\cong c'$ . I’m thinking about the “representable” way of describing the other types of forgetful functors in terms of a 2-category; can we say that $F\colon C\to D$ forgets (at most) bland structure if all the functors $Cat(X,C) \to Cat(X,D)$ have some property?

At present, it appears to be something of a ‘coincidence’ that the gauge group of electromagnetism is the same as the group of phases. In other words, we shouldn’t identify these two copies of U(1),

That’s good to know. Now I’ll try to remember it. It’s always tricky with small groups like U(1) and small numbers like 2 and 3 to tell whether two copies of them are “the same” or “different.” (-:

what are the unitary natural automorphisms of the identity functor on $Hilb_{\mathbb{H}}$ and $Hilb_{\mathbb{R}}$ ?

Well, the real case isn’t too hard, is it? I feel sure the automorphisms of $1_{Hilb_{\mathbb{R}}}$ must also be $\mathbb{R}^\times$ , and that that should be true over any (commutative) field. Of those, only $1$ and $-1$ will act unitarily, so the unitary automorphisms of $1_{Hilb_{\mathbb{R}}}$ should be $\mathbb{Z}/2$ .

The quaternionic case seems more problematic because of noncommutativity. For instance, if we regard $\mathbb{H}$ as a 1-dimensional (right) $\mathbb{H}$ -Hilbert space, then $\mathbb{H}$ acts on this space by left multiplication, so any automorphism of $1_{Hilb_{\mathbb{H}}}$ is going to have to commute with all quaternions, and there aren’t even that many quaternions that do that! In fact, it seems that only the real numbers commute with all quaternions, so that again we should have $Aut(1_{Hilb_{\mathbb{H}}}) = \mathbb{R}^\times$ with only $\{\pm 1\}$ being unitary. Is that right?

What does this mean for real and quaternionic quantum mechanics? Does the “unobservable phase” get reduced to an “unobservable sign”?

Posted by: Mike Shulman on February 9, 2011 12:22 AM | Permalink | PGP Sig | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Mike wrote:

… so that again we should have $Aut(1_{Hilb_{\mathbb{H}}}) = \mathbb{R}^\times$ with only $\{\pm 1\}$ being unitary. Is that right?

I don’t think so! I’m getting a lot more natural automorphisms of the identity functor on $Hilb_{\mathbb{H}}$ . This “useful clue” should help — if I’m not seriously confused.

Once we agree on this, it should be easy and fun to work out the whole 2-group of symmetries of $\Hilb_{\mathbb{R}}$ , $\Hilb_{\mathbb{C}}$ and $\Hilb_{\mathbb{H}}$ .

Posted by: John Baez on February 9, 2011 7:47 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Isn’t this solved by a slight variant of Tannaka duality for algebra modules?

Posted by: Urs Schreiber on February 9, 2011 8:23 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

I guess so. But it’s really easy to show directly, like this.

Posted by: John Baez on February 10, 2011 4:54 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Isn’t this solved by a slight variant of Tannaka duality for algebra modules?

I guess so. But it’s really easy to show directly, like this.

Full Tannaka duality is also really easy to show directly! Like this.

Posted by: Urs Schreiber on February 10, 2011 7:35 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Okay, good. I guess all this stuff is pretty simple.

Posted by: John Baez on February 10, 2011 7:47 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

I guess all this stuff is pretty simple.

In the famous words of Peter Freyd:

the purpose of categorical algebra is to show that which is trivial is trivially trivial.

I believe therefore the terms “Yoneda lemma” and “Tannaka duality” should not be thought of as “scary” (I can’t think of any concept in math that should be thought of as scary – physics is scary, climate science is scary because much less is understood, math is all nice and clean and maximally secure):

they save us from rediriving the trivially trivial over and over again and recognize at a glance when we have a trivial situation.

Posted by: Urs Schreiber on February 10, 2011 3:23 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Well one of us is confused, but it could just as easily be me. Here’s what I was thinking in more detail: suppose $\alpha$ is an endo-natural-transformation of $1_{Hilb_{\mathbb{H}}}$ . Now $\mathbb{H} \in Hilb_{\mathbb{H}}$ , so $\alpha$ has a component which is an $\mathbb{H}$ -linear map $f \coloneqq \alpha_{\mathbb{H}} \colon \mathbb{H} \to \mathbb{H}$ . Since “ $\mathbb{H}$ -linear” means right $\mathbb{H}$ -linear, for any $x \in \mathbb{H}$ we have $f(x) = f(1) \cdot x$ , so $f$ is necessarily left multiplication by the quaternion $f(1)$ .

But since $\alpha$ is a natural transformation from $1_{Hilb_{\mathbb{H}}}$ to itself, for any $\mathbb{H}$ -linear $g\colon \mathbb{H} \to \mathbb{H}$ we have $1_{Hilb_{\mathbb{H}}}(g) \circ \alpha_{\mathbb{H}} = \alpha_{\mathbb{H}} \circ 1_{Hilb_{\mathbb{H}}}(g)$ which is to say $f\circ g = g\circ f$ . Now as you said in the useful clue, for any quaternion $y$ we have an $\mathbb{H}$ -linear map given by left multiplication by $y$ , $g_y(x) = y\cdot x$ . So $f\circ g = g\circ f$ for all $g\colon \mathbb{H} \to \mathbb{H}$ means $f(1) \cdot y = y\cdot f(1)$ for all quaternions $y$ , which I think means that $f(1)$ must be real.

I thought this was the reason we call endomorphisms of an identity functor the center. Am I going wrong somewhere?

Urs wrote:

Isn’t this solved by a slight variant of Tannaka duality for algebra modules?

I don’t know; is there a version of Tannaka duality when the ground field is noncommutative? We can’t use ordinary enriched category theory to state it, since $\mathbb{H} Mod$ is not a monoidal category.

Posted by: Mike Shulman on February 9, 2011 2:35 PM | Permalink | PGP Sig | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Mike wrote:

Am I going wrong somewhere?

No, you’re right. I was being silly.

For the sake of any nonexperts listening in, here’s the whole argument, stripped of scary names like ‘Tannaka-Krein’ and ‘Yoneda’.

Suppose $\mathbb{K}$ is a normed division algebra and $\alpha$ is a natural transformation from the identity functor $1_{Hilb_{\mathbb{K}}}$ to itself. We want to show there’s an element $c$ of the center of $\mathbb{K}$ such that for every $\mathbb{K}$ -Hilbert space $H$ , $\alpha_H : H \to H$ is just multiplication by $c$ .

Here’s how. Since $\mathbb{K}$ is a $\mathbb{K}$ -Hilbert space, let’s start there: $\alpha_{\mathbb{K}} : \mathbb{K} \to \mathbb{K}$ must be left multiplication by some $c \in \mathbb{K}$ . For $\alpha$ to be be natural this needs to commute with all linear transformations $f : \mathbb{K} \to \mathbb{K}$ , which are also given by left multiplication. So, $c$ must lie in the center of $\mathbb{K}$ .

What about $\alpha_H$ for some other $\mathbb{K}$ -Hilbert space $H$ ? For any vector in $H$ there’s a linear map $f: \mathbb{K} \to H$ hitting that vector, so by naturality $\alpha_H : H \to H$ must multiply that vector by $c$ , too. (Draw the commutative square in the definition of natural transformation.) So, we’re done.

If we also want $\alpha_H$ to be unitary we need $|c| = 1$ .

So, the group of unitary natural automorphisms of $1_{Hilb_{\mathbb{K}}}$ is $\mathbb{Z}_2, \mathrm{U}(1)$ and $\mathbb{Z}_2$ for $\mathbb{K} = \mathbb{R}, \mathbb{C}$ and $\mathbb{H}$ , respectively.

Posted by: John Baez on February 10, 2011 12:28 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Isn’t this solved by a slight variant of Tannaka duality for algebra modules?

I don’t know; is there a version of Tannaka duality when the ground field is noncommutative? We can’t use ordinary enriched category theory to state it, since ℍMod is not a monoidal category.

Therefore “slight variant”: use ordinary Tannaka duality to deduce that the endomorphisms of $Vect_{\mathbb{H}} \to Vect$ are $\mathbb{H}$ , and then find among these those that are also $\mathbb{H}$ -linear (and preserve the Hilbert space stucture, if desired) and hence factor as an endomorphism of $Id_{Vect_{\mathbb{H}}}$ followed by whiskering with the forgetful functor.

That comes down to essentially the argument you just spelled out, only that it adds the observation that your analysis of the component on $\mathbb{H}$ is not just necessary but also sufficient.

(Of course you can and probably do regard that as obvious. Which corresponds to the fact that the Tannaka duality needed here is the “plain” one, the one that is just pure Yoneda, hence “essentially trivial”).

Posted by: Urs Schreiber on February 9, 2011 4:49 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Ah, I see what you mean. Yes, that makes sense.

Posted by: Mike Shulman on February 9, 2011 7:25 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

At present, it appears to be something of a ‘coincidence’ that the gauge group of electromagnetism is the same as the group of phases. In other words, we shouldn’t identify these two copies of U(1),

That’s good to know. Now I’ll try to remember it. It’s always tricky with small groups like U(1) and small numbers like 2 and 3 to tell whether two copies of them are “the same” or “different.” (-:

I’d have two comments:

First, one immediate way to see that the quantum mechanical phase is not identified with the gauge group of electromagnetism is that there are plenty of quantum mechanical systems, all of them with complex phases, but only few of them “containing electromagnetism” in the first place.

Of course you could imagine that of all these theoretically possible systems only some will appear as aspects of our observed world and that for all those the fundamrental reason that they contain complex phases is by some mysterious reason the fact that we also observe electromagnetism.

For instance you could maybe say: Chern-Simons theory is a quantum system with complex phases, but which does not “contain electromagnetism” in any way. But maybe one of the several hypothesis out there is correct, which assert that Chern-Simons theory is secretly one building block of the fundamental theory that describes the world, and by some mysterious mechanism the fact that it has complex phases may then be understood as a consequence that this fundamental theory also contains electromagnetism.

That however seems very implausible, even though maybe logically it might not have been ruled out.

So in this sense I would say it is quite clear that quantum phases are not electromagnetic phases.

But now I am going to argue the opposite, after generalizing the perspective on the question.

Go through the geometric quantization of the single particle (say the single electron) subject to background gravity and background electromagnetism and trace back the origin of the complexity of the Hilbert space that you end up with. You see that it comes from the fact that this Hilbert space is the $L^2$ -space of sections of a complex line bundle on target space, which is complex because it is associated to the $U(1)$ -principal bundle whose connection encodes the background electromagnetic field.

To say this in other words: the charged particle is a 0+1 dimensional sigma-model QFT defined by the fact that on its configuration space is a circle bundle with connection that encodes the background gauge field. Its space of states is a complex vector space because it is the space of sections of the line bundle associated to this circle bundle.

Stated this way, we see that this is the same kind of mechanism that makes for instance also Chern-Simons theory have complex phases:

CS theory is a (2+1)-dimensional sigma model QFT with target something like the space $\mathbf{B}G$ and background gauge field a circle 3-bundle with connection on this space: (a “ $U(1)$ -bundle 2-gerbe”). Its space of states is a space of (certain) sections of a bundle associated to the transgression of this circle 3-bundle to the surface space of target space, which is again a circle 1-bundle with connection.

This circle 1-bundle with connection of Chern-Simons theory in codimension 1 certainly “is not electromagnetism”, but it has mathematically precisely the same form as the background field of the electrically charged particle.

So in a way the origin of the complexity of the phases both of the electrically charged particle as well as for instance of Chern-Simons theory is that in codimension 1 their background fields are in both cases circle bundles with connection.

The analogous statement is true for a large class of quantum systems. I feel that this is the reason for complex phases in quantum physics.

Posted by: Urs Schreiber on February 9, 2011 6:51 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

My prostrated offerings to John Oppenheimer, to Freeman Dyson, to Richard Feynman, to Andre Weil, to Goro Shimura, to S. S. Chern, to Andrew Weil, to Richard Taylor, to Alain Connes, to Mahmood Hesabi, to Reza Mansouri, to Mohammad Reza Mokhtarzadeh, to Shahin Sheikh-Jabbari, to Yasaman Farzan and last but not least to John Baez. However, I am still looking for how to pray most devotedly to the math God.

Posted by: s. h. banihashemi on February 3, 2011 8:48 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Do good (math)!

Posted by: Mike Stay on February 3, 2011 10:33 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

A version of my paper on this stuff is now available on the arXiv. It does not yet contain the corrections suggested by people above. There’s a more up-to-date version here on my website:

John Baez, Division algebras in quantum theory.

Here’s a comment I got in email from Fred Lunnon. He also posted it to the geometric algebra mailing list, and he suggested that I could respond to it here. I’d be delighted if other people here tackle any questions that they they know the answers to, because there are a lot! I’ll answer the rest in a while.

Remarks on ArXiV paper and overlapping thread on The n-Category Cafe:

John C. Baez “Division Algebras and Quantum Theory” arXiv and n-Category Café: The Three-Fold Way (Part 4).

I found this stuff on the whole readable, entertaining, provocative, and at pains to explain things fully; though there were some exceptions.

(O) Misprints.

p13 Thm. 4 — for “cones” read “cone”. p23 Sec. 6 — for “deeeper” read “deeper”

(A)

In a number of places from section 3 onwards, the term “symplectic” appears, denoting self-adjoint representations over the quaternions. Is there any connection with a symplectic group? If so what, and which one — Sp(n) or Sp(2n, $\mathbb{R}$ ) or Sp(2n, $\mathbb{C}$ ) or …, in the notation of Wikipedia: Symplectic group. If not, why not just call them “self-adjoint” or (as suggested there) “unitary”?

(B)

A couple of queries from early in section 1:

A theorem which perhaps I should know but do not: why must any unitary representation of a compact group be finite-dimensional?

And what is meant by “underlying”, appearing in several places, as in: “underlying complex representation of a representation of G on some quaternionic Hilbert space”?

(C)

Section 4 pp. 17–18 explains in detail why the “real” versus “symplectic” classification is meaningful, but on this showing “complex” seems to be merely a ragbag of leftovers: why should it count as a class on the same footing as the others?

(D)

Section 5 p. 19 mentions a connection between (matrix) representations and particle spin which is presumably familiar to physicists, but leaves me baffled. Exactly what aspect of the physical behaviour of an electron (say) is modelled by some spin-1/2 representation of SU(2), but not already modelled satisfactorily by quaternions [or SU(2) itself]?

(E)

It’s 40 years since I first encountered category theory — and despite numerous attempts, some inspired by Baez’s evident enthusiasm, I still have no idea what it’s actually for. Can anybody explain to me, for instance, how its deployment enhances this particular paper?

(F)

I’m prepared to put up with a good deal of hand-waving from somebody who brings it off with sufficient conviction; however a particularly cryptic example early in section 1 (again!) quickly pulled me up short: “Because we can think of a pair of complex numbers as a quaternion …”

Just in case anybody else spends as long as I did, scratching my head over this before eventually concluding it must actually be something I already knew, here’s a summary of what I tentatively think it is about.

Notation:

$\mathbb{R}$ denotes real numbers $a, b, c, d,$ …;

$\mathbb{C}$ denotes complex numbers $u = a + b I$ , $v = c + d I$ , …;

$\mathbb{H}$ denotes quaternions $a + b i + c j + d k$ ;

$\mathbb{K}(n)$ denotes $n \times n$ matrices over $\mathbb{K}$ , where $\mathbb{K}$ denotes $\mathbb{R}$ or $\mathbb{C}$ or $\mathbb{H}$ ;

$SO(3)$ denotes $3 \times 3$ orthonormal matrices over $\mathbb{R}$ (inverse = transpose);

$SU(2)$ denotes $2 \times 2$ unitary matrices over $\mathbb{C}$ (inverse = adjoint == conjugate transpose); $S(2)$ denotes (symmetries of) ordinary sphere;

$Spin(3,0)$ denotes quotient by positive reals of even versor group in Clifford algebra $Cl(3,0)$ , or equivalently in $Cl(0,3)$ ;

$\mathbb{H} \otimes \mathbb{C}$ (for example) denotes the tensor product, in which each component in $\mathbb{R}$ of a member of $\mathbb{H}$ is replaced by a member of $\mathbb{C}$ — “complexification” — or equivalently vice-versa;

$2 \mathbb{C}$ or $\mathbb{C} \oplus \mathbb{C}$ (for example) denotes the double complex numbers, with sum and product defined component-wise.

Firstly, what the quotation above does NOT justify: complex-complex numbers are not isomorphic to quaternions, not even as (multiplicative) groups: in fact, as (multiplicative and additive) rings,

$\mathbb{C} \otimes \mathbb{C} \cong 2 \mathbb{C}$

under the bijection $(1 \otimes 1)(u + v)/2 + (I \otimes I)(u - v)/2 \mapsto u \oplus v$

with $u,v \in \mathbb{C}$ and their multiplication is commutative, in contrast to $\mathbb{H}$ .

Instead, the correspondence maps $u = a + b I \in \C$ to $a + b i \in H$ , and maps the pair $[u, v]$ to $u + v j$ ; finally

$[a + b I , c + d I] \mapsto a + b i + c j + d k .$

While this wheeze is a useful mnemonic, it’s hard to see what significance it could possibly have in the present context!

Next a classic correspondence between quaternions and unitary matrices, and a few more things:

$\mathbb{H}$ unit $\cong$ $Spin(3,0) \cong$ S(2) $\cong$ SO(3) double-cover $\cong$ SU(2)

given by

$a + b i + c j + d k with a^2 + b^2 + c^2 + d^2 = 1$

$\mapsto$

$[[a - b I, c I + d], [c I - d, a + b I ]] \in SU(2)$

an isomorphism of rings and Lie-groups, and a diffeomorphism.

More generally, this correspondence complexifies immediately to the ring isomorphism

$\mathbb{H} \otimes \mathbb{C} \cong Cl(3,0) \cong |C(2)$

via substitution of $u,v,...$ in $\mathbb{C}$ for $a,b,... \in \mathbb{R}$ .

See

Wikipedia, Representation theory of SU(2)

Thomas MacFarlane, The identity of SU(2) and $S^3$ .

The only thing I don’t understand above is:

$S(2)$ denotes (symmetries of) ordinary sphere

because the sphere is different than the group of symmetries of the sphere. The sphere is usually denoted $S^2$ , and the group of symmetries of the sphere is either $SO(3)$ if one disallows reflections, $O(3)$ if one includes them, or perhaps the double covers of these groups if one really wants, namely $Spin(3) \cong SU(2)$ and $Pin(3) \cong SU(2) \times \mathbb{Z}/2$ .

Luckily, the notation $S(2)$ is only used once above, and there it’s used to mean the double cover of $SO(3)$ , which I’d call $Spin(3)$ , or perhaps $Spin(3,0)$ if I were in a certain mood.

Posted by: John Baez on February 4, 2011 4:59 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

I’ll answer a couple questions now:

A theorem which perhaps I should know but do not: why must any unitary representation of a compact group be finite-dimensional?

That’s not true: all compact groups have lots of infinite-dimensional unitary representations. The theorem is this: every irreducible strongly continuous unitary representation of a compact group is finite-dimensional. This is a corollary of the Peter-Weyl theorem, which is the basic theorem on representations of compact groups. The proof takes some work!

By the way, the ‘strongly continuous’ clause may look technical, but we need it, since without some clause involving topology we can’t really use the compactness of our group, which is a topological condition.

Puzzle, for anyone who wants it: find an infinite-dimensional irreducible unitary representation of the compact group $SU(2)$ .

And what is meant by “underlying”, appearing in several places, as in: “underlying complex representation of a representation of G on some quaternionic Hilbert space”?

The concept of “underlying” is extremely general: one may speak of the underlying set of an abelian group (since an abelian group is a set with some extra structure), the underlying abelian group of a ring (since a ring is an abelian group with some extra structure), etcetera. But I won’t try to explain this concept in its full generality, since that involves category theory. I’ll stick to answering the question in the case you’re wondering about!

The underlying complex Hilbert space of a quaternionic Hilbert space $H$ is defined as follows. We take $H$ and make it into a complex vector space by thinking of $\mathbb{C}$ as a subalgebra of $\mathbb{H}$ in the usual way, and restricting our ability to multiply elements of $H$ by scalars to those scalars in $\mathbb{C}$ . Then, $H$ becomes a complex Hilbert space where we define a new complex-valued inner product by taking the ‘complex part’ of the original quaternionic inner product:

$Co(a + b i + c j + d k) = a + b i$

where, unlike Fred Lunnon, I’m lazily using $i$ both to mean the quaternion and the complex number by that name.

Posted by: John Baez on February 5, 2011 3:13 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

We take $H$ and make it into a complex vector space by thinking of $\mathbb{C}$ as a subalgebra of $\mathbb{H}$ in the usual way

Isn’t there something fishy about that too, though? I mean, sure, the “usual” embedding of $\mathbb{C}$ into $\mathbb{H}$ takes $a+b i$ to $a+b i$ , but abstractly there’s no reason why the quaternionic $i$ is any more like the complex $i$ than is the quaternionic $j$ , or the quaternionic $k$ , or for that matter any unit imaginary quaternion. So don’t we have a whole $S^2$ worth of embeddings of $\mathbb{C}$ into $\mathbb{H}$ ?

Posted by: Mike Shulman on February 5, 2011 5:48 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Mike wrote:

Isn’t there something fishy about that too, though?

Well, ‘fishy’ is a moral judgement, and in this particular case I think it’s extremely important not to throw the baby out with the bathwater just because the water smells a bit fishy — so I won’t answer ‘yes’ or ‘no’ to this question.

I mean, sure, the “usual” embedding of $\mathbb{C}$ into $\mathbb{H}$ takes $a+b i$ to $a+b i$ , but abstractly there’s no reason why the quaternionic $i$ is any more like the complex $i$ than is the quaternionic $j$ , or the quaternionic $k$ , or for that matter any unit imaginary quaternion. So don’t we have a whole $S^2$ worth of embeddings of $\mathbb{C}$ into $\mathbb{H}$ ?

Now that’s a factual question, so I can answer it — and the answer is yes!

There’s not just one inclusion of $\mathbb{C}$ into $\mathbb{H}$ ; there’s a whole sphere’s worth of them. So you may rightly object to me talking about ‘the’ forgetful functor from $\mathbb{H}$ -Hilbert spaces to $\mathbb{C}$ -Hilbert spaces, or ‘the’ underlying complex Hilbert space of a quaternionic Hilbert space. There is, in fact, a whole sphere’s worth of them!

However, it’s a fairly harmless sin as long as you keep your wits about you. There’s a lot of inclusions of $\mathbb{C}$ into $\mathbb{H}$ … but they’re all related by symmetries of $\mathbb{H}$ ! The automorphism group of $\mathbb{H}$ is $SO(3)$ : this acts as rotations on the space of imaginary quaternions

$\{ a i + b j + c k : a,b,c \in \mathbb{R} \} .$

So, this group acts transitively on the $S^2$ which parametrizes inclusions of $\mathbb{C}$ into $\mathbb{H}$ .

So, while I may use my favorite inclusion $\mathbb{C} \to \mathbb{H}$ to define my forgetful functor

$F : Hilb_{\mathbb{H}} \to Hilb_{\mathbb{C}}$

and you may use your favorite inclusion to define yours, say

$F\,' : Hilb_{\mathbb{H}} \to Hilb_{\mathbb{C}}$

there’s an automorphism of categories

$\alpha : Hilb_{\mathbb{H}} \to Hilb_{\mathbb{H}}$

coming from an automorphism of $\mathbb{H}$ , which relates your forgetful functor to mine:

$F\,' = F \circ \alpha$

This is why for many applications we can pick any one inclusion $\mathbb{C} \to \mathbb{H}$ , call it the ‘standard’ one, and use that, without worrying that another choice would give significantly different results.

Posted by: John Baez on February 5, 2011 8:40 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Okay, makes sense. I observe that while SO(3) acts transitively on $S^2$ , the action is not free, and the stabilizer group of a point is $S^1$ —which appears to be the same $S^1$ that popped up over here. That is, we choose a unit quaternion (a point of $S^2$ ) in order to embed $\mathbb{C}$ in $\mathbb{H}$ , and then the $S^1$ that’s left of SO(3) lets us rotate through the unit quaternions orthogonal to our chosen one. Is there some way to say that formally?

Posted by: Mike Shulman on February 5, 2011 7:01 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Interesting! Yes, there must be some beautiful way to formalize what you’re saying: the fibration

$S^1 \to SO(3) \to S^2$

is showing up when we study the fibers of the functor

$UnitaryIrrep_\mathbb{H}(G) \to UnitaryIrrep_\mathbb{C}(G)$

because $SO(3) = Aut(\mathbb{H})$ acts on $UnitaryIrrep_\mathbb{H}(G)$ , and the stabilizer of the chosen copy of $\mathbb{C}$ in $\mathbb{H}$ is $S^1$ .

But that’s as far as I’ll carry the ball right now… I have to get some other work done!

Posted by: John Baez on February 6, 2011 3:41 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Yes, there must be some beautiful way to formalize what you’re saying…

Well, here’s the best I’ve been able to come up with, which is just making a bit more precise what you just said. SO(3) acts on the category $UnitaryIrrep_{\mathbb{H}}(G)$ , so it also acts on the functor category $CAT(UnitaryIrrep_{\mathbb{H}}(G), UnitaryIrrep_{\mathbb{C}}(G))$ . The “forgetful functors” corresponding to embeddings of $\mathbb{C}$ in $\mathbb{H}$ determine a single orbit of this latter action, which is isomorphic to $S^2$ with its $SO(3)$ action. Thus the stabilizer of any particular forgetful functor $U$ is $S^1$ , which means that for any $g$ in that $S^1$ (of course, different forgetful functors correspond to different copies of $S^1$ in $SO(3)$ ) we have $U g = U$ . But this just says that that copy of $S^1$ acts on the fibers of $U$ .

Posted by: Mike Shulman on February 9, 2011 3:33 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Now I’ll answer a couple more of Fred Lunnon’s questions:

Section 4 pp. 17–18 explains in detail why the “real” versus “symplectic” classification is meaningful, but on this showing “complex” seems to be merely a ragbag of leftovers: why should it count as a class on the same footing as the others?

That’s a good question. The short answer is “yes, it’s just a ragbag of leftovers”. The long answer would use Section 6 of my paper, where I treat the categories of real, complex, and quaternionic Hilbert spaces on a ‘separate but equal’ footing, and describe functors from each of these categories to each other one. If we studied irreducible unitary group representations of these three kinds, we would then see that each kind looks like the other two kinds plus a ragbag of leftovers.

Section 5 p. 19 mentions a connection between (matrix) representations and particle spin which is presumably familiar to physicists, but leaves me baffled. Exactly what aspect of the physical behaviour of an electron (say) is modelled by some spin-1/2 representation of SU(2), but not already modelled satisfactorily by quaternions [or SU(2) itself]?

You can’t model the electron well by $SU(2)$ itself, because its states form a vector space — this is the idea of quantum mechanics, that you can take ‘superpositions’ of states, meaning linear combinations. Normally physicists think of the space of states of the electron as a 2-dimensional complex Hilbert space, but I’m trying to say that for many purposes, it’s fine to use a 1-dimensional quaternionic Hilbert space.

I’m not prepared to say more here about what I mean by “for many purposes”. Everything I have to say about that is already in my paper.

This might help for understanding more about quantum mechanics and spin and SU(2) (but nothing about quaternions):

• Michael Weiss, Spin.

Posted by: John Baez on February 5, 2011 9:02 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Fred Lunnon wrote:

It’s 40 years since I first encountered category theory — and despite numerous attempts, some inspired by Baez’s evident enthusiasm, I still have no idea what it’s actually for. Can anybody explain to me, for instance, how its deployment enhances this particular paper?

I’m studying things like the collection of all quaternionic Hilbert spaces, and these things are categories.

I’m also studying things like the process of complexifying a real Hilbert space, or the process of taking the underlying complex Hilbert space of a quaternionic Hilbert space, and these things are functors between categories.

Indeed, I study six such functors going between $Hilb_{\mathbb{R}}$ , $Hilb_{\mathbb{C}}$ and $Hilb_{\mathbb{H}}$ . These are important for seeing how real, complex and quaternionic quantum mechanics fit together in unified structure.

However, I’m deliberately keeping the amount of category theory in this paper to a bare minimum; as you can see in my conversation with Mike Shulman here, it can easily get a lot worse!

Indeed, a certain segment of this paper is a kind of watering-down or popularization of an older paper where in one section I discussed real and quaternionic structures using a lot more category theory. I thought that was really cool. But nobody else seems to have felt that way, so I thought I’d try saying some of the same things in a less esoteric way.

Posted by: John Baez on February 6, 2011 4:04 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Okay, I think this is the last question.

Fred wrote:

I’m prepared to put up with a good deal of hand-waving from somebody who brings it off with sufficient conviction; however a particularly cryptic example early in section 1 (again!) quickly pulled me up short:

“Because we can think of a pair of complex numbers as a quaternion …”

Just in case anybody else spends as long as I did, scratching my head over this before eventually concluding it must actually be something I already knew…

Yeah, it’s just what you already knew.

While this wheeze is a useful mnemonic, it’s hard to see what significance it could possibly have in the present context!

I was just pointing out that the usual spin-1/2 rep of $SU(2)$ on $\mathbb{C}^2$ can be reinterpreted as the rep of unit quaternions on $\mathbb{H}$ by left multiplication. To do this, we reinterpret a pair of complex numbers as a single quaternion, in the way you described.

Of course the multiplication in $\mathbb{C} \oplus \mathbb{C}$ is different than that in $\mathbb{H}$ , but that’s okay.

Posted by: John Baez on February 6, 2011 9:51 AM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

You write on p. 7

Surprisingly, this is enough to let us carry out a bit of quantum theory as if $\mathbb{O}$ , $\mathbb{O}^2$ and $\mathbb{O}^3$ were well-defined octonionic Hilbert spaces.

Is there the possibility, then, in some sense of a $Hilb_{\mathbb{O}}$ , and if so could it find a place in chapter 6?

Posted by: David Corfield on February 7, 2011 12:55 PM | Permalink | Reply to this

Re: The Three-Fold Way (Part 4)

$MathML-enabled post (click for more details).$

Good question! I haven’t thought about what $Hilb_{\mathbb{O}}$ would be like, were it to exist.

I don’t really know the sense in which $\mathbb{O}^3$ is an ‘octonionic Hilbert space’, but I know that the set of ‘unit vectors mod phase’ should be $\mathbb{OP}^2$ , and I know that the relevant symmetry group of this, the isometry group, is the exceptional group $\mathrm{F}_4$ .

Whatever $Hilb_{\mathbb{O}}$ is, the group $Aut(\mathbb{O}) = \mathrm{G}_2$ should act on it.

So, this could be a way to put a couple of exceptional compact simple Lie groups into a nice context. All the nonexceptional ones show up in $Hilb_{\mathbb{R}}$ , $Hilb_{\mathbb{C}}$ and $Hilb_{\mathbb{H}}$ .

If we got the bioctonions, quateroctonions and octooctonions into the game, we might account for the other exceptional compact simple Lie groups: $\mathrm{E}_6$ , $\mathrm{E}_7$ , and $\mathrm{E}_8$ . That would be quite magical.

Posted by: John Baez on February 8, 2011 3:19 AM | Permalink | Reply to this

Read the post The Three-Fold Way (Part 5)
Weblog: The n-Category Café
Excerpt: Learn about the functors going between the categories of real, complex and quaternionic Hilbert spaces.
Tracked: February 8, 2011 3:25 AM

The n-Category Café

Skip to the Main Content

January 29, 2011