M13

February 10, 2013

M₁₃

Posted by John Baez

$MathML-enabled post (click for more details).$

People of a certain ilk wonder why we need groupoids. Why aren’t groups enough?

There are many answers to this question. The answers provided by Alan Weinstein and Ronald Brown should be convincing to any open-minded topologist or geometer. But what if you’re a group theorist… say, someone who studies finite groups? What do groupoids have to offer you?

I just noticed a nice answer to this question. The Mathieu group $M_{12}$ is somewhat easier to understand if you think of it as part of a groupoid. John Conway calls this groupoid $M_{13}$ .

Don’t confuse this with the Hercules Cluster, which is called M₁₃:

The font makes a big difference! The globular cluster M₁₃ is a Messier object. The groupoid $M_{13}$ is a less messy object.

$MathML-enabled post (click for more details).$

I’ve been looking for a good excuse for that pun for years. I could stop here and be completely satisfied! But now that I’ve got your curiosity up, let me describe $M_{12}$ and $M_{13}$ .

Finite simple groups come in a bunch of infinite families, but there are also 26 exceptional ones, the sporadic finite simple groups. The first of these to be discovered were the Mathieu groups, and the second smallest of these is $M_{12}$ . The smallest is $M_{11}$ , but this is conveniently described as the subgroup of $M_{12}$ that fixes a chosen point. So, anyone seriously interested in finite groups should get to know $M_{12}$ .

Until recently I just knew three descriptions of $M_{12}$ .

The easiest comes from Conway and Sloane’s classic book Sphere Packings, Lattices and Groups. If you get 12 equal-sized balls to touch a central one of the same size, and arrange them to lie at the corners of a regular icosahedron, they don’t touch their neighbors:

There’s even room to roll them around in interesting ways! For example, you can twist 5 of them around clockwise so that this arrangement:

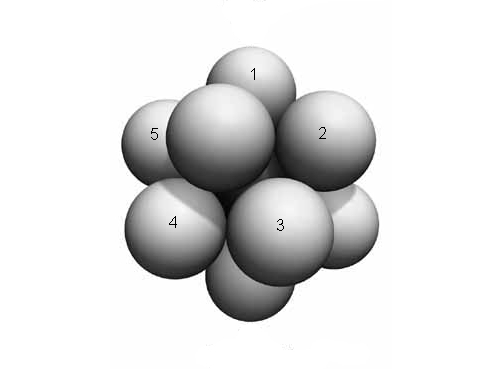
becomes this:

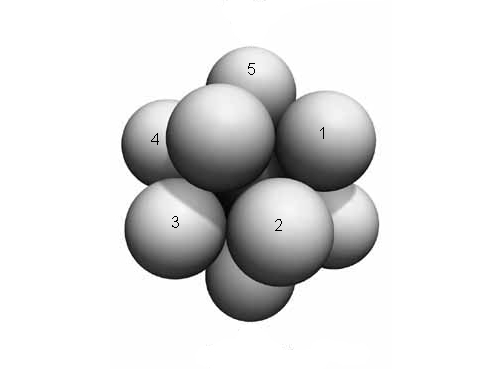
We can generate lots of permutations of the 12 outer balls using twists of this sort - in fact, all even permutations. But suppose we only use moves where we first twist 5 balls around clockwise and then twist 5 others counterclockwise. These generate a smaller group: the Mathieu group $M_{12}$ !

Here’s a second description of $M_{12}$ , which is very terse but much less concrete. Let’s say a group of permutations of some set is $k$ -tuply transitive if given any ordered $k$ -tuple of distinct elements of that set, and any other $k$ -tuple of this sort, there’s a permutation in the group that carries one to the other. It turns out that aside from the symmetric groups $S_n$ , which consist of all permutations of an $n$ -element set, and the alternating groups $A_n$ , which consist of all the even permutations, there are only four finite permutation groups that are $k$ -tuply transitive for $k > 3$ . Moreover, they’re all Mathieu groups!

This lets us characterize $M_{12}$ in a very speedy way. Up to isomorphism, it’s the only quintuply transitive group of permutations of a 12-element set other than $S_{12}$ and $A_{12}$ .

In terms of our ‘rolling ball’ description of $M_{12}$ , this means that given any list of 5 balls, and any other list of 5 balls, there exists an element of $M_{12}$ mapping the first list to the second. In fact there’s a unique element of $M_{12}$ that does the job, so we say this group of permutation is sharply quintuply transitive.

Unlike the first description, the second one doesn’t really let us get our hands on $M_{12}$ . But given that it’s sharply quintuply transitive, it’s at least easy to count the elements of $M_{12}$ . There must be one for each way to pick a list of 5 distinct things out of 12. So, this group has

$12 \times 11 \times 10 \times 9 \times 8 = 95040$

elements.

The third description goes is more concrete than the second. Up to isomorphism, there’s a unique way to choose a bunch of 6-element subsets of a 12-element set, called blocks, such that each 5-element subset lies in a unique block. Given this, $M_{12}$ consists of all permutations of the 12-element set that map blocks to blocks.

Of course, to get your hands on $M_{12}$ using this description, you need to know how to find 6-element blocks so that each 5-element subset lies in a unique block. If you were stuck on a desert island you could eventually figure it out. But since you’re not, I’ll tell you.

Take a 12-point set and think of it as the projective line over $\mathbb{F}_{11}$ : in other words, the integers mod 11 together with a point called $\infty$ . Among the integers mod 11, six are perfect squares:

$\{0,1,3,4,5,9 \}$

Let this set be a block. From this, we get all the other blocks by applying fractional linear transformations:

$z \mapsto \frac{a z + b}{c z + d}$

where the matrix

$\left( \begin{array}{cc} a & b \\ c & d \end{array}\right)$

has determinant 1. If the fractional linear transformation involves dividing by zero, we say it gives $\infty$ .

This description is nice because it connects $M_{12}$ to lots of good math. In fact, I’m no longer sure the description using the groupoid $M_{13}$ is better! But let me give it anyway—because even if it isn’t ‘better’, it’s still very pretty.

Start with the projective plane over $\mathbb{F}_3$ . This is the set of lines through the origin in a 3d vector space over $\mathbb{F}_3$ , but Bob Harris has drawn a nice picture of it:

Any two points lie on a unique line, and any two lines intersect in a unique point. Each line contains 4 points and each point lies on 4 lines. There are 13 points and 13 lines.

Now, put a ‘counter’ on each point except one. A move consists of moving a counter from any point $x$ to the empty point $y$ , then exchanging the two other counters on the line containing $x$ and $y$ .

$M_{13}$ consists of the permutations of counters that can be obtained by composing finite sequence of moves. This is not a group, since we can only compose two moves if the point left empty after carrying out the first move is the empty point at the start of the second move. But it’s a groupoid!

More precisely: the objects of $M_{13}$ are the 13 positions of the point that’s left empty. The morphisms are the permutations of counters that arise from finite sequences of composable moves.

$M_{12}$ can then be defined as the subgroupoid consisting of morphisms that leave a particular point empty. This is actually a group!

This should remind you of the famous 15-puzzle, which also gives a groupoid:

But the groupoid $M_{13}$ seems to be more important, mathematically speaking. You can read more about it here:

John H. Conway, Noam D. Elkies and Jeremy L. Martin, The Mathieu group $M_{12}$ and its pseudogroup extension $M_{13}$ , Experimental Mathematics 15 (2006), 223–236.
Mathieu groupoid, Wikipedia.

People of a certain ilk—the sort who challenge us to prove our interests are interesting—will ask how $M_{13}$ helps us prove new theorems about $M_{12}$ . I don’t know the answer to that question. Do you?

Another obvious question is whether a similar puzzle exists for $M_{24}$ , a much deeper group, which can be defined as the unique quintuply transitive group of permutations of 24 things. So far I’ve only seen a lonely MathOverflow question about this, with no answer.

Notice that if we think of groups as one-object groupoids, $M_{13}$ is equivalent, as a groupoid, to $M_{12}$ . So, arguably, this is a case where an important finite group is equivalent to a finite groupoid that’s easier to describe. So we should look for more. It would be nice if other sporadic finite simple groups were best understood using groupoids in this way, but I don’t have much evidence for that yet. Just $M_{13}$ .

And finally… here’s one more thing about $M_{12}$ , which I should mention before I forget.

Take a deck of 24 cards. Cut it exactly in half, so you’ve got 12 in each hand. Shuffle them perfectly, so the cards alternate. There are two ways to do this: the card that was originally on top can stay on top, or not. Keep doing this as often as you want. You get lots of permutations of the cards this way. How many? This many:

$2^{11} \times 12 \times 11 \times 10 \times 9 \times 8 = 2048 \times 95040$

And here’s the really cool part: they form a group that contains $M_{12}$ ! It’s the semidirect product of $M_{12}$ and $(\mathbb{Z}/2)^{11}$ . I recently learned this here:

Persi Diaconis, Ronald Graham and William M. Kantor, The mathematics of perfect shuffles, Advances in Applied Mathematics 4 (1983), 175–196.

I thank Graham Jones for pointing me in this direction, which eventually led me to read about $M_{13}$ .

Here’s a hint about why we get the semidirect product of $M_{12}$ and $(\mathbb{Z}/2)^{11}$ . The shuffles I’m talking about, called perfect shuffles, give permutations with a special property: they commute with turning the deck upside down! So, we can take our deck of cards and divide it into 12 pairs—the top card and the bottom card, the card second from the top and the card second from the bottom, and so on—and our shuffles will send each such pair to another pair.

So, we get permutations of these 12 pairs of the cards in our deck, and the group of permutations we get is $M_{12}$ . But we also get various ways of permuting the two cards within each pair… and these permutations form the group $(\mathbb{Z}/2)^{11}$ .

Why not $(\mathbb{Z}/2)^{12}$ ? Well, think about it… or read the paper!

Posted at February 10, 2013 12:02 AM UTC

TrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/2597

40 Comments & 0 Trackbacks

Re: M13

$MathML-enabled post (click for more details).$

$M_12$ isn’t the smallest sporadic group—that’s $M_11$ .

If you take the action of $M_12$ on $12$ points, then the subgroup which fixes one point is $M_11$ , which is also simple.

(In fact, $M_12$ has two different actions on $12$ objects, related by an outer automorphism of $M_12$ in a manner analogous to the two actions of $S_6$ on $6$ objects. Indeed, in some ways, $M_12$ is like a sort of hyped-up $S_6$ . As you mentioned, $M_12$ sends the $6$ -point “blocks” to blocks in the action on $12$ points. (The complement of a block is also a block.) The subgroup of $M_12$ which sends a block to itself is $S_6$ (this follows immediately from the fact that $M_12$ is $5$ -transitive). This $S_6$ subgroup acts as all permutations on each of the complementary blocks, but via two different actions, related by an outer automorphism of $S_6$ . $M_12$ fits into $M_24$ in the same way, but the process stops there: $M_24$ has only trivial outer automorphism group.)

Posted by: Tim Silverman on February 10, 2013 10:13 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Whoops! I’ll fix my blog article. I feel that $M_{12}$ is good to tackle before $M_{11}$ , sort of like how the $E_8$ lattice is good to tackle before the $E_7$ and $E_6$ lattices: these smaller objects are conveniently described as portions of the bigger one. But I’m a lot less familiar with Mathieu groups than these lattices… and I momentarily completely forgot about $M_{11}$ !

I really like your idea of $M_{12}$ as a ‘doubled’ $S_6$ and $M_{24}$ as a ‘doubled’ $M_{12}$ .

Posted by: John Baez on February 10, 2013 4:43 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Do you mean “dodecahedron”?

Posted by: Tom Ellis on February 10, 2013 10:42 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

I take that back. I see you said “corners” not “faces”! Good old duality.

Posted by: Tom Ellis on February 10, 2013 10:44 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

In a similar way, deleting one strand of a braid does not form a homomorphism of braid groups, but deleting one strand of a pure braid does form a homomorphism of pure braid groups. But, I first read about the beautiful nonsense related to that over on TWF, so I expect you’re way ahead of me, there.

Posted by: Jesse C. McKeown on February 10, 2013 2:54 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

“The smallest is M₁₁, but this is conveniently described as the subgroup of M₁₁ that fixes a chosen point.”

Should that be “as the subgroup of M₁₂ that fixes a chosen point”?

Posted by: David on February 10, 2013 5:30 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Yes, thanks - fixed.

Posted by: John Baez on February 10, 2013 5:40 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Over on Google+, Ramsay Dyer wrote:

I recently read your post on the Klein quartic thanks to a post from Roice Nelson. The symmetry group is shown to be that of the Fano plane, which I guess is the projective plane of order 2. The relation of $M_{12}$ to this projective plane of order 3 is more complicated, but it made me wonder why none of the three mentioned descriptions of $M_{12}$ involved the group of symmetries of some surface with constant curvature. I guess that if such a characterisation exists it is too complicated to be enlightening?

I replied:

I hadn’t looked for a description of $M_{12}$ as the symmetry group of a surface of constant curvature. People are busy trying to understand how $M_{24}$ shows up in string theory, and this involves K3 surfaces. These are complex surfaces, so they have 2 complex dimensions or 4 real dimensions. I don’t understand this stuff, but this paper seems less unreadable than most:

Miranda Cheng, K3 surfaces, N=4 dyons, and the Mathieu group $M_{24}$ .

Let me quote a bit:

In the last century, S. Mukai [4] considered the finite groups which act on K3 surfaces and fix the holomorphic 2-form, a condition tied to the physical condition of supersymmetry and defining the so-called symplectic automorphisms, and showed that all of them can be embedded inside the sporadic group $M_{23}$ . This relation between the classical geometry of K3 and the Mathieu group was later further explained by S. Kondo [5] from a lattice theoretic view point.

Before discussing the recent observations relating K3 surfaces and the largest Mathieu group $M_{24}$ , let us first comment on the possible origin of the appearance of $M_{24}$ in the elliptic genus. Since it was shown that all symplectic automorphisms of K3 surfaces can be embedded in M23, the idea that the full $M_{24}$ acts on the spectrum of CFT [conformal field theories] on K3 seems simply excluded. The fallacy of such an argument lies in the fact that the moduli space of string theory on K3 surfaces includes more than just the classical geometry part.

Since $M_{12}$ is contained in $M_{24}$ , I bet it’s one of the finite groups Mukai considered.

Posted by: John Baez on February 10, 2013 7:12 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Then I added:

Oh, by the way: Hurwitz showed that a $g$ -holed torus with constant curvature can have at most $84(g-1)$ symmetries that preserve the metric and the orientation:

Hurwitz’s automorphism theorem, Wikipedia.

The proof is fairly amazing: you can’t help but wonder where the number 84 shows up! Wikipedia explains why.

The Klein quartic is as symmetrical as possible: it has $g = 3$ holes and $168$ symmetries. But since the Mathieu group $M_{12}$ has $95040$ elements, to get it to show up as a symmetry group this way we’d need a surface with at least

$95040/84 + 1 = 1132.42\dots$

holes. That is, at least 1133 holes. Since this number is large and the hole number isn’t even a whole number, I suspect that looking in this direction is not likely to be fruitful. The surface could exist yet still not be enlightening.

Posted by: John Baez on February 10, 2013 8:22 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

And as is well known, there is also the interesting relationship between $M_{12}$ and the complex Leech lattice. Perhaps the $M_{13}$ groupoid further illuminates this relationship.

There might also be a slick groupoid approach to studying R.A. Wilson’s octonionic Leech lattice construction. Wilson was able to show the full automorphism group of the Leech lattice $Co_{0}$ is generated by symmetries that can be written as $3\times 3$ matrices over the octonions. Going a small step further, one can show these matrices are determinant preserving, so are of type $E_{6(-26)}$ . Hence, symmetries of the Leech lattice can be seen as collineations (or isometries) of the Cayley plane.

Posted by: Mike Rios on February 10, 2013 8:44 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Wow, that stuff looks cool! Thanks for pointing it out! I’d long been hoping for a nice connection between the Leech lattice and octonions, simply because $3 \times 8 = 24$ . My friend Geoffrey Dixon did some work on this. If it really solidifies, someone could try to give an octonionic formulation of heterotic string theory using these ideas.

I don’t know the well-known relationship between $M_{12}$ and the complex Leech lattice. I could ask random people in the café here, but I suspect it’s not quite that well-known. Could you say what that is, or give me a pointer?

(Of course I could Google it….)

Posted by: John Baez on February 10, 2013 9:30 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Yes, Wilson used three copies of the $E_8$ lattice to construct the octonionic Leech lattice. Connecting this to heterotic strings would be exciting indeed. I suspect the Cayley plane will play a role here.

The relationship between $M_12$ and the complex Leech lattice is the one mentioned on pg. 201 of Conway’s Sphere packings book, with $2M_12$ as the automorphism group of the extended ternary Golay code.

Posted by: Mike Rios on February 10, 2013 10:31 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Ok, that’s just some fan post from a math learner, who just likes to say thank you for this delightful post!

Mike Rios says “And as is well known..”.

Where have you guys learned all that stuff? I only know one book in my university library that talks about sporadic groups, Leech lattices, octonions, E8 etc. and that is Sphere Packings, Lattices and Groups. But I find this one often extremely hard to decipher.

Of course, there is JB over the years and some occasional posts and questions from other folks on the net. But why have no other authors put out books about these beautiful mathematical objects yet? Is this some oral tradition, not in the literature thing?

Asked more directly: what’s the matter with you mathematicians?!

(And where to start reading about groupoids?)

Posted by: Kris on February 10, 2013 9:33 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

I’m glad you liked this post!

Where have you guys learned all that stuff?

Reading papers and books, I guess.

I only know one book in my university library that talks about sporadic groups, Leech lattices, octonions, E8 etc. and that is Sphere Packings, Lattices and Groups. But I find this one often extremely hard to decipher.

I find this one easy to decipher, probably because I’ve spent decades reading math books. But it’s often a bit tiring to read, because it contains a lot of facts and I find it easier to read math when the facts are connected to form ‘stories’. So, I’ve been reading and rereading this book for many years, and learning more each time.

The bibliography of this book is HUGE, so there’s no shortage of stuff to read! But here are a few other things - I’ll try to pick ‘fun’ ones:

John Baez, The octonions.
John Baez, Klein’s quartic curve.
John H. Conway and Derek A. Smith, On Quaternions and Octonions: Their Geometry, Arithmetic and Symmetry, A. K. Peters Ltd., 2003. (Review here.)
John H. Conway, Heidi Burgiel and Chaim Goodman-Strauss, The Symmetries of Things, A. K. Peters Ltd., 2008. (Video here.)
David A. Richter, How to make the Mathieu group $M_{24}$ .

I should also emphasize that there’s a HUGE amount of material available on Wikipedia, especially if you go to the references. These days, whenever I want to get started on a mathematical topic, I start there. It may not say everything, and it may not explain it well… but everything there is ‘well-known’ material that’s worth knowing.

MathOverflow is also useful… and of course the arXiv.

(And where to start reading about groupoids?)

I gave two references right at the beginning of this post: click on those guy’s names! The Wikipedia article is also a good place to start.

Posted by: John Baez on February 10, 2013 9:55 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Many thanks again!! Very much appreciated.

Maybe I have to give Sphere Packings, Lattices and Groups another try!

I guess, I am just too spoiled by your articles! It’s always such a great fun reading them.

All the best, Kris

Posted by: Kris on February 11, 2013 12:54 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Thanks! This book I mentioned is supposed to be extremely fun, full of jokes and puns but also lots of good group theory:

• John H. Conway, Heidi Burgiel and Chaim Goodman-Strauss, The Symmetries of Things, A. K. Peters Ltd., 2008.

Posted by: John Baez on February 11, 2013 7:23 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

The second time $M_13$ has shown up on this blog. I was wondering in the very early days about that idea

… that sporadic groups are not groups, they are representatives of some wider class of objects, only finitely many of which have happened, by chance, to be groups.

I dare say something deeper than groupoids would be needed. Remember the discussion after my miserable attempt to capture a talk given by John McKay. There was some speculation about higher dimensional algebraic structure.

I see John McKay himself contributed, pointing us to Chevalley’s Tohoku construction, as he had done before.

Posted by: David Corfield on February 11, 2013 9:48 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

David wrote:

I was wondering in the very early days about that idea

… that sporadic groups are not groups, they are representatives of some wider class of objects, only finitely many of which have happened, by chance, to be groups.

I dare say something deeper than groupoids would be needed.

I vaguely remembered those remarks but didn’t review them while writing my post here. I see now that you mentioned Conway et al’s paper The Mathieu group $M_{12}$ and its pseudogroup extension $M_{13}$ . I’m sure that when I saw that title, I never guessed that ‘pseudogroup’ meant groupoid!

I can imagine certain people complaining that this title is yet another case of denying groupoids their rightful day in the sun. Less conspiracy-minded myself, I’m still surprised the authors didn’t use the standard term here. But I’m very happy that the Wikipedia articles is titled Mathieu groupoid, and as soon as I saw that I got interested.

It’s remarkable how easy it is to describe $M_{13}$ compared to $M_{12}$ . I’d be shocked if all the other sporadic finite simple groups were so easily described using groupoids, but someone should at least try. I have an itch to tackle $M_{24}$ , but really Conway should try it. And if he’s already tried and failed…

This Mathieu group business is a numerologist’s dream. For example, I read:

$M_{21}$ has 168 simple subgroups of order 360 and 360 simple subgroups of order 168. In the larger projective general linear group $PGL(3,4)$ both sets of subgroups form single conjugacy classes…

How insane is that?

Posted by: John Baez on February 12, 2013 4:54 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

$M_{21}$ is $PSL(3, 4)$ . That’s the one that Sasha Borovik told me

probably holds the world record for the most bizarre Schur multiplier.

Just how bizarre is it? I can’t see it noted anywhere.

John McKay pointed us to the Schur multiplier to better understand the sporadics.

Posted by: David Corfield on February 12, 2013 9:48 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

David wrote:

Just how bizarre is it? I can’t see it noted anywhere.

The Schur multiplier of $PSL(3,4)$ seems to be calculated here as direct product of cyclic groups of order 4, 4 and 3.

For those who forget what a Schur multiplier is, it’s the second group cohomology with integer coefficients, so I think for finite groups it helps us find the universal central extension.

In the process of finding this fact I also found that the Schur multipliers of $M_{12}$ and $M_{22}$ were incorrectly computed in 1966, and these calculations were corrected in 1968.

Also, in 1898, G. A. Miller published a paper mistakenly claiming to prove that $M_{24}$ does not exist!

Posted by: John Baez on February 12, 2013 4:35 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Does not Schur multiplier also refer to a particular representative of that cohomology group, i.e a function f: G times G to A?

Posted by: jim stasheff on February 13, 2013 12:49 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Hmm, bizarre meant very large, I guess.

That seems to be the largest of the simple groups , but there is the Steinberg group, $^2A_3(3^2)$ , with multiplier order 36.

The Chevalley group, $A_2(4)$ , is just $M_21$ again.

Posted by: David Corfield on February 13, 2013 9:16 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

It’s not just large, it’s large compared to what would be expected for a member of the $PSL(n, q)$ series. $PSL(n, q$ ) usually has a cyclic Schur multiplier of order $(n, q-1)$ , so we’d expect $PSL(3, 4)$ to have a multiplier of order $3$ anyway. It’s the extra factor of $4^2$ that’s particularly surprising.

Similarly, $PSU(n, q)$ (aka $^2A_{n-1}(q)$ ) usually has a cyclic multipler of order $(n, q+1)$ , so we’d expect $PSU(4,3)$ to have a multiplier of order $4$ . But there’s an extra surprising factor of $3^2$ .

There may be something else surprising about the way these exceptional Schur covers arise, but I can’t remember how they come about…

Posted by: Tim Silverman on February 13, 2013 9:58 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Very nice! A couple of questions:

1). Does anyone know of a nice web-app with which one could play with sliding counters on the projective plane over $F_3$ , like a sliding-block puzzle? That would be a good way to get a feel for how $M_{13}$ and $M_{12}$ work and relate to one another.

2). The first three descriptions of $M_{12}$ all involve the number 5: twisting 5 points of an icosahedron; the quintuple-transitivity of the group; and the 5-element subsets involved in picking out blocks. Is there a nice way to see how the number 5 shows up in the new description as well?

Posted by: Stuart Presnell on February 11, 2013 11:04 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Stuart wrote:

Does anyone know of a nice web-app with which one could play with sliding counters on the projective plane over $\mathbb{F}^3$ , like a sliding-block puzzle?

This page claims to provide a link to such a game:

Lieven le Bruyn, Conway’s puzzle M(13).

But alas, clicking on the link gives only the message

This site is currently under construction.

For inquiries contact: sebastian_egner(at)yahoo.com

If you want to politely pester him, that would be nice.

In the meantime, you can try this:

Puzzles: simple groups at play, Scientific American, 2008.

This gives puzzles illustrating the 95040-element Mathieu group $M_{12}$ , the 244823040-group Mathieu $M_{24}$ , and the 8315553613086720000-element Conway group $Co_0$ , which is the automorphism group of the Leech lattice.

The first three descriptions of $M_12$ all involve the number 5: twisting 5 points of an icosahedron; the quintuple-transitivity of the group; and the 5-element subsets involved in picking out blocks. Is there a nice way to see how the number 5 shows up in the new description as well?

Hmm. Dunno—fun puzzle.

Posted by: John Baez on February 12, 2013 5:10 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

I contacted Sebastian Egner and he sent me the files of the original Java program. It gives a very nice playable version of the sliding block puzzle, and even has a solver that can work out what moves are needed to reset any given derangement of the pieces.

I think he’d be happy for someone else to host it and make it available for educational and recreational purposes, if someone’s willing to do that. (I’m checking with him to confirm.)

(I’m also working on an implementation of the puzzle played on the projective plane over F3, as shown in Bob Harris’s drawing above, which I’ll make available when it’s finished.)

Posted by: Stuart Presnell on March 23, 2013 12:34 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Thanks, Stuart! If Sebastian Egner okays it, you can send me the Java program and I’ll either host it or find someone who can. Full credit will be given to Egner, of course. If he has a page written in HTML about this puzzle, it would be good if he/you could pass that on too.

Posted by: John Baez on March 23, 2013 7:29 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

If you really want to `play’, see Igor Kriz’s home page:

Sporadic simple puzzles

Now featured in the Scientific American.

If you ever tried the 15-puzzle or Rubik’s cube, you may be interested in the following three puzzles, which were programmed by Paul Siegel, a Michigan undergraduate, as a part of an REU project. The puzzles should run on any Windows32 machine.

M12.exe

M24.exe

DottoPrj.exe

For instructions, or to find out how solving these puzzles will help you learn about Sporadic simple groups without taking a course in advanced algebra, click here: README

Here is a picture of a prototype of the Number Planet, a mechanical puzzle designed by Oskar Van Deventer and myself, and based on M12. For more questions, please email me.

Posted by: jim_stasheff on February 14, 2013 12:54 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

What a lovely post!

It’s philosphically interesting how “nature” hands us groupoids. I’m not seeing all of how it was “handed” to us in this case- I have some questions.

What’s the motivation for the “moves”? Why move a counter into an empty position, then reverse the other two? Generalizing, what if I have more than one empty position? What if I take a projective plane over another finite field and play analogous games? In what sense was the game played to obtain M₁₃ “handed to me by nature”?

Posted by: Daniel Moskovich on February 14, 2013 2:56 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Dan wrote:

What’s the motivation for the “moves”?

Of course Conway invented them because he wanted a new improved way to describe the famous and important group $M_{12}$ .

We may afterwards begin to understand a deeper meaning to this, if we’re clever enough…

What if I take a projective plane over another finite field and play analogous games?

This is a good way to see if there’s a deeper meaning. Propose some specific ‘analogous games’ and see what groupoids they give!

One nice thing about the projective plane over $\mathbb{F}_3$ is that each line has 4 points, so that moving a counter from one point to another determines a line with exactly 2 other points, whose counters we can switch.

If we used $\mathbb{F}_2$ , each line would have 3 points, so that moving a counter from one point to another would determine a line with exactly 1 other point, whose counter we could not move. What groupoid does this give?

(Now it’s dinnertime, so I leave this as a small puzzle…)

Posted by: John Baez on February 14, 2013 3:42 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Let’s see… If I understand this correctly, we have 7 points and 7 lines, 3 points on each line, each pair of lines intersecting at exactly one point. Playing the analogous game gives me a certain groupoid, and (if I understand this correctly) the subgroupoid consisting of morphisms that leave a particular point empty seems to me to be the symmetric group S₆.

If that’s correct, then we can ask about F₃ if we leave two counters empty. We can only perform our move on a line with 3 full counters, so this cuts down the number of morphisms. Which groupoid do we get? Which group do we get by leaving two particular points empty?

Another question would be F₅, where each line has 6 points, and when we move a counter into an empty place along a line, the 4 other counters have their orders inverted. Might this give an interesting groupoid?

Posted by: Daniel Moskovich on February 14, 2013 2:57 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Daniel wrote:

Let’s see… If I understand this correctly, we have 7 points and 7 lines, 3 points on each line, each pair of lines intersecting at exactly one point. Playing the analogous game gives me a certain groupoid, and (if I understand this correctly) the subgroupoid consisting of morphisms that leave a particular point empty seems to me to be the symmetric group $S_6$ .

Yes, that sounds right.

If that’s correct, then we can ask about $\mathbb{F}_3$ if we leave two counters empty. We can only perform our move on a line with 3 full counters…

What’s “our move”? Not the move I described, where you move the empty counter from one point to another point on the same line. You must have some other move in mind.

Another question would be $\mathbb{F}_5$ , where each line has 6 points, and when we move a counter into an empty place along a line, the 4 other counters have their orders inverted. Might this give an interesting groupoid?

This move is ill-defined. There’s no order on the points on a line in a projective plane. So, ‘reversing the order’ doesn’t make sense, unless we go ahead and put an order on each line, which breaks the symmetry of our structure. That’s why $\mathbb{F}_3$ was so special! We didn’t need to do this.

Posted by: John Baez on February 14, 2013 4:23 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

John wrote:

There’s no order on the points on a line in a projective plane. So, ‘reversing the order’ doesn’t make sense, unless we order each line, which breaks the symmetry of our structure.

Here I was trying to emphasize that creating puzzles that don’t break the symmetry of the projective plane over $\mathbb{F}_q$ - that is, the projective linear group $PGL(2,\mathbb{F}_q)$ , often called $PGL(2,q)$ - is not so easy!

Conway’s $M_{13}$ puzzle doesn’t break the symmetry, so the whole group $PGL(2, \mathbb{F}_3)$ acts on his puzzle and thus on the groupoid $M_{13}$ .

Looking for similar puzzles that don’t break the $PGL(2,q)$ symmetry would be an interesting challenge. This could shed light on why $M_{13}$ is special.

But I wasn’t trying to say that orienting the lines in the projective plane is always a bad idea. For example, to define the octonions starting from the projective plane over $\mathbb{F}_2$ :

we need to choose a cyclic ordering on each line, like this:

For details read this.

Posted by: John Baez on February 14, 2013 6:59 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

This makes sense- thanks!

What our move? Not the move I described, where you move the empty counter from one point to another point on the same line. You must have some other move in mind.

My subconscious image of the move is: Reflect the (oriented) line across the empty point, and simultaneously rotate it one unit. I was subconsciously orienting it…

So for the case of 3 points per line, looking from the empty point X down a line, we see two points in sequence, say AB. If we now swap X and A, then, looking down the line from X, we see BA. For 4 points per line it is the same story- if before the move we saw ABC, after the move, we see CBA.

Another way of saying the same thing (I think): Reverse the orientation of the line, and rotate it one unit.

Of course, this generalizes for any configuration of oriented circles- I can always change orientation while rotating. If the circles have a finite number of points, this is well-defined I think. The moves generate a groupoid, and we can ask for the stabilizer of a point (or of some set of points).

I wonder which groups naturally arise this way…

Posted by: Daniel Moskovich on February 15, 2013 5:59 AM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

For a complete representation on the 480 possible permutations of directed Fano plane octonions, please see my Mathematica demonstration (requires Mathematica CDF plugin).

BTW - it also links the 240 split real even E8 vertices and Lisi fundamental particle assignments.

Posted by: jgmoxness on February 14, 2013 11:35 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Wait a second…by groupoid, do you mean:

(a) A magma, i.e., a set equipped with some binary operation;

(b) A category whose arrows are invertible?

Because some of the references seem to use (a) as the definition, while others use (b)…and it scares me!

Posted by: Alex on February 18, 2013 6:26 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

He means a category with all arrows invertible.

Posted by: Todd Trimble on February 18, 2013 7:25 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

Here on the n-Café we always say ‘magma’ for magma, and ‘groupoid’ for a category with all arrows invertible. I said:

More precisely: the objects of $M_{13}$ are the 13 positions of the point that’s left empty. The morphisms are the permutations of counters that arise from finite sequences of composable moves.

‘Morphism’ is a synonym for ‘arrow’.

Posted by: John Baez on February 18, 2013 7:40 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

More Baez-relevant “M13” (it’s a globular cluster!; it’s a groupoid!; it’s a dessert topping!) here (with some PR)

Posted by: Richard on February 24, 2013 5:16 PM | Permalink | Reply to this

Re: M13

$MathML-enabled post (click for more details).$

circular DNA - so is it knotted?

Posted by: jim stasheff on February 26, 2013 1:10 PM | Permalink | Reply to this

The n-Category Café

Skip to the Main Content

February 10, 2013

Posted by John Baez

Some Related Entries

40 Comments & 0 Trackbacks

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Re: M13

Access Keys:

M₁₃