The n-Category Café

Introduction

First, we introduce the place we work, $\mathsf{Fsetcat}$, that is a category of categories enriched over the category of filtered sets ($\mathsf{Fset}$). The symmetric monoidal category $\mathsf{Fset}$ contains $\Set$ and $[0, \infty]$ as full submonoidal categories, hence the category $\mathsf{Fsetcat}$ contains $\mathsf{Cat}$ and $\mathsf{GMet}$ (category of generalized metric space). Then by the framework of Leinster and Leinster–Shulman, we can consider the magnitude of “finite” $\mathsf{Fset}$-categories, that is an extension of both of the Euler characteristic of finite categories and the magnitude of finite metric spaces, introduced by Leinster.

Second, we give a description of metric fibration, introduced by Leinster, as the “Grothendieck fibration”. In precise terms, we generalize the notion of Grothendieck fibration of small categories in $\mathsf{Fset}$-enriched setting, and then we restrict it to $\mathsf{Met}$ (category of metric spaces). Such a restriction coincides with the definition of metric fibrations. Further we obtain the notion of metric actions that plays a role of lax functors in $\mathsf{Cat}$ case, and we show a correspondence between them via the Grothendieck construction.

Finally, we give a classification of metric fibrations, which is parallel to that of topological fiber bundles. That is, the classification of metric fibrations is reduced to that of “principal fibrations” (torsors in $\mathsf{Met}$), which is done by the “1-Čech cohomology” in an appropriate sense. Further, we can define the “fundamental group $\pi^m_1(X)$” of a metric space $X$, which is a group object in $\mathsf{Met}$, such that the conjugation classes of homomorphisms $\mathsf{Hom}(\pi^m_1(X), \mathcal{G})$ corresponds to the isomorphism classes of $\mathcal{G}$-torsors over $X$. Namely, it is classified like topological covering spaces.

Filtered sets

First of all, we introduce a workspace $\mathsf{Fsetcat}$ that contains $\mathsf{Cat}$ and $\mathsf{GMet}$. This large category inspires us to deal with objects sometimes like a small category and sometimes like a metric space.

Definitions

• A filtered set is a set $X$ with subsets $X_{\ell} \subset X$ for any $\ell \in [0, \infty]$ satisfying that $X_{\ell} \subset X_{\ell'}$ for any $\ell \leq \ell'$ and $X_\infty = \bigcup_{\ell \in \mathbb{R}_{\geq 0}}X_{\ell} = X$. We formally define that $X_{\ell} = \emptyset$ for $\ell \lt 0$.

• A filtered map $f : X \longrightarrow Y$} is a map with $f X_{\ell} \subset Y_{\ell}$ for any $\ell \in [0, \infty]$.

• We define $X \otimes Y$ by $(X\otimes Y)_{\ell} = \bigcup_{\ell' + \ell'' = \ell}X_{\ell'} \times X_{\ell''}$.

• For $x \in X$, we define $\deg x = \ell$ if $x \in X_{\ell} \setminus \bigcup_{\ell' \lt \ell }X_{\ell'}$. We have $\deg(x, y) = \deg x + \deg y$ for any $(x, y) \in X\otimes Y$.

We denote the category of filtered sets by $\mathsf{Fset}$. We can consider $\mathsf{Fset}$ as the subcategory of separated presheaves in $\Set^{[0, \infty]^{\mathrm{op}}}$, and then the tensor product is the Day convolution.

We denote the yoneda functor $[0, \infty] \longrightarrow \Set^{[0, \infty]^{\mathrm{op}}}$ by $\mathsf{y}$. The filtered set $\mathsf{y}(r)$ consists of only one element with degree $r$. Note that $\mathsf{y} (\infty) = \emptyset$. It can be checked that $(\mathsf{Fset}, \otimes )$ is a symmetric monoidal category with the unit object $\ast(0)$.

Further, there is a symmetric monoidal embedding $\Set \longrightarrow \mathsf{Fset}$ sending a set $X$ to a filtered set with $\deg x = 0$ for any $x \in X$. Now we have the following left diagram of monoidal embedding, that induces the right diagram of embedding.

Here, we denote the category of $\mathsf{Fset}$-categories by $\mathsf{Fsetcat}$. We also denote the category of $[0, \infty]$-categories by $\mathsf{GMet}$, the category of generalized metric spaces.

Benefits from $\mathsf{Fsetcat}$

Examples of magnitude

Before we consider metric fibrations from our view point $\mathsf{Fsetcat}$, we list up here some other benefits we obtain from there.

• We can define magnitude and (co)weighting on a class of $\mathsf{Fset}$-categories with a finiteness condition. It contains usual magnitude and (co)weighting of finite metric spaces, and also the Euler characteristic of finite categories introduced by Leinster.

• We can consider magnitude (co)weighting for broader class of metric spaces than so far. (This is actually not a benefit from the consideration of $\mathsf{Fsetcat}$ essentially, but I reached it in the process of defining magnitude on $\mathsf{Fsetcat}$. ) Namely, we can consider magnitude (co)weighting of locally finite graphs being not necessarily finite.

  If a metric space is finite, then we can compute its magnitude by summing up the magnitude (co)weighting. If a graph is not finite, it is not possible since the sum diverges, however, we can still consider its (co)weighting when the graph is locally finite.

  For example, let’s consider the Cayley graph of a finitely generated group. Then its magnitude (co)weighting takes the same value at each vertex, and it coincides with the inverse of growth series. The growth series of a finitely generated group is defined as $\sum_{g \in G} q^{\mathsf{wl}g}$. Here, $\mathsf{wl}$ is the word length with respect to a generating system, and the “inverse” is taken in the formal power series ring $\mathbb{Z}[[q]]$. This observation may be a foothold for applying magnitude theory to geometric group theory. We can also consider the Poincaré polynomial of a ranked poset as a magnitude weighting. (This is possible without extending the definitions of magnitude.)

MH as HH

We can also define magnitude homology of $\mathsf{Fset}$-categories. Leinster–Shulman pointed out that the magnitude homology has a form of Hochschild homology in a generalized sense. Here, by considering a metric space like a small category in $\mathsf{Fsetcat}$, we obtain a more ring theoretic description.

Theorem   For $C \in \mathsf{Fsetcat}$, we have an isomorphism $$\mathsf{MH}^{\ell}_\bullet C \cong \mathrm{Gr}_\ell \mathsf{HH}_\bullet(\mathrm{Gr}P_C(\mathbb{Z}), M_C(\mathbb{Z}))$$ for any $\ell \in \mathbb{R}_{\geq 0}$. Hence we have $$\bigoplus_{\ell\geq 0}\mathsf{MH}^{\ell}_\bullet C \cong \mathsf{HH}_\bullet({\mathrm{Gr}}P_C(\mathbb{Z}), M_C(\mathbb{Z})).$$

Here, $\mathrm{Gr}P_C(\mathbb{Z})$ is the “category algebra of $\mathsf{Fset}$-categories” defined as follows.

Definition   For $C \in \mathsf{Fsetcat}$, we define a filtered ring $\mathrm{Gr}P_C(\mathbb{Z})$ by:

• $\mathrm{Gr}P_C(\mathbb{Z}) = \mathbb{Z}\mathrm{Mor} C$ with $(\mathrm{Gr}P_C(\mathbb{Z}))_{\ell} = \mathbb{Z} \{f \in \mathrm{Mor} C \mid \deg f \leq \ell\}$ as a filtered abelian group.

• For any $f, g \in \mathrm{Mor} C$, we define an associative product $\cdot$ by
  $$f\cdot g = \begin{cases}g\circ f & \text{if }  t f = s g  \text{ and }  \deg g\circ f = \deg f + \deg g, \\ 0 & \text{ otherwise}.\end{cases}$$

We also define an action of $\mathrm{Gr}P_C(\mathbb{Z})$ on the abelian group $M_C(\mathbb{Z}) := \mathbb{Z}(\mathrm{Ob} C\times \mathrm{Ob} C)$ from the right and the left by

$$f\cdot (a, b) = \begin{cases} (s f, b) & \text{ if }  t f = a , \\ 0 & \text{ otherwise},\end{cases}$$

and

$$(a, b) \cdot f = \begin{cases} (a, t f) & \text{ if }  s f = b , \\ 0 & \text{ otherwise}.\end{cases}$$

We use techniques of homology theory for small categories to give such an expression. This can also be considered as a kind of generalization of Gerstenhaber–Schack’s theorem asserting that the cohomology of a simplicial complex is isomorphic to the Hochschild homology of the incidence algebra.

Homotopies on $\mathsf{Fsetcat}$

By the usual nerve construction for small categories, we can construct a filtered simplicial set and filtered chain complex from a $\mathsf{Fset}$-category. We say a filtered set $X$ is $\mathbb{Z}$-filtered if any $x \in X$ has an integral degree. We denote the category of $\mathbb{Z}$-filtered sets by $\mathbb{Z}\mathsf{Fset}$.

For a $\mathbb{Z}\mathsf{Fset}$-category, we have a $\mathbb{Z}$-filtered chain complex by the above construction, and we can construct a spectral sequence $E$ from it. Then we have the following.

Theorem   Let $C \in \mathbb{Z}\mathsf{Fsetcat}$.

• $E^1_{p, q} = \mathsf{MH}^{p}_{p+q}C$.

• If $C$ is a digraph, we have $E^2_{p, 0} = \widetilde{H}_p C$, where $\widetilde{H}_\bullet$ denotes the reduced path homology introduced by Grigor’yan–Muranov–Lin–S.-T. Yau et al.

• It converges to the homology of the underlying small category $\underline{C}$ if it converges, in particular when $\max \{\deg f \mid f \in \mathrm{Mor} C\}$ is finite.

Now we discuss homotopy invariance of each page of this spectral sequence. We show that the $(r+1)$-th page of this spectral sequence is invariant under “$r$-homotopy”.

Two morphisms $f, g : C \longrightarrow D \in \mathsf{Fsetcat}$ are $1$-step $r$-homotopic if there is a morphism $H : C \otimes I_r \longrightarrow D$ with $H_0 = f, H_1 = g$. Here, $I_r$ is the $\mathsf{Fset}$-category with just two objects $0, 1$ and one non-trivial morphism from $0$ to $1$ with degree $r$.

The equivalence relation generated by “$1$-steps” is the $r$-homotopy. For example:

• $0$-homotopy for small categories is exactly the natural transformation.

• $1$-homotopy for digraphs is exactly the digraph homotopy.

• $r$-homotopy for metric spaces is exactly the $r$-closeness of Lipschitz maps. Namely, Lipschitz maps $f, g : X \longrightarrow Y$ are $r$-homotopic if and only if $d(f x, g x) \leq r$ for any $x \in X$.

Theorem   The $(r+1)$-page of the above spectral sequence $E^{r+1}_{\bullet\bullet}$ is invariant under $r$-homotopy.

This contains the “digraph homotopy invariance of GLMY homology”. Further, this result suggests us that there are “$r$-homotopy theories” on $\mathsf{Fsetcat}$ that are the lifts of the following model structures on filtered chain complexes.

Theorem (J. Cirici, D. -E. Santander, M. Livernet and S. Whitehouse)  On the category of bounded filtered chain complexes, there is a model structure $M_r$ for each $r\geq 0$ such that the weak equivalences are the chain maps inducing quasi-isomorphisms on the $E^r$ term.

We can also expect that the following cofibration category structure on digraphs is a part of the “1-homotopy theory”.

Theorem (D. Carranza, B. Doherty, M. Opie, M. Sarazola and L.-Z. Wong)   On the category of digraphs, there is a cofibration category structure such that the weak equivalences are the digraph maps inducing isomorphisms on the GLMY homology.

Metric fibrations

Original definition by Leinster

First we recall the definition of metric fibration given by Leinster.

Definition (Leinster) Let $\pi : E \longrightarrow X$ be a Lipschitz map between metric spaces. We say that $\pi$ is a metric fibration over $X$ if it satisfies the following: for any $\varepsilon \in E$ and $x \in X$, there uniquely exists $\e_x \in \pi^{-1}x$ such that

• $d_E(\e, \e_x) = d_X(\pi \e, x)$,

• $d_E(\e, \e') = d_E(\e, \e_x) + d_E(\e_x, \e')$ for any $\e' \in \pi^{-1}x$.

We denote the category of metric fibrations over $X$ by $\mathsf{Fib}_X$. The morphisms are base and fiber preserving Lipschitz maps.

A remarkable property of the metric fibration is that the magnitude behaves like the Euler characteristic of topological fiber bundles as follows.

Proposition (Leinster) Let $\pi : E \longrightarrow X$ be a metric fibration. If $E$ is a finite metric space, then its magnitude is a product of those of $X$ and $\pi^{-1}x$ for any $x \in X$.

We note that fibers of $\pi$ are isometric.

Examples

• For a product of metric spaces $E = X\times Y$, the projection $X\times Y \longrightarrow X$ is a metric fibration.

• The projection from the complete bipartite graph $K_{3, 3}$ to $K_3$ is a metric fibration. Hence the magnitude of $K_{3,3}$ is same as that of $K_3 \times K_2$ as pointed out by Leinster.

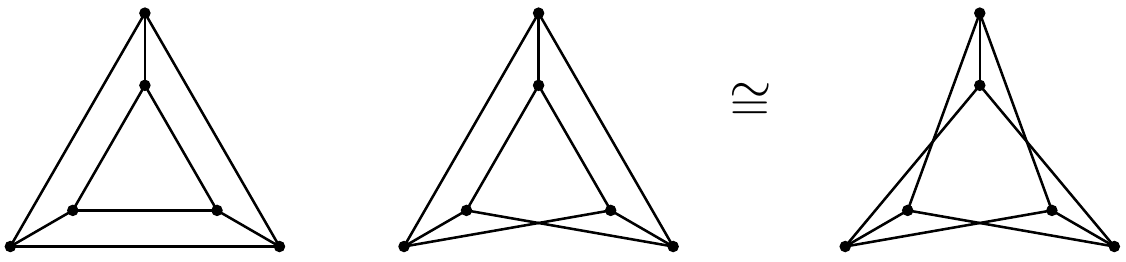
The graph on the left is $K_3\times K_2$, and the graph on the right is isomorphic to $K_{3, 3}$. They both have the magnitude $\frac{6}{1 + 3q + 2q^2}$.

Metric fibrations as Grothendieck fibrations

As is well known to category theorists, there is a notion of fibrations for small categories called Grothendieck fibrations, of which we don’t explain the definition here. This notion is equivalent to lax functors via the so-called Grothendieck construction, and we can generalize this story in $\mathsf{Fset}$-enriched setting. What is remarkable is that the restriction of such a generalized Grothendieck fibration to $\mathsf{Met}$ (the category of metric spaces and Lipschitz maps) is exactly the metric fibration. We define the metric counterpart of lax functors as follows.

Definition  Let $X$ be a metric space.

• A metric action $F : X \longrightarrow \mathsf{Met}$ consists of metric spaces $F x \in \mathsf{Met}$ for any $x \in X$ and isometries $F_{x x'} : F x \longrightarrow F x'$ for any $x, x' \in X$ satisfying the following for any $x, x', x'' \in X$:

  • $F_{x x} = {\mathrm{id}}_{F x}$ and $F_{x'x} = F_{x x'}^{-1}$,
  • $d_{F x''}(F_{x'x''}F_{x x'}a, F_{x x''}a) \leq d_X(x, x') + d_X(x', x'') - d_X(x, x'')$ for any $a \in F x$.

• A metric transformation $\theta : F \Rightarrow G$ consists of Lipschitz maps $\theta_x : F x \longrightarrow G x$ for any $x \in X$ satisfying that $G_{x x'}\theta_x = \theta_{x'}F_{x x'}$ for any $x, x' \in X$. We can define the composite of metric transformations $\theta$ and $\theta'$ by $(\theta'\theta)_x = \theta'_x\theta_x$. We denote the category of metric actions $X \longrightarrow \mathsf{Met}$ and metric transformations by $\mathsf{Met}_X$.

The equivalence of Grothendieck fibrations and lax functors is generalized to $\mathsf{Fset}$-enriched situation and restricted to $\mathsf{Met}$ as follows.

Theorem   The Grothendieck construction gives a category equivalence $$\mathsf{Met}_X \simeq \mathsf{Fib}_X.$$

Classification of metric fibrations

Now we give a complete classification of metric fibrations by several means, which is parallel to that of topological fiber bundles. Namely, we define “principal fibrations”, “fundamental groups” and a “$1$-Čech cohomology” for metric spaces, and obtain equivalences between categories of these objects. Roughly speaking, we obtain an analogy of the following correspondence in the case of topological fiber bundles with a discrete structure group.

Fiber bundles over $X$ with structure group $G$

$\leftrightarrow$ Principal $G$-bundles over $X$ ($G$-torsors)

$\leftrightarrow$ $[X, B G] \cong \mathsf{Hom}(\pi_1(X), G)/conjugation$

$\leftrightarrow$ $\mathsf{H}^1(X, G)$

We explain these correspondences in the following, and we start from the definition of torsors in $\mathsf{Met}$.

$\mathcal{G}$-torsors

We can define a subcategory $\mathsf{Tors}_X^{\mathcal{G}}$ of $\mathsf{Fib}_X$ that consists of “principal $\mathcal{G}$-fibrations” called $\mathcal{G}$-torsors. Here and in the following, a group $\mathcal{G}$ is not just a group but is a group object of $\mathsf{Met}$, which we call a metric group. Namely, it is a metric space as well as a group whose operations are all Lipschitz maps.

Definition   Let $G$ be a group (not a metric one). A metric fibration $\pi : E \longrightarrow X$ is a $G$-torsor over $X$ if it satisfies the following:

• $G$ acts isometrically on $E$ from the right, and preserves each fiber of $\pi$.

• Each fiber of $\pi$ is a right $G$-torsor in the following sense. Let $G$ be a group and $Y$ be a metric space. We say that $Y$ is a right $G$-torsor if $G$ acts on $Y$ from the right and satisfies the following :

  • It is free and transitive.
  • $g : Y \longrightarrow Y$ is an isometry for any $g \in G$.
  • We have $d_Y(y, yg) = d_Y(y', y'g)$ for any $y, y' \in Y$ and $g \in G$.

For a $G$-torsor $\pi : E \longrightarrow X$, we can equip $G$ with a metric group structure that is isometric to any fiber of $\pi$. Hence, in the following, we write “$\mathcal{G}$-torsors” instead of “$G$-torsors”, where $\mathcal{G}$ is such a metric group obtained from $G$.

A $\mathcal{G}$-morphism between $\mathcal{G}$-torsors is a $G$-equivariant map that is also a morphism of metric fibrations. We denote the category of $\mathcal{G}$-torsors over $X$ and $\mathcal{G}$-morphisms by $\mathsf{Tors}_X^{\mathcal{G}}$. Note that the category $\mathsf{Tors}_X^{\mathcal{G}}$ is a subgroupoid of $\mathsf{Fib}_X$.

On the other hand, we can also define a subcategory $\mathsf{PMet}_X^{\mathcal{G}}$ of $\mathsf{Met}_X^{\mathcal{G}}$ that is the counterpart of $\mathsf{Tors}_X^{\mathcal{G}}$.

Definition   Let $X$ be a metric space and $\mathcal{G}$ be a metric group.

• A $\mathcal{G}$-metric action $F : X \longrightarrow \mathsf{Met}$ is a metric action satisfying the following:

  • $F_x = \mathcal{G}$ for any $x \in X$.
  • $F_{x x'}$ is a left multiplication by some $f_{x x'} \in \mathcal{G}$ for any $x, x' \in X$.

• Let $F, G : X \longrightarrow \mathsf{Met}$ be $\mathcal{G}$-metric actions. A $\mathcal{G}$-metric transformation $\theta : F \Rightarrow G$ is a metric transformation such that each component $\theta_x : F x \longrightarrow G x$ is a left multiplication by an element $\theta_x \in \mathcal{G}$. We denote the category of $\mathcal{G}$-metric actions $X \longrightarrow \mathsf{Met}$ and $\mathcal{G}$-metric transformations by $\mathsf{PMet}_X^{\mathcal{G}}$.

Apparently, $\mathsf{PMet}_X^{\mathcal{G}}$ is a subcategory of $\mathsf{Met}_X$ and is also a groupoid. Then we have the following.

Theorem   The Grothendieck construction gives a category equivalence $$\mathsf{PMet}_X^\mathcal{G} \simeq \mathsf{Tors}_X^\mathcal{G}.$$

Fundamental metric group

As an example of a metric group, we construct the fundamental metric group $\pi_1^m(X, x)$ of a metric space $X$. Roughly speaking, it is a collection of “loops in $X$” based at some $x \in X$, which are identified by a “homotopy relation”. In precise terms, we define it as follows.

Definition   Let $X$ be a metric space and $x \in X$.

• For each $n \geq 0$, we define a set $P_n(X, x)$ by $$P_n(X, x) := \{(x, x_1, \dots, x_n, x) \in X^{n+2}\}.$$ We also define that $P(X, x) := \bigcup_n P_n(X, x)$.

• We define a connected graph $G(X, x)$ with the vertex set $P(X, x)$ as follows. For $u, v \in P(X, x)$, an unordered pair $\{u, v\}$ spans an edge if and only if it satisfies both of the following :

  • There is an $n \geq 0$ such that $u \in P_n(X, x)$ and $v \in P_{n+1}(X, x)$.
  • There is a $0 \leq j \leq n$ such that $u_i = v_i$ for $1 \leq i \leq j$ and $u_i = v_{i+1}$ for $j+1 \leq i \leq n$, where we have $u = (x, u_1, \dots, u_n, x)$ and $v = (x, v_1, \dots, v_{n+1}, x)$.

• We equip the graph $G(X, x)$ with a weighted graph structure by defining a function $w_{G(X, x)}$ on edges by
  $$w_{G(X, x)}\{u, v\} = \begin{cases} d_X(v_j, v_{j+1}) + d_X(v_{j+1}, v_{j+2}) - d_X(v_j, v_{j+2}) & v_j\neq v_{j+2}, \\ 0 & v_j = v_{j+2}, \end{cases}$$ where we use the notation $v_j$ as in the previous bullet point.

• We denote the quasi-metric space obtained from the weighted graph $G(X, x)$ by $Q(X, x)$. Let $\pi_1^{m}(X, x)$ be the metric space obtained from $Q(X, x)$ by identifying points with distance $0$. The metric space $\pi^m_1(X, x)$ has a metric group structure given by the concatenation. We call the metric group $\pi_1^m(X, x)$ the fundamental metric group of $X$ with the base point $x$.

We sometimes write $\pi_1^m(X)$ since it does not depend on the choice of the base point, similarly to the topological case. We also define a category $\mathsf{Hom}(\pi_1^m(X), \mathcal{G})$ of homomorphisms $\pi_1^m(X) \longrightarrow \mathcal{G}$, where a morphism between homomorphisms is a conjugation relation. Then we have the following.

Theorem   We have a category equivalence $$\mathsf{Hom} (\pi^m_1(X), \mathcal{G}) \simeq \mathsf{PMet}^{\mathcal{G}}_X.$$

As an example, we classify $\mathcal{G}$-torsors over cycle graphs as follows. We note that the notion of a metric group is equivalent to that of a “normed group” as E. Roff stated in her thesis. For a metric group $\mathcal{G}$, we denote the corresponding norm of an element $g \in \mathcal{G}$ by $|g| \in \mathbb{Z}_{\geq 0}$.

Proposition  Let $C_n$ be an $n$-cycle graph. Then we have $$\pi^m_1(C_n) \cong \begin{cases}\mathbb{Z}  \text{ with }  |1| = 1 & n :  \text{odd}, \\ 0 & n :  \text{even}. \end{cases}$$ Hence we have that $$\mathsf{PMet}_{C_n}^{\mathcal{G}} \simeq \begin{cases}\mathsf{Hom} (\mathbb{Z}, \mathcal{G}) & n :  \text{odd}, \\ 0 & n :  \text{even}, \end{cases}$$ for any metric group $\mathcal{G}$, which implies that there is only a trivial metric fibration over $C_{2n}$ and that there is at most one non-trivial metric fibration over $C_{2n+1}$.

Associated bundle construction and structure groups

Now, similarly to the topological case, we can define an “associated bundle construction” from a torsor and a metric space $Y$.

First we give another example of a metric group. For a metric space $Y$, let $\mathsf{Aut} Y$ be the group of self-isometries on $Y$. We define a distance function on $\mathsf{Aut} Y$ by

$$d_{\mathsf{Aut} Y}(f, g) = \sup_{y\in Y} d_Y(fy, gy).$$

Then $(\mathsf{Aut} Y, d_{\mathsf{Aut} Y})$ is a metric group if $Y$ is bounded. Even if not, it is an extended metric group that admits $\infty$ as a distance. We suppose that $\mathsf{Aut} Y$ is a metric group in the following, but the discussions can be applied to the extended case.

Now we can construct a metric fibration with fiber $Y$ from a $\mathsf{Aut} Y$-torsor similarly to the topological case. This construction gives the following.

Theorem  Suppose that $Y$ is a bounded metric space. Then we have a category equivalence $$\mathsf{PMet}_X^{\mathsf{Aut} Y} \simeq {\mathsf{core}}\mathsf{Fib}_X^Y,$$ where $\mathsf{Fib}_X^Y$ is the full subcategory of $\mathsf{Fib}_X$ that consists of metric fibrations with the fiber $Y$, and we denote the core of a category by $\mathsf{core}$.

Čech cohomology of $\mathcal{G}$-torsors

For a $\mathcal{G}$-torsor $X$, we define a “$1$-Čech cohomology” $\mathsf{H}^1(X, \mathcal{G})$ as a groupoid. This is an analogy from the Čech cohomology constructed from the local sections of a principal bundle. We first define the “local section” of a $\mathcal{G}$-torsor.

Definition  Let $\pi : E \longrightarrow X$ be a $\mathcal{G}$-torsor. For $x_i, x_j \in X$, we define a local section of $\pi$ over a pair $(x_i, x_j)$ as a pair of points $(\varepsilon_i, \varepsilon_j) \in E^2$ such that $\pi \e_i = x_i, \pi \e_j = x_j$ and $\varepsilon_j$ is the lift of $x_j$ along $\varepsilon_i$.

We say that $((\varepsilon^{ij}_i,\varepsilon^{ij}_j))_{(i, j)\in I^2}$ is a local section of $\pi$ if each $(\varepsilon^{ij}_i, \varepsilon^{ij}_j)$ is a local section of $\pi$ over a pair $(x_i, x_j)$ and satisfies that $\varepsilon^{ij}_i = \varepsilon^{ji}_i$.

Now we would like to define a cocycle from the differences of any pair of adjacent local sections. Before that, we define the Čech cohomology in general.

Definition  Let $X$ be a metric space and suppose that points of $X$ are indexed as $X = \{x_i\}_{i \in I}$. For a metric group $\mathcal{G}$, we define the $1$-cohomology of $X$ with coefficients in $\mathcal{G}$ as the category $\mathsf{H}^1(X; \mathcal{G})$ by $$\mathrm{Ob}\mathsf{H}^1(X; \mathcal{G}) = \left\{(a_{ijk}) \in \mathcal{G}^{I^3} \mid a_{ijk}a_{kj\ell } = a_{ij\ell}, |a_{ijk}a_{jki}a_{kij}| \leq |\Delta(x_i, x_j, x_k)|\right\},$$ and $$\mathsf{H}^1(X; \mathcal{G})((a_{ijk}), (b_{ijk})) = \left\{(f_{ij}) \in \mathcal{G}^{I^2} \mid a_{ijk}f_{jk} = f_{ij}b_{ijk} \right\},$$ where we denote the conjugation-invariant norm on $\mathcal{G}$ by $|-|$. In the above, we used the notation $|\Delta(x_1, x_2, x_3)|$ for $x_1, x_2, x_3 \in X$ defined as $$|\Delta(x_1, x_2, x_3)| := \min \left\{d_X(x_i, x_j) + d_X(x_j, x_k) - d_X(x_i, x_k) \mid \{i, j, k\} = \{1, 2, 3\}\right\}.$$ We call an object of $\mathsf{H}^1(X; \mathcal{G})$ a cocycle. The category $\mathsf{H}^1(X; \mathcal{G})$ is in fact a groupoid.

Proposition  Let $\pi : E \longrightarrow X$ be a $\mathcal{G}$-torsor. For a local section $s =((\varepsilon^{ij}_i,\varepsilon^{ij}_j))_{(i, j)\in I^2}$ of $\pi$, we can construct a cocycle $\alpha_s \pi \in \mathrm{Ob} \mathsf{H}^1(X;\mathcal{G})$. Further, for any two local sections $s, s'$ of $\pi$, the corresponding cocycles $\alpha_s \pi$ and $\alpha_{s'} \pi$ are isomorphic.

Conversely we can construct a $\mathcal{G}$-torsor from a cocyle by pasting copies of $\mathcal{G}$’s along the cocycle. Then we have the following from these correspondences.

Theorem   We have a category equivalence $$\mathsf{H}^1(X; \mathcal{G}) \simeq \mathsf{Tors}^{\mathcal{G}}_X.$$