This Week’s Finds in Mathematical Physics (Week 270)
Posted by John Baez
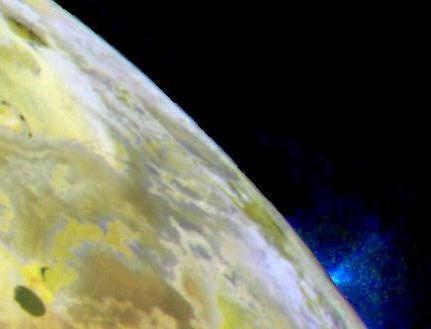
In week270 of This Week’s Finds, see lava flowing on Jupiter’s moon Io.
Hear about Greg
Egan’s brand new novel, Incandescence. Then - see talks about
my favorite three numbers. Read about the Mathieu group M12.
Learn how each regular polytope with 5-fold rotational symmetry is
secretly linked to a lattice in a space of twice as many dimensions.
And see a simple but surprising property of the number 12.

Connes-Egan; Re: This Week’s Finds in Mathematical Physics (Week 270)
Week 270 = 2 * 3^3 * 5 is delightful. Even through the stench of brimstone…
Suppose that physical space is actually a noncommutative geometry. What primitive aliens in an exotic environment could figure that out? I await the Connes-Egan collaboration…