Line Bundles on Complex Tori (Part 4)
Posted by John Baez
Last time I introduced a 2-dimensional complex variety called the Eisenstein surface
where is the lattice of Eisenstein integers. We worked out the Néron–Severi group of this surface: that is, the group of equivalence classes of holomorphic line bundles on this surface, where we count two as equivalent if they’re isomorphic as topological line bundles. And we got a nice answer:
where consists of hermitian matrices with Eisenstein integers as entries.
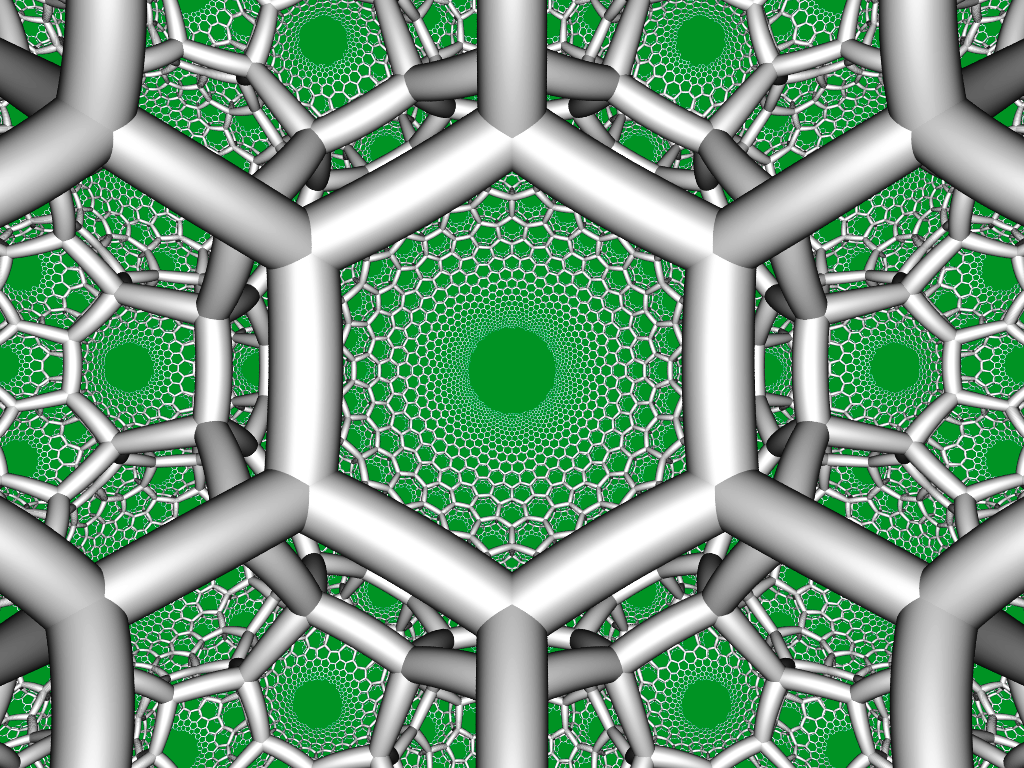
Now we’ll see how this is related to the ‘hexagonal tiling honeycomb’:
We’ll see an explicit bijection between so-called ‘principal polarizations’ of the Eisenstein surface and the centers of hexagons in this picture! We won’t prove it works — I hope to do that later. But we’ll get everything set up.
The hexagonal tiling honeycomb
This picture by Roice Nelson shows a remarkable structure: the hexagonal tiling honeycomb.
What is it? Roughly speaking, a honeycomb is a way of filling 3d space with polyhedra. The most symmetrical honeycombs are the ‘regular’ ones. For any honeycomb, we define a flag to be a chosen vertex lying on a chosen edge lying on a chosen face lying on a chosen polyhedron. A honeycomb is regular if its geometrical symmetries act transitively on flags.
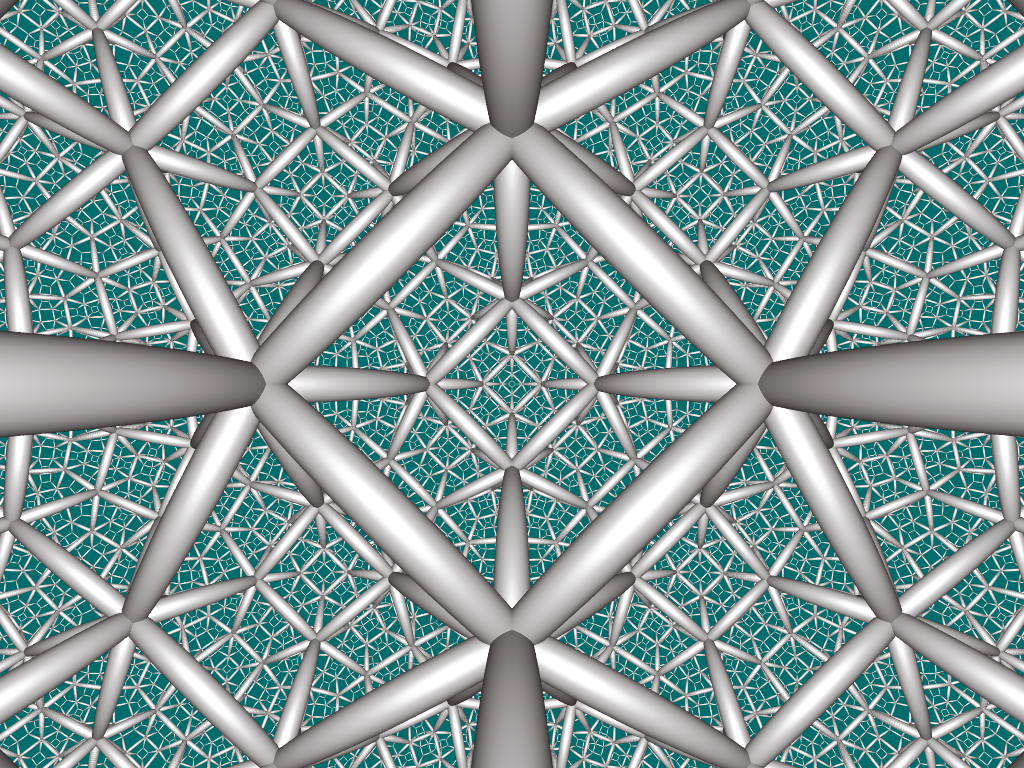
The most familiar regular honeycomb is the usual way of filling Euclidean space with cubes. This cubic honeycomb is denoted by the symbol , because a square has 4 edges, 3 squares meet at each corner of a cube, and 4 cubes meet along each edge of this honeycomb. We can also define regular honeycombs in hyperbolic space. For example, the order-5 cubic honeycomb is a hyperbolic honeycomb denoted , since 5 cubes meet along each edge:
Coxeter showed there are 15 regular hyperbolic honeycombs. The hexagonal tiling honeycomb is one of these. But it does not contain polyhedra of the usual sort! Instead, it contains flat Euclidean planes embedded in hyperbolic space, each plane containing the vertices of infinitely many regular hexagons. You can think of such a sheet of hexagons as a generalized polyhedron with infinitely many faces. You can see a bunch of such sheets in the picture:
The symbol for the hexagonal tiling honeycomb is , because a hexagon has 6 edges, 3 hexagons meet at each corner in a plane tiled by regular hexagons, and 3 such planes meet along each edge of this honeycomb. You can see that too if you look carefully.
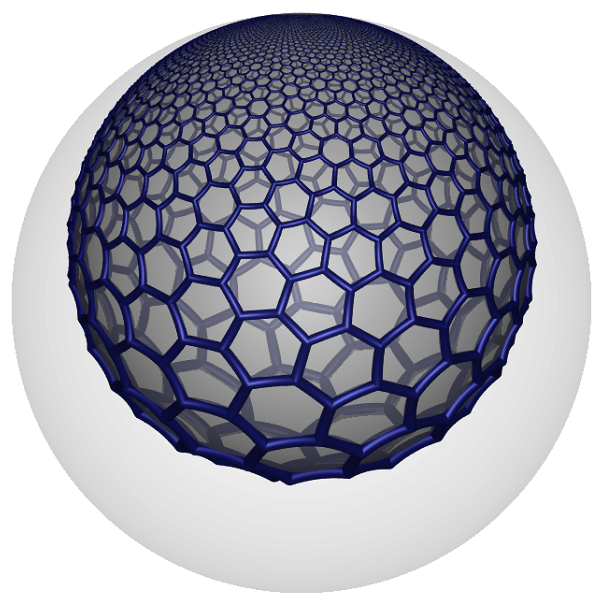
A flat Euclidean plane in hyperbolic space is called a horosphere. Here’s a picture of a horosphere tiled with regular hexagons, yet again drawn by Roice:
Unlike the previous pictures, which are views from inside hyperbolic space, this uses the Poincaré ball model of hyperbolic space. As you can see here, a horosphere is a limiting case of a sphere in hyperbolic space, where one point of the sphere has become a ‘point at infinity’.
Be careful. A horosphere is intrinsically flat, so if you draw regular hexagons on it their internal angles are
as usual in Euclidean geometry. But a horosphere is not ‘totally geodesic’: straight lines in the horosphere are not geodesics in hyperbolic space! Thus, a hexagon in hyperbolic space with the same vertices as one of the hexagons in the horosphere actually bulges out from the horosphere a bit — and its internal angles are less than : they are
It’s really these hexagons in hyperbolic space that are faces of the hexagonal tiling honeycomb, not those tiling the horospheres, though perhaps you can barely see the difference. This can be quite confusing until you think about a simpler example, like the difference between a cube in Euclidean 3-space and a cube drawn on a sphere in Euclidean space.
Connection to special relativity
Johnson and Weiss have studied the symmetry group of the hexagonal tiling honeycomb:
- Norman W. Johnson and Asia Ivic Weiss, Quadratic integers and Coxeter groups, Canad. J.Math. 51 (1999), 1307–1336.
They describe this group using the ring of Eisenstein integers:
where is the cube root of unity . And I believe their work implies this result:
Theorem. The orientation-preserving symmetries of the hexagonal tiling honeycomb form the group .
I’ll sketch a proof later, starting from what they actually show.
For comparison, the group of all orientation-preserving symmetries of hyperbolic space forms the larger group . This group is the same as … and this naturally brings Minkowski spacetime into the picture!
You see, in special relativity, Minkowski spacetime is equipped with the nondegenerate bilinear form
usually called the Minkowski metric.
Hyperbolic space sits inside Minowski spacetime as the hyperboloid of points with and . Equivalently, we can think of Minkowski spacetime as the space of hermitian complex matrices, using the fact that every such matrix is of the form
and
One reason this viewpoint is productive is that the group of symmetries of Minkowski spacetime that preserve the orientation and also preserve the distinction between future and past is the projective special linear group . The idea here is that any element acts on by
This action clearly preserves the Minkowski metric (since it preserves the determinant of ) and also the orientation and the direction of time (because is connected). However, multiples of the identity matrix, namely the matrices , act trivially. So, we get an action of the quotient group .
In these terms, the future cone in Minkowski spacetime is the cone of positive definite hermitian matrices:
Sitting inside this we have the hyperboloid
which is none other than hyperbolic space! The Minkowski metric on induces the usual Riemannian metric on hyperbolic space (up to a change of sign).
Indeed, not only is the symmetry group of the hexagonal tiling honeycomb abstractly isomorphic to the subgroup
we’ve also seen this subgroup acts as orientation-preserving isometries of hyperbolic space. So it seems almost obvious that acts on hyperbolic space so as to preserve some hexagonal tiling honeycomb!
Constructing the hexagonal tiling honeycomb
Thus, the big question is: how can we actually construct a hexagonal tiling honeycomb inside that is preserved by the action of ? I want to answer this question.
Sitting in the complex numbers we have the ring of Eisenstein integers. This lets us define a lattice in Minkowski spacetime, called , consisting of hermitian matrices with entries that are Eisenstein integers. James Dolan and I conjectured this:
Conjecture. The points in the lattice that lie on the hyperboloid are the centers of hexagons in a hexagonal tiling honeycomb.
I think Greg Egan has done enough work to make this clear. I will try to write up proof here next time. Once this is done, it should be fairly easy to construct the other features of the hexagonal tiling honeycomb. They should all be related in various ways to the lattice .
Connection to the Néron–Severi group of the Eisenstein surface
But what does any of this have to do with the supposed theme of these blog posts: line bundles on complex tori? To answer this, we need to remember that last time I gave an explicit isomorphism between
- the Néron–Severi group of the Eisenstein surface
and
- (viewed as an additive group).
This isomorphism wasn’t new: experts know a lot about it. For example, under this correspondence, elements with and correspond to elements of the Néron–Severi group coming from ample line bundles. Elements of the Néron–Severi group coming from ample line bundles are called polarizations.
Furthermore, elements with with and are known to correspond to certain specially nice polarizations called ‘principal’ polarizations.
So, the conjecture implies this:
Main Result. There is an explicit bijection between principal polarizations of the Eisenstein surface and centers of hexagons in the hexagonal tiling honeycomb.
There’s a lot more to say, but I’ll stop here for now, at least after filling in some details that I owe you.
Symmetries of the hexagonal tiling honeycomb
As shown already by Coxeter, the group of all isometries of hyperbolic space mapping the hexagonal tiling honeycomb to itself is the Coxeter group . That means this group has a presentation with generators and relations
Each generator corresponds to a reflection in hyperbolic space — that is, an orientation-reversing transformation that preserves angles and distances. The products of pairs of generators are orientation preserving, and they generate an index-2 subgroup of called .
Johnson and Weiss describe the group using the Eisenstein integers. Namely, they show it’s isomorphic to the group .
But what the heck is that?
As usual, is the group of matrices with entries in having determinant . But Johnson and Weiss define a slightly larger group to consist of all matrices with entries in that have determinant with absolute value . This in turn has a subgroup consisting of multiples of the identity where has absolute value . Then they define .
What does the group actually amount to? Since all the units in have absolute value — they’re just the 6th roots of unity — is the same as the group consisting of all invertible matrices with entries in . The subgroup consists of matrices where is a 6th root of unity. If I’m not confused, this is just the center of . So what they’re calling is modulo its center. This is usually called .
All this is a bit confusing, but I think that with massive help from Johnson and Weiss we’ve shown this:
Theorem. The orientation-preserving symmetries of the hexagonal tiling honeycomb form the group .
The interplay between and will become clearer next time: the latter group contains some 60 degree rotations that the former group does not!