August 31, 2007
Axis of Evil? Or Axis of Opportunity?
Posted by John Baez
This paper argues that the axes of elliptical galaxies don’t point in random directions. but have a slight tendency to point roughly towards the ‘axis of evil’. The ‘axis of evil’ is a controversial concept in itself: a direction that seems to be picked out by anisotropies in the cosmic microwave background!
Trying to put a more positive spin on the idea, the author calls it the ‘axis of opportunity’:
- Michael J. Longo, The axis of opportunity: the large-scale correlation of elliptical galaxies.
I thank Daniel Rocha for pointing out this paper to me.
August 30, 2007
On Hess and Lack on Bundles of Categories
Posted by Urs Schreiber
Kathryn Hess and Steve Lack are working on a Bundle Theory for Categories, various aspects of which are very close to the things we like to talk about here at the -Café.
John Baez kindly pointed out to me the very nice set of slides
Kathryn Hess
Bundle Theory for Categories
(slides) .
These slides discuss definitions and examples of this framework from 0-categories over 1-categories to 2-categories.
Here I shall walk through the material of that talk concerning 1-categories by exemplifying every step in terms of the example where the bundle of categories in question is the Atiyah groupoid of an (ordinary!) principal -bundle.
I believe this is helpful for putting these constructions in perspective.
I shall make use of the discussion of the Atiyah groupoid as given in
-Transport and Higher Schreier Theory
and
Curvature, the Atiyah Sequence and Inner Automorphisms,
but I try to make the discussion self-contained and elementary.
Question about von Neumann Algebras
Posted by Urs Schreiber
Jim Stasheff asks me to share this quote:
Study is hard work. It is so much easier to find something else to do in its place than to stay at the grind of it. We have excuses aplenty for avoiding the dull, hard, daily attempt to learn. There is always something so much more important to do than reading. There is always some excuse for not stretching our souls with new ideas and insights now or yet or ever.
by Joan Chittister
Quoted in Essential Monastic Wisdom, by Hugh Feiss .
And another email I receive reminds me of the truth of this. Somebody writes
In one of your entries in the -category Café blog, you raised a question that is very relevant to what I’m doing. Did you settle the question in the end as to whether all bimodules over von Neumann algebras really do for sure come from homomorphisms? Do you have any suggestions for what I can read to find out?
This reminds me of my feeble attempts to learn von Neumann algebra theory (was it here?), and how I already start forgetting what I did learn. I think the above statement, that all bimodules in fact come from algebra homomorphisms, is at least true for type III factors.
Somebody please help. Me, and, probably more importantly, the person who wrote the above message.
August 29, 2007
Journal Publishers Hire the “Pit Bull of PR”
Posted by John Baez
Ever hear about PRISM? It’s a publisher’s group — backed by Elsevier and others — that’s leading the fight against open access to scholarly publications.
To plan their strategy, PRISM hired none other than Eric Dezenhall:
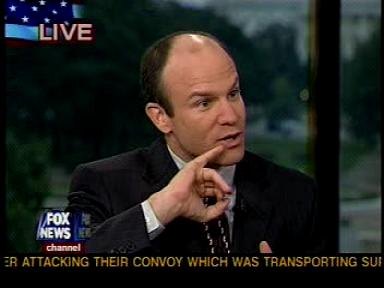
This is the guy who BusinessWeek called the “pit bull of PR”. The guy whose firm Bill Moyers called “the Mafia of industry”. The guy who wrote:
Damage control used to be about soft, fuzzy concepts like image. Now it’s about survival, and this has made the battle bloodier.
And, guess what he advised the publishers to do!
August 28, 2007
Arrow-Theoretic Differential Theory III: Higher Morphisms
Posted by Urs Schreiber
In Arrow-Theoretic Differential Theory, Part II I argued that we need to pass to tangent categories in order to understand, not just -curvature, but also higher morphisms of Lie -algebras.
In Vienna John Baez quizzed me about these ideas, thereby leading me to the more refined insight which I mentioned in More on Tangent Categories.
This insight, which involved making explicit a fact and a construction which I had already been using in a way but not truly realized myself, I have now started to seriously incorporate while revising the provisional Structure of Lie -algebras.
Since it all seems to work out quite nicely – unless I am making some dumb mistake – here is an update on that matter:
Higher Morphisms of Lie -Algebras from Arrow-Theoretic Differential Theory
Abstract.
We would like to achieve a good explicit understanding of higher morphisms of Lie -algebras. We notice that various formerly puzzling aspects of this seem to become clearer as one passes from Lie -algebras to their Lie -algebras of inner derivations in a certain way. Using this, we define higher morphisms of Lie -algebras explicitly and in general. These should constitute an -category. While we fall short of verifying this in full generality, we do obtain the Baez-Crans 2-category of Lie 2-algebras [1] in the special case where we restrict everything to Lie 2-algebras.
August 27, 2007
This Week’s Finds in Mathematical Physics (Week 256)
Posted by John Baez
In week256, learn a bit of what happened at a conference on Poisson sigma models and Lie algebroids at the Erwin Schrödinger Institute, run by Anton Alekseev, Henrique Bursztyn and Thomas Strobl. Higher categories are finding their way into classical mechanics! Then, hear more of the Tale of Groupoidification: how to turn a span of groupoids into an operator between vector spaces.
August 26, 2007
The Reasoner
Posted by David Corfield
My past and future colleague Jon Williamson started a monthly digest of research on reasoning - The Reasoner. I was asked to guest edit the August edition, which required me to write an editorial and to interview someone of my choice. I opted for Brendan Larvor, a philosopher with very close interests to my own. You can read these items here.
August 25, 2007
The G and the B
Posted by Urs Schreiber
We have a pretty good understanding of what the “-field” in string theory really is, in terms of arrow-theory.
This nicely explains a bunch of things. I used to be very annoyed with myself, though, for not understanding, on this nice abstract level, one of the more intriguing aspects of the -field:
beyond its mere nature as being the 2-categorical version of a line bundle with connection, it turns out that the connection 2-form here unifies in an intriguing way with a Riemannian metric (and with a dilaton field, in fact).
Both these rank-2 tensors and sum up to an object which is known as the “open string metric” to string theorists, and which happens to have a surprisingly nice and natural geometrical interpretation in the context of what is called generalized complex geometry.
In this approach, due to Nigel Hitchin, one studies the geometry of manifolds all in terms of the sum of their tangent and cotangent bundle making use of various kinds of useful natural structures present on this bundle, like its canonical bilinear pairing as well as the Courant bracket.
As is indicated to some extent in section 3.8 of
Marco Gualtieri
Generalized Complex Geometry
math.DG/0401221
this bundle is to be thought of as the Atiyah Lie 2-algebroid of an abelian gerbe on , hence as the infinitesimal version of something like the 2-groupoid of automorphisms of the corresponding 2-bundle.
The more or less obvious -algebroid structures on should corespond to the Atiyah Lie -algebroids of --bundles.
While I do follow all the algebra underlying generalized complex geometry, I had always said to myself that at some point I should better understand what all this really means. Given the available nice categorical picture of line 2-bundles, there should be a nice arrow-theoretic integrated version of understanding all this, showing how a line 2-bundle with connection knows something about Riemannian structures.
Unfortunately, I had never really taken the time to think this through.
Now, today Marco Gualtieri approached me with a question. Since he is already happy with having unified the 2-form connection with the Riemannian metric in his formalism, he was trying to get hints for how all the other string background fields would fit into this mathematical picture, like the RR-forms and in particular the dilaton.
This made me really angry with myself. I wished I had figured out at least some of the answers to these obviously open and crucial questions before he asked me! I knew these needed to be answered at some point.
As it goes, this kind of frustration sometimes is the best motivatin to get going. While S. Merkulov was teaching us this morning about operads, Poisson structures and graph complexes, I believe I made some progress with understanding what’s going on.
The key is, it seems, to first figure out what the Atiyah -groupoid of an -bundle really is. I’ll discuss this using tangent -categories and flows on -categories, as described in Arrow-theoretic differential geometry.
I shall try to indicate what I mean by that, and how it gives rise to the appearance of the structures we see in generalized complex geometry.
August 24, 2007
That Shift in Dimension
Posted by Urs Schreiber
John Baez and I spent the evening in Café Einstein in Vienna (remember, we are at that conference), drinking beer and talking about Stokes’ theorem, natural -transformations and the holographic principle, and how it is all the same thing, really.
Well, okay, I admit we didn’t quite finish proving that theorem, or even making that statement precise. But we had those printouts with us, and after sufficiently many beers, we very much enjoyed figuring out some simple underlying principle of the – at first sight apparently mind bogglingly weird – statement of
Maxim Kontsevich
Deformation quantization of Poisson manifolds, I
q-alg/9709040,
which says that any classical kinematics is canonically quantizable by some strange formula which involves lots of sums over lots of diagrams…
…and the maybe even more surprising explanation of this formula (which Kontsevich apparently knew but didn’t bother to talk about) in terms of correlators of a topological two-dimensional theory of quantum fields with values in the original phase space (hence something that is everywhere of one dimension higher than one would think it should be) as given in
Alberto S. Cattaneo, Giovanni Felder
A path integral approach to the Kontsevich quantization formula
math/9902090 .
John went to bed, while I carefully carried our little insight back to the institute, in my bare hands, so as to talk about it here. The following is supposed to, in turn, help explain, in elementary terms understandable by anyone who understands all or either of
- high school quantum mechanics
- Stokes’ theorem
why on earth the quantization of an -dimensional theory may be obtained by a topological -dimensional field theory.
This is meant for those who enjoy things like Kindergarten Quantum Mechanics. If you are not among these, don’t bother continue reading.
August 23, 2007
Wilson Loop Defects on the String
Posted by Urs Schreiber
Just heard a very interesting talk by Samuel Monnier on his work
A. Alekseev, S. Monnier
Quantization of Wilson Loops in Wess Zumino Witten models
hep-th/0702174 .
August 22, 2007
Lyakhovich and Sharapov on QFT (On BV-Quantization, Part III)
Posted by Urs Schreiber
Today at ESI in Vienna, S. Lyakhovich kindly pointed me to lots of his work. While most everybody was gone hiking, I spent the afternoon reading his articles.
These all develop two main threads:
A) A very clear-sighted description of classical and quantum, Lagrangian and Hamiltonian, gauge and constrained mechanics, closely related to, but going beyond, BV-formalism. Lyakhovich gives the nicest description of the BRST operator which I remember having seen.
B) Holography. While Lyakhovich doesn’t mention that word, he does discuss the underlying issues. In particular, this work does provide some nice insights into the relation between Chern-Simons theory in -dimensions and the coresponding theories on the -dimensional boundary.
I’d expect that various aspects of this body of work will give the impression of familiarity to various experts. But I am struck by the clarity with wich these concepts are understood and ordered to a coherent whole.
Among the many talks we had related to BV-AKSZ and holographic phenomena, all apparently pointing to a deeper story waiting at our fingertips to be fully unraveled, the one on this work stands out as having the clear intent not only to plough through labyrinths of impressive formulas, but to actually increase the level of conceptual understanding.
Gerbes in The Guardian
Posted by John Baez
In his post on Future Gazing, David Corfield invites us to predict the future of -categories over the next year — the second year of the n-Café. I’m seeing lots of signs that -categories are catching on — but it could be happening even faster than I expected. Here’s one article I would not have thought to see in an important British newspaper:
- Marc Abraham, Beyond definition: when I say gerbe I don’t mean grebe, The Guardian, Education Guardian Weekly, August 21, 2007.
Thanks go to Eugenia Cheng for pointing this one out!
Justificatory Narratives
Posted by David Corfield
Eighteen months ago, back at my old blog, I discussed a paper by Robert W. Batterman, On the Specialness of Special Functions (The Nonrandom Effusions of the Divine Mathematician). It has now appeared in the latest edition of the British journal of the Philosophy of Science, so I’ll copy the post to the Café. As you will see, what bothers me is a readiness to delineate sharply justificatory narratives of pieces of mathematics into two classes: ‘mathematics’ and ‘physics’. Also, I find it unfair that we get to hear much more up-to-data accounts from the physics side than from the mathematics side.
August 21, 2007
More on Tangent Categories
Posted by Urs Schreiber
I am the luckiest man in the world, having John Baez around here at ESI in Vienna to talk to – over breakfast, over lunch, and in between – about -curvature and that stuf which I like to think of as tangent categories and arrow-theoretic differential theory.
At the same time, the dg-wizards like Dmitry Roytenberg are around, and using that bridge which connects -categorical algebra with physicist’s BRST-BV formalism I can connect these two huge reservoirs of ideas and let the information flow botrh ways. That helps a lot.
Here are some insights which I gained by
a) trying to address questions John urged me to answer more properly, and by all the input I get from him;
b) comparing this to what the dg-BV people are doing by passing back and forth over that bridge.
More concretely, I shall
A) point out in detail how the notion of forming the “tangent category” which I was talking about coincides, in the appropriate sense and in the applicable cases, exactly with what people do who form the shifted tangent bundle of a differential graded manifold, thereby giving yet another way of making the relation of tangent categories to the ordinary notion of tangent spaces manifest.
B) indicate how one should correctly think of the -category of curvature -functors and how this relates to the fact that they are related to universal -bundles.
C) remark on the relation of tangent categories with the Yoneda embedding
August 18, 2007
On BV Quantization, Part II
Posted by Urs Schreiber
A review of some basics of classical BV formalism, with an eye towards my claim (motivated in part I) that this is secretly about quantization on an -category (or rather on a Lie -groupoid – or, rather, on the corresponding Lie -algebroid).
August 17, 2007
On BV Quantization. Part I.
Posted by Urs Schreiber
Of course, one of the questions I heard after my talk on the right structure 3-group of Chern-Simons theory here at the ESI workshop Poisson-Sigma Models, Lie Algebroids, Deformations and Higher Analogues in Vienna was
Why would we need to realize Chern-Simons theory as a 3-functor?
The true answer is
Because that’s how the dao works. It simply is the way it will turn out to be and we shouldn’t fight it.
But I am not supposed to argue like that. I am supposed to give answers that point out that it is useful to do this. That it helps to solve problems which otherwise cannot be solved.
Okay, sure. If it follows the dao, it is bound to help us solve problems. So here we go: I point out that we have a pretty good idea how to better understand 2-dimensional CFT starting from 3-functorial CFT, which crucially depends on that 3-functoriality. And in order to understand that still better, it would be helpful to understand how the Chern-Simons 3-functor comes to us from first principles.
I could go on about that point, but that’s not what my topic shall be right at the moment. Rather, there is yet another good reply:
Since (so I claim), -dimensional QFT is best understood as an -functor, it follows that if you already have a better than average understanding of -dimensional QFT, then chances are that you are already secretly using -functors yourself. Without noticing so. And in that case, it would immensely boost our overall understanding if we’d made that explicit. Since it will add to your computational prowess the glory of conceptual understanding.
That’s actually why I am here at this workshop in the first place: a priori I am not all that interested in -algebras, dG-manifolds, Courant algebroids and all the other highly involved algebraic structures that people here like to fill their blackboards with. These structures all look rather involved and somewhat messy. I wouldn’t really want to care about them – unless I knew that all this is really the shadow of very sensible structures: -algebras are just Lie -algebras, dG-manifolds are just a funny way to talk about higher Lie algebroids, Courant algebroids are just certain Lie 2-algebroids coming from something like sections of an Atiyah-2-bundle. And so on.
Only with this interpretation in mind does all this here make sense to me, and I’d dare to claim that, while the differential algebraic realization is very helpful for efficient computations, only with these interpretations do we know what the right things to do with these gadgets are.
Okay. Now there is one big topic here which is probably the most general, most powerful and most fascinating of all of these. And it is the one where I don’t yet quite know the “true” interpretation of, in the above sense:
Batalin-Vilkovisky formalism, also known as BV-Quantization.
Or, at least I didn’t. Until I heard Jae-Suk Park and Dmitry Roytenberg give talks on this.
Now I think I am beginning to see on the horizon that, possibly, BV-Quantization in fact is secretly precisely about what Isham called Quantization on a category, or rather on an -category, and what I keep referring to as the program of studying the the charged quantum -particle.
The key is once again to keep in mind the equivalence between (quasi-free) differential graded algebras, -algebras and Lie -algebras for arbitrary , and to keep in mind that Lie -algebras are the differential version of Lie -group – or in fact that Lie -algebroids are the differential version of Lie -groupoids – and to use that in order to translate all that differential algebra (and BV quantization is graded differential algebra taken to the extreme) back into something of manifest intrinsic meaning.
Of course I am far from fully understanding BV-quantization, let alone that translation which i would like to perform. But that won’t stop me from talking about it.
Here I start, instead of beginning to comprehensively describe BV-formalism itself (but Jim Stasheff just writes in to tell me to read
Jim Stasheff
The (secret?) homological algebra of the Batalin-Vilkovisky approach
hep-th/9712157
), by pointing out that there are indications that people in BV-theory have already, without admitting it, realized Chern-Simons theory as a 3-functor. And it seems they have even found, implicitly, just that Lie 3-algebra which I am claiming is the right Lie 3-algebra of Chern-Simons theory.
Higher Gauge Theory and the String Group
Posted by John Baez
Tomorrow — unless stopped by global warming demonstrations at Heathrow — I’ll go to meet Urs Schreiber in Vienna. I’ve been wanting to talk to him for quite a while now. He produces math faster than I can keep up, and I hope it’ll be easier to catch up in person. Blogs are great, but conversation is still better for many things.
We’ll be attending a Workshop on Poisson Geometry and Sigma Models organized by Anton Alekseev, Henrique Bursztyn and Thomas Strobl at the Erwin Schrödinger Institute. I’m giving this talk:
- John Baez, Higher gauge theory and the string group.
Abstract: Higher gauge theory is a generalization of gauge theory that describes the parallel transport not just of particles, but also strings or higher-dimensional branes. To handle strings, we categorify familiar notions from gauge theory and consider ‘principal 2-bundles’ with a given ‘structure 2-group’. These are a slight generalization of nonabelian gerbes. We focus on examples related to the 2-groups associated to any compact simply-connected simple Lie group . We describe how these 2-groups are built using the level- central extension of the loop group of , and how they are related to the ‘string group’. Finally, we discuss 2-bundles with as structure 2-group, and pose the problem of computing characteristic classes for such 2-bundles in terms of connections.
Future Gazing
Posted by David Corfield
A year and a day into this blog perhaps we should open up a thread to allow some speculation as to what the next year might bring. Restricting myself here to philosophy, but feel free to choose any discipline, what prospects are there for greater attention being paid to n-categories from that quarter? I reckon the most likely notice will come from philosophers of physics, at least at the level. Hans Halvorson (Princeton) I know is interested, and is arranging a conference at which John is speaking (Oct 3-4).
But what about the central heartlands of philosophy? Can we hope for a first step towards something akin to the revolution wrought by Russell and then the Vienna Circle? A sympathetic philosopher, Mike Beaney, asks for the “potential philosophical pay-off”? As I reply there, what’s not clear to me is what and how to pay. Perhaps now I’ll be returning to a philosophy department I can get a better idea.
Something I’d like to know more about is the reception of Russell’s ideas. How quickly were his achievements recognised? How long was it before the average Anglo-Saxon philosopher felt it incumbent upon him or herself to learn some predicate logic? I ought to spend some time looking through back copies of Mind.
August 16, 2007
On Roberts and Ruzzi’s Connections over Posets
Posted by Urs Schreiber
I should say more about how
J. Roberts, G. Ruzzi
A cohomological description of connections and curvature over posets
math/0604173
which last time I mentioned here, relates to what I keep talking about.
Given any group , the authors of the above paper consider (I am slightly rephrasing this)
a one-object groupoid which they call
a one-object strict 2-groupoid which they call
a one-object strict 3-groupoid which they call
They then discuss how a connection on a -bundle is, locally, essentially a functor taking values in , while its curvature is a 2-functor taking values in , while the Bianchi identity is a statement in .
(Actually, J. Roberts and G. Ruzzi don’t write about 1- and 2-functors, but about cocycles on posets with values in 1- and 2-categories. But, as roughly indicated on their p. 22, below equation (43), there is a functorial picture underlying this.)
In The inner automorphism 3-group of a strict 2-group, David Roberts and I discuss how for any strict 2-group (so it might in particular be just an ordinary (1-)group), there is a 3-group (a Gray-group, in general, meaning that everything is strict except possibly the 2-functoriality of the product 2-functor) which fits into an exact sequence (We consider this as an exact sequence on the level of the underlying strict 2-groupoids, but in fact all morphisms are morphisms of 3-groups.)
Among other things, the capital letters here are supposed to remind us that does remember the center of the 2-group.
I am claiming that a good way to think of this is to realize as the “tangent 2-groupoid” to the identity 2-functor on .
In fact, we have an embedding [proposition 3, p. 11] whose image we call , and which is the really interesting part.
The fact that “remembers the center” is important, since it makes “contractible”. This, in turn, makes it play the role of the universal -bundles. But it also makes it, from my perspective, the “right” codomain for 3-curvature, as described, for instance, in section 2.4 of Arrow-theoretic differential theory.)
Of course one might ask what one gets when we divide out the center from . I believe what one gets is exactly what John Roberts and G. Ruzzi find.
I believe that
the groupoid is what I call , namely the one-object groupoid whose set of morphisms is the group (that much is obvious)
the 2-groupoid is the quotient of (the suspension of) by the categorical center
the 3-groupoid is the quotient of (the suspension of) by the center
August 15, 2007
Math Teach Wiki
Posted by David Corfield
Back from Tuscany, I find two e-mails requests awaiting me.
First, and I’m now very late on this story, Alexandre Borovik asked me to draw attention to the plight of a Mathematical Summer School held in Turkey.
Second, Tim Porter wished readers to know about “the very last PhD thesis from Bangor (for the foreseeable future)”, where
Richard Lewis looks at the problem of the interpretation of the formal maps to a crossed module introduced by Porter and Turaev and using a simplicial analogue of etale spaces gets a representation in terms of locally constant stacks.
Bangor stopped taking mathematics students in 2005.
Perhaps we need a Wiki to treat the unjustified stopping of mathematical instruction to accompany the one on journals.
August 14, 2007
The Canonical 1-Particle, Part II
Posted by Urs Schreiber
As I possibly mentioned before, I am interested in understanding how to canonically (in the category-theoretic sense!) quantize a parallel transport -functor to obtain the quantum propagation -functor of the extended QFT describing the -particle charged under the -functor.
I want to understand this in particular in order to systematically understand how Chern-Simons theory arises as a 3-functorial theory, because we have some idea about how that will allow to understand 2-dimensional CFT from first principles.
The first step in this program is to find the right structure -group which makes a parallel transport -functor taking values in it reproduce the desired classical action functional. (This step for Chern-Simons I discussed here.)
The next step is to undertand in detail how a classical parallel transport -functor is quantized to a propagation -functor. That is, how to proceed along the third edge of the cube.
This involves finding the right arrow-theory for “taking sections” and/or (following Freed) “doing the path integral”.
I am testing my ideas on this on the simple case of the 1-particle, i.e. of ordinary quantum mechanics. In The canonical 1-Particle it was pointed out that the path integral, including its measure, might be conceivable entirely in terms of a colimit involving the classical parallel transport functor.
The main observation there was this: suppose, for definiteness, we are looking at the particle propagating on the 2-dimensional plane, which we model by the category of the graph . Just to get our hands on some concrete and tractable example.
Then, decreeing that the space of “one step histories”, i.e. of paths the particle may trace out in one time interval, is that of paths of at most one edge length, one finds that the path integral over paths of unit temporal length is the colimit of some functor over the category One of the important assumptions which I made in part one was that our functor here, which is supposed to be the pullback of some “wave function” to the space of these histories, is actually that: a functor instead of a function, taking values in something like John Baez’s phased sets (a set equipped with a map to a group “of phases”, usually ).
My main point here is to not only to justify this assumption, but to actually derive it using arrow-theoretic differential theory. But before getting into that, let me finish saying what the main point of part one had been:
namely, if we assume our functor on the above category to take values in something like sets, and to be “free” in that it sends all morphisms to monomorphisms, then its colimit over the above category is goverened by the Leinster-measure This just says, in words, that the colimit will take the disjoint union of all the phased sets but then get rid of the overcounting which is induced by the fact that the set in the center sits inside all the other sets but must only be counted once.
It is clear how the analogous situation looks like in arbitrary dimensions: in dimensions we find 1s everywhere except in the center, where we get a weight .
So with denoting the value of our functor at , the colimit produces a phased set isomorphic to
If we take our lattice spacing scale to be given by Planck’s constant as where is the mass of the 1-particle and the time unit, then this is precisely the Taylor expansion to first order of the exponential of the lattice Laplace operator (Here denotes the first-order expansion of the exponential.)
If we hence take to be the category of “one step histories” and pull-push our wave functions through the correspondence this induces the action of the usual (euclidean) quantum mechanical propagator to first order in our time unit.
To propagate the 1-particle over more than one time step, we continue pull-pushing through the correspondences The map induced by such steps is In the joint limit this approaches the ordinary continuous (euclidean) propagator.
I had said all this before, if maybe not as coherently. But it deserves to be said again.
Here I will now discuss why indeed wave functions are functors with values in phased sets, using just fundamental (if you wish) arrow-theoretic differential theory.
Lazaroiu on G-Flows on Categories
Posted by Urs Schreiber
I just heard a talk (in the context of the ESI program that I am attending) by C. Lazaroiu which mentioned aspects of his article
C. I. Lazaroiu
Graded D-branes and skew categories
hep-th/0612041
in which he studies categories of branes for the topological string.
These categories are typically graded by an abelian group . This grading can be understood as originating in the supersymmetry which was “twisted” to go from the superconformal to the topological string (I once tried to summarize some aspects of this process here).
Now, in the entry Supercategories I argued that such categories should have the property that there is a -flow on them. Using the Arrow-theoretic differential theory which I was talking about, we can talk about -flows on categories as a generalization of the ordinary concept of the flow along an ordinary vector field – which is indeed reproduced in terms of smooth -flows.
In particular, if we have a supercategory we want to see a -flow on it. Or, if we have -extended supersymmetry, really an -flow. (I pointed out that these seem to have appeared in the study of representations of the -extended 1-dimensional supertranslation algebra here.)
Similarly, if the supersymmetry is “twisted” such that the -grading somehow turns into that of a larger abelian group (like most notably, as described in Aspinwall’s review), we woud want to see the relevant category to come equipped with an -flow.
Given these considerations, I was pleased to see that this is exactly what Lazariou does arrive at in his work.
Proposition. What Lazaroiu calls a graded category with shifts [Lazaroiu06, p.7] is a category with a -flow [Supercategories, def. 1].
In other words, Lazaroiu’s “graded categories with shifts” are categories eqipped with a (faithful) action of the group by inner automorphisms : (which are usefully thought of as a generalization of the concept of a Lie derivative).
August 13, 2007
Question about Tensor Categories
Posted by Urs Schreiber
Hendryk Pfeiffer asked me to forward the following question to the Café.
Dear -category people,
I have a question about tensor categories on which I would appreciate comments and references. As probably several people are interested in this, I decided to ask this question here.
The short version of my question is:
Are there examples of -linear additive spherical categories that are non-degenerate, but not semisimple?
In more detail:
August 12, 2007
Math Journal Wiki
Posted by John Baez
It’s great to see what while I was goofing off in Norway, Blake Stacey has been doing some real work. After some discussion on this blog, he set up a prototype of what he called the ‘MathSciJournalWiki’.
Thanks to the conversation below, it now has a catchier name: Eureka.
What is it, exactly? It’s an attempt to catalyze the move to open-access math publication by keeping an eye on math journals and their publishers. It should spotlight new developments like cloaking by academic publishers, and the mass resignations of editorial boards from evil Elsevier–Springer journals like Topology and K-Theory.
However, it’s is still in an embryonic form. That’s great! It means you, reading this, have some power to shape its future! Here are some comments and questions I have…
This Week’s Finds in Mathematical Physics (Week 255)
Posted by John Baez
In week255, hear what happened at the 2007 Abel Symposium in Oslo. Read explanations of Jacob Lurie and Ulrike Tillman’s talks on cobordism n-categories, Dennis Sullivan and Ralph Cohen’s talks on string topology, Stephan Stolz’s talk on cohomology and quantum field theory, and Fabien Morel’s talk on A1-homotopy theory.
But first, take a tour of the Paris Observatory:

August 10, 2007
String and Chern-Simons Lie 3-Algebras
Posted by Urs Schreiber
String and Chern-Simons Lie 3-Algebras
a slide show
August 8, 2007
Arrow-Theoretic Differential Theory, Part II
Posted by Urs Schreiber
Recently I was contacted by somebody who had thought truly long, hard and deep about higher morphisms of Lie -algebras: homotopies and higher homotopies of maps of -algebras.
He expressed concerns that the formulas for these higher homotopies which we give in the provisional Structure of Lie -Algebras, while coming close, actually in general have to receive correction terms.
Correction terms, that is, which apparently nobody has managed to get a complete handle on. (Should you, dear reader, be the exception to this statement, please let me know.)
While I have to admit that at one point I did falsely believe that the formulas we give are correct in general (I am grateful to Danny Stevenson for a remark on this point), I was relieved to be able to point out that there are explicit warnings on p. 3 and on p. 12 pointing out that the formulas in fact do apply – but only for the case that the target Lie -algebra has a rather special property.
In fact – and this is where it seems things become interesting – this special property is shared in particular by Lie -algebras which are inner derivation Lie -algebras of Lie -algebras As readers of this blog know, I have for a long time now assembled what I consider increasing evidence that inner derivation Lie -algebras and inner automorphism Lie -groups are quite important concepts – for various reasons, actually. See for instance the recent article with David Roberts on The inner automorphism 3-group of a strict 2-group for more details.
So now I am wondering: is it a coincidence that all attempts to explicitly define higher homotopies of Lie -algebras so far have failed, while the only case that is understandable is that where the target Lie -algebra is one of inner derivations? Or is this maybe trying to tell us something?
I am now going to argue that this is possibly supposed to be telling us something.
More precisely, I shall indicate that using what I called Arrow-Theoretic Differential Theory one finds what is actually a simple, obvious and natural explanation.
At least as far as I can see currently.
August 7, 2007
Adinkras
Posted by Urs Schreiber
Late one night, a while ago, Blake Stacey noticed that there are “category diagrams” which, in the twilight, look like Adinkras

– at least to some people:
Doran, C. F.; Faux, M. G.; Gates, S. J.; Hubsch, T.; Iga, K. M.; Landweber, G. D.
On Graph-Theoretic Identifications of Adinkras, Supersymmetry Representations and Superfields
math-ph/0512016
The simplest such Adinkra appearing in the study of -extended supersymmetry looks like This corresponds to . A slightly more interesting one is obtained for :
I was struck, since to me these look like the categorical super-point and the categorical 2-super point.

Let me try to explain…
August 5, 2007
Questions about Modules
Posted by John Baez
Here’s another question that’s been bugging me a long time. I had so much luck with the last one that I’m feeling slightly optimistic. We’ll see.
Question: Which categories are categories of modules for some ring? Which functors between these are given by tensoring with some bimodule? And which natural transformations between those are given by tensoring with some bimodule morphism?
I can make this a bit more precise…
August 4, 2007
Gauge Tranformations of n-Bundles and (n-1)-Gerbes
Posted by Urs Schreiber
Over on sci.math.research Christoph Wockel today asks:
can anyone help me with finding the appropriate notion of a gauge transformation on a gerbe (abelian or not)? I would be interested in particular in lifting gerbes. I tried to find it several times but did not succeed. My guess would be to define it as a vertical 2-bundle automorphismsm of the corresponding 2-bundle, but I did not find this mentioned anywhere… Accordingly, gauge transformations would build up a 2-group rather than a group.
I’ll give a quick reply here, indicating the basic idea. Upon request I can spell out more details.
August 3, 2007
QFT of Charged n-Particle: Towards 2-Functorial CFT
Posted by Urs Schreiber
[ Update: We now have a more developed write-up:
Jens Fjelstad and U. S.
Rational CFT is parallel transport
(pdf)
]
With Jens Fjelstad I am working on understanding 2-dimensional conformal field theory as an extended quantum field theory, that is: as a propagation 2-functor of a charged 2-particle.
Various aspects of this I mention every now and then. An overview of some of the existing work had accompanied my Fields Institute Talk: On 2-dimensional QFT: from Arrows to Disks.
Here is a description of some main aspects of the project.
August 2, 2007
QFT of Charged n-Particle: Extended Worldvolumes
Posted by Urs Schreiber
For a while I had lost my License to Quantize. But now I got it back. So I resume talking about the Quantum theory of the Charged -Particle (I, II, III, IV, V, VI, VII, VIII, IX).
Recall, this is supposed to be the study of the situation where we
- have an -particle (: a particle, : a string, : a membrane) modeled by an -category (its parameter space) which is essentially an -category
for the pointlike particle
for the line-like string
for the disk-shaped membrane
- propagating (i.e. being mapped into) a target space (for instance the path -groupoid of a spacetime for a sigma-model, or an -group for a gauge theory )
- and coupled there to a background field modeled by an -bundle with connection, thought of as the corresponding parallel transport -functor
and we want to consider the quantization of this setup, which is supposed to be itself an -functor, namely the Segal-like extended worldvolume QFT which assigns to points an -vector space of states, to 1-dimensional cobordisms a morphisms of -vector spaces, and so on.
While in the original Segal picture an -dimensional QFT is thought of as a mere 1-functor which sends -dimensional cobordisms going between their -dimensional boundaries to linear maps between vector spaces associated to these boundaries, we are here modelling our -dimensional boundaries – namely what we call “parameter space” – by -categories, thus assigning data not just to the top two dimensions, but all the way down to points.
This is idea is usually called an extended functorial QFT. Some people refer to it as “localized” QFT. I am not sure exactly what the best term would actually be. But in need of a term, I shall here refer to this kind of -categorical refinement of functorial QFT as local refinement.
But – and this is what I am going to talk about here – there is actually also a different, alternative way to extend a Segal-like QFT functor to an -functor discussed in the literature:
instead of allowing the original -dimensional objects to decompose into lower-dimensional pieces and thus regarding them as -morphisms instead of objects, one sometime leaves the objects the way they are and instead throws in more morphisms between the existing cobordisms. For instance one may want not to divide out homeomorphisms of -dimensional cobordisms in an -dimensional topological QFT, but instead throw in one 2-morphism for each such homeomorphism. This concept I shall here refer to as global refinement.
(See the literature mentioned here.)
One may wonder what to make of this parallel existence of two different notions of extended QFT. Here I want to point out that
Local refinement is adjoint to global refinement.
In a sense that can be made precise in the context of the -particle. It crucially involves Gray tensor products of higher categories.
One nice way to think about this turns out to be this:
The worldvolume (worldline for the particle, worldsheet for the string, etc.) of the -particle arises from the Gray tensor product of the particle with the timeline.
I thought that’s a cute statement which deserves to be put in bold here. Below I give the precise statement that this is referring to.
August 1, 2007
Astronomical London
Posted by John Baez
In my recent post on Astronomical Paris, I gave you a tour of L’Observatoire de Paris. Now I’m in Greenwich, a stone’s throw away from the Royal Observatory:

Like the observatory in Paris, this one has a long and glamorous history, from the first Astronomer Royal — John Flamsteed, who quarreled with Newton and Halley, and publicly burnt a book they wrote based on his observations without crediting him — to the current one, Martin Rees.
I wrote a bit about the Royal Observatory back in week175. Later, some kind soul offered to give me a tour when I visited next. Now I’d like that very much… I could take photos and give you a comparison tour.
But, I can’t remember who made the offer! It’s very embarrassing. ![]()
If anyone feels like showing me the secrets of this observatory, could they drop me a line?
 Posts with this logo use
Posts with this logo use 














